Boolean Addition, Multiplication, Commutative Law, Associative Law, Distributive Law, Demorganâ€™s Theorems

<< DC Supply Voltage, TTL Logic Levels, Noise Margin, Power Dissipation

Simplification of Boolean Expression, Standard POS form, Minterms and Maxterms >>

CS302 - Digital Logic & Design

Lesson No. 08

BOOLEAN ALGEBRA AND LOGIC SIMPLIFICATION

Any digital circuit no matter how complex can be described by Boolean Expressions.

Boolean algebra is the mathematics of Digital Systems. Knowledge of Boolean algebra is

indispensable to the study and analysis of logic gates. AND, OR, NOT, NAND and NOR gates

perform simple Boolean operations and Boolean expressions represent the Boolean

operations performed by the logic gates.

· AND gate

F = A.B

· OR gate

F=A+B

F=A

· NOT gate

F = A.B

· NAND gate

F=A+B

NOR gate

Boolean expressions which represent Boolean functions help in two ways. The function

and operation of a Logic Circuit can be determined by Boolean expressions without

implementing the Logic Circuit. Secondly, Logic circuits can be very large and complex. Such

large circuits having many gates can be simplified and implemented using fewer gates.

Determining a simpler Logic circuit having fewer gates which is identical to the original logic

circuit in terms of the function it performs can be easily done by evaluating and simplifying

Boolean expressions.

Boolean Algebra expressions are written in terms of variables and literals using laws,

rules and theorems of Boolean Algebra. Simplification of Boolean expressions is also based

on the Boolean laws, rules and theiorems.

Boolean Algebra Definitions

1. Variable

A variable is a symbol usually an uppercase letter used to represent a logical quantity.

A variable can have a 0 or 1 value.

2. Complement

A complement is the inverse of a variable and is indicated by a bar over the variable.

Complement of variable X is X . If X = 0 then X = 1 and if X = 1 then X = 0.

3. Literal

A Literal is a variable or the complement of a variable.

Boolean Addition

Boolean Addition operation is performed by an OR gate. In Boolean algebra the

expression defining Boolean Addition is a sum term which is the sum of literals.

A + B, A + B, A + B + C

A sum term is 1 when any one literal is a 1

A sum term is 0 when all literals are a 0.

CS302 - Digital Logic & Design

Boolean Multiplication

Boolean Multiplication operation is performed by an AND gate. In Boolean algebra the

expression defining Boolean Multiplication is a product term which is the product of literals.

A.B , A.B , A.B.C

A product term is 1 when all literal terms are a 1

A product term is 0 when any one literal is a 0.

Laws of Boolean Algebra

The basic laws of Boolean Algebra are the same as ordinary algebra and hold true for

any number of variables.

1. Commutative Law for addition and multiplication

2. Associative Law for addition and multiplication

3. Distributive Law

1. Commutative Law for Addition and Multiplication

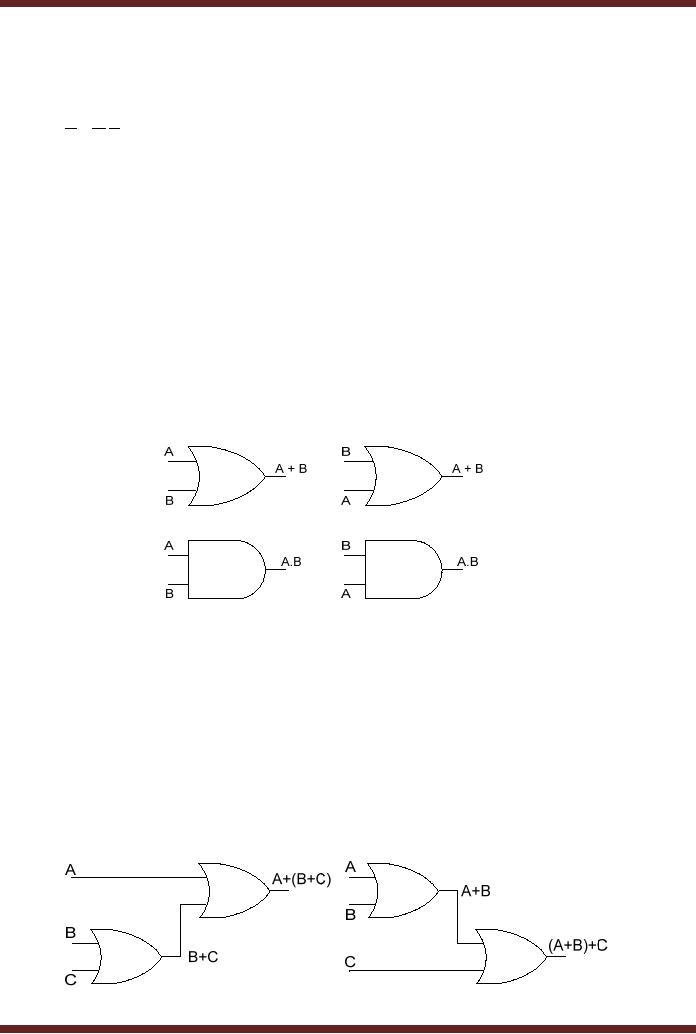
· Commutative Law for Addition

A+B=B+A

· Commutative Law for Multiplication

A.B = B.A

Figure 8.1

Implementation of Commutative Laws

In terms of implementation, the Boolean Addition and Multiplication of two or more

literals is the same no matter how they are ordered at the input of an OR and AND Gates

respectively. Commutative law for Addition and Multiplication holds true for n number of

literals.

2. Associative Law for Addition and Multiplication

· Associative Law for Addition

A + (B + C) = (A + B) + C

· Associative Law for MultiplicationA.(B.C) = (A.B).C

CS302 - Digital Logic & Design

A.(B.C)

A.B

(A.B).C

B.C

Figure 8.2

Implementation of Associative Laws

In terms of implementation, the Associative ordering of literals for Boolean Addition and

Multiplication is the same at the input of an OR and AND gates. Commutative law for Addition

and Multiplication holds true for n number of literals. The addition of literals B and C followed

by the addition of literal A with the result of B+C is the same as adding literals A and B

followed by the addition of literal C.

The multiplication of literals B and C followed by the multiplication of the result of B.C

with literal A is the same as multiplying literals A and B followed by the multiplication of literal

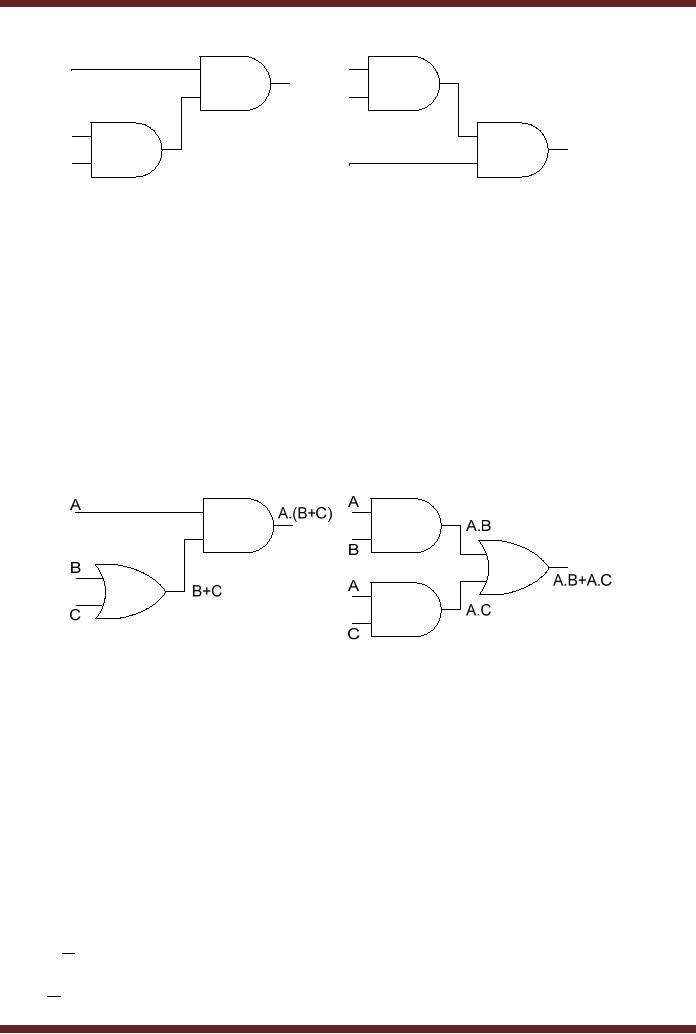
3. Distributive Law

· Distributive Law

A.(B + C) = A.B + A.C

Figure 8.3

Implementation of Distributive Law

Distributive law holds true for any number of literals. Adding literals B and C followed

by multiplying the result with literal A is the same as multiplying literal A with literal B and

adding the result to the product of literals A and C.

Rules of Boolean Algebra

Rules of Boolean Algebra can be proved by replacing the literals with Boolean values 0

and 1.

A+0=A

A+1=1

A.0 = 0

A.1 = A

A+A=A

A+ A=1

A.A = A

A. A = 0

CS302 - Digital Logic & Design

9. A = A

10. A + A.B = A

= A.(1 + B)

where (1+B) according to Rule 2 is equal to 1

11. A + A.B = A + B

= A(B+1) + A.B

according to Rule 2 (B+1) = 1

= AB +A + A.B

= B(A+ A ) +A

according to Rule 6 A + A = 1

=B+A

12. (A+B).(A+C) = A+B.C

= AA+AC+AB+BC applying the Distributive Law

= A(1+C+B) +BC

according to Rule 2 (1+B+C) = 1

= A+BC

Demorgan's Theorems

Demorgan's First Theorem states: The complement of a product of variables is equal

to the sum of the complements of the variables.

A.B = A + B

Demorgan;s Second Theorem states: The complement of sum of variables is equal to

the product of the complements of the variables.

A + B = A.B

Demorgan's two theorems prove the equivalency of the NAND and negative-OR gates

and the NOR and negative-AND gates respectively. Figure 8.4

A +B

A.B

A +B

A.B

Figure 8.4

Implementation of Demorgan's Theorems

Demorgan's Theorems can be applied to expressions having any number of variables

· X.Y.Z = X + Y + Z

X + Y + Z = X.Y.Z

CS302 - Digital Logic & Design

Demorgan's Theorem can be applied to a combination of other variables

(A + B.C).(A.C + B) = (A + B.C) + (A.C + B)

= A.(B.C) + (A.C).B

= A.(B + C) + (A + C).B

= A.B + A.C + A.B + B.C

= A.B + A.C + B.C

Boolean Analysis of Logic Circuits

Boolean algebra provides a concise way to represent the operation of a logic circuit.

The complete function of the logic circuit can be determined by evaluating the Boolean

expression using different input combinations.

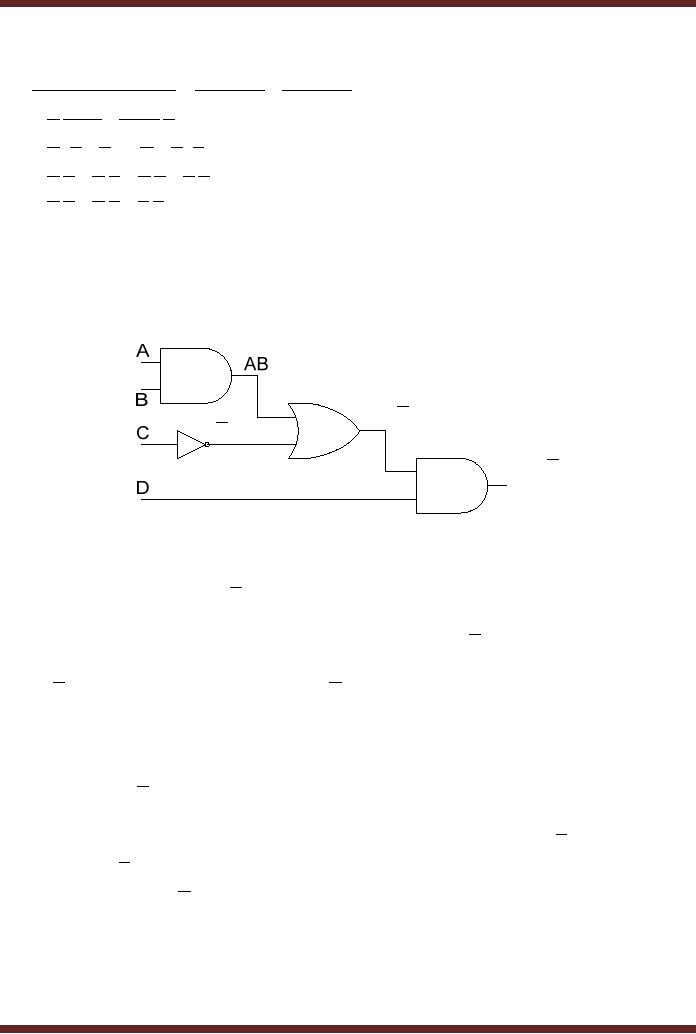
AB + C

(AB + C)D

Figure 8.5

Boolean expression representing a Logic Circuit

The expression (AB + C)D can be derived from the circuit by starting from the left

hand, input side of the Logic Circuit. The AND gate provides the output AB. The OR gate adds

the product term AB and the complement C to result in (AB + C) term. The AND gate on the

right hand side of the circuit performs a multiplication operation between the term

(AB + C) and the literal D resulting in (AB + C)D .

There are four variables, therefore the function table or truth table for the logic circuit

has 16 possible input combinations. The expression can be evaluated by trying out the 16

combinations. Alternately, the input combinations A, B, C and D that set the output of the

expression (AB + C)D to 1 can be easily determined.

From the expression, the output is a 1 if both variable D = 1 and term (AB + C) =1.

The term (AB + C) =1 only if AB=1 or C=0.

Thus expression (AB + C)D =1

if D=1 AND (C=0 OR AB=1)

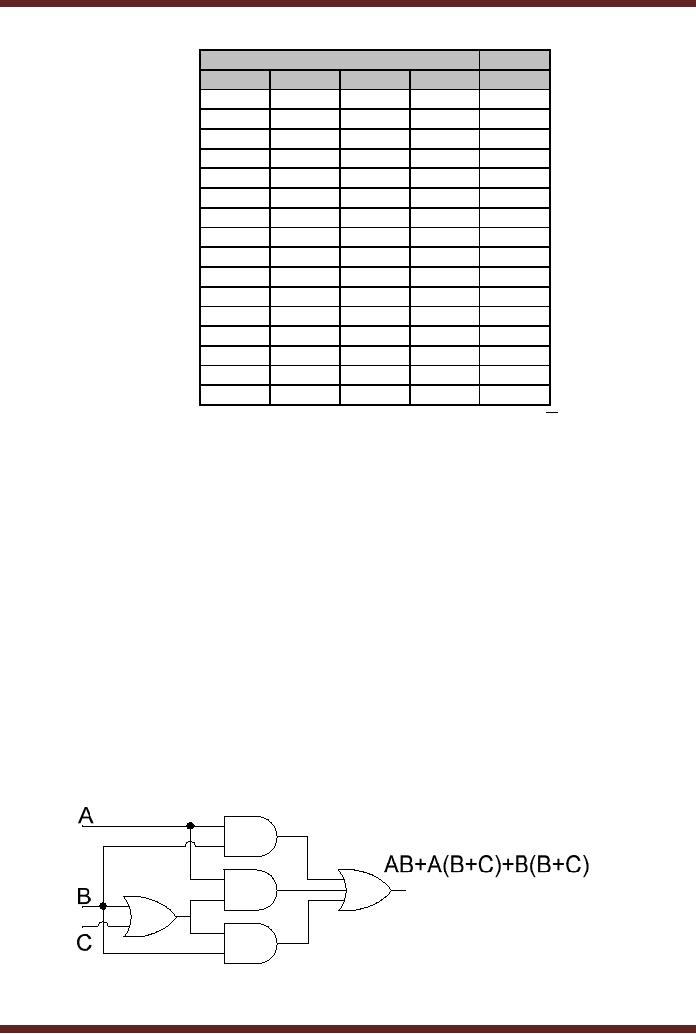
CS302 - Digital Logic & Design

Inputs

Output

Function table for expression (AB + C)D

Table 8.1

In the function table the input conditions for variables A, B, C and D that satisfy the

condition D=1 AND C=0 are 0001, 0101, 1001. The condition D=1 AND AB=1 are satisfied by

input combination 1111. The condition D=1 AND (C=0 OR AB=1) is satisfied by the input

combination 1101.

Simplification using Boolean Algebra

Many times a Boolean expression has to be simplified using laws, rules and theorems

of Boolean Algebra. The simplified expression results in fewer variables and a simpler circuit.

Consider the Boolean expression AB + A(B+C) + B(B+C) and the Logic Circuit represented by

the expression. Figure 8.6. The simplification of the expression results in an expression B +

AC represented by a simpler circuit having fewer gates. Figure 8.7

= AB + A(B+C) + B(B+C)

= AB + AB + AC + BB +BC

using Distributive Law

= AB + AC + B + BC

BB = B using rule 7

= AB + AC + B

(B+BC) = B using rule 10

= B + AC

(B+AB) = B using rule 10

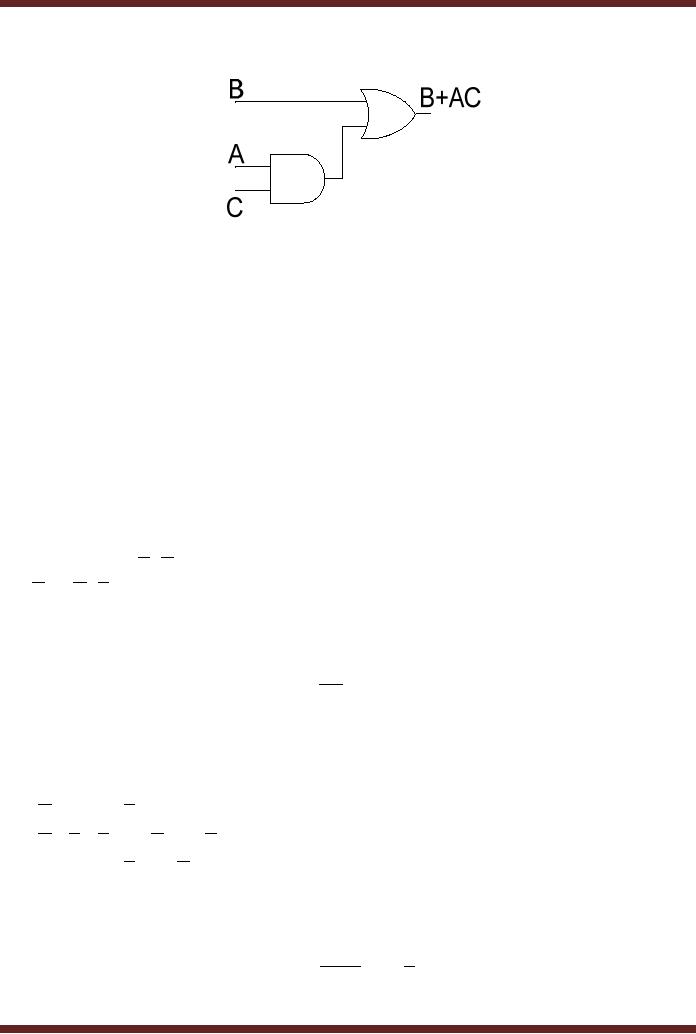
Figure 8.6

Logic Circuit represented by Boolean expression AB + A(B+C) + B(B+C)

CS302 - Digital Logic & Design

Figure 8.7

Simplified Logic Circuit represented by Boolean expression B+AC

Standard Form of Boolean Expressions

All Boolean expressions can be converted into and represented in one of the two

standard forms

Sum-of-Products form

Product-of-Sums form

1. Sum of Product form

When two or more product terms are summed by Boolean addition, the result is a

Sum-of-Product or SOP expression.

AB + ABC

ABC + CDE + BCD

AB + ABC + AC

The Domain of an SOP expression is the set of variables contained in the expression,

both complemented and un-complemented. A SOP expression can have a single variable term

such as A. A SOP expression can not have a term of more than one variable having an over

bar extending over the entire term, such as AB + C .

2. Product of Sums form

When two or more sum terms are multiplied by Boolean multiplication, the result is a

Product-of-Sum or POS expression.

(A + B)(A + B + C)

(A + B + C)(C + D + E)(B + C + D)

(A + B)(A + B + C)(A + C)

The Domain of a POS expression is the set of variables contained in the expression,

both complemented and un-complemented. A POS expression can have a single variable term

such as A. A POS expression can not have a term of more than one variable having an over

bar extending over the entire term such as (A + B)(A + B + C) .

CS302 - Digital Logic & Design

Implementation of an SOP and POS expression

A SOP expression can be implemented by an AND-OR combination of gates. The

product terms are implemented by an AND gate and the SOP expression is implemented by

OR gate connected to the outputs of the AND gates. Figure 8.8

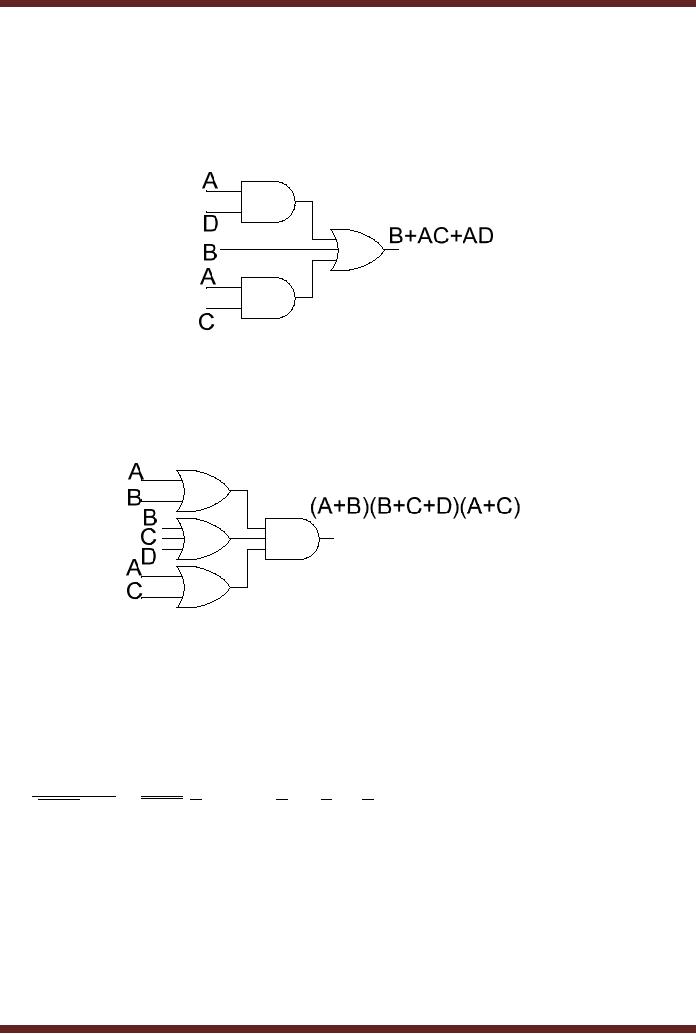
Figure 8.8

SOP Implementation of Boolean expression B+AC+AD

A POS expression can be implemented by an OR-AND combination of gates. The sum

terms are implemented by OR gates and the POS expression is implemented by AND gate

connected to the outputs of the OR gates.

Figure 8.9

POS Implementation of Boolean expression (A+B)(B+C+D)(A+C)

Conversion of a general expression to SOP form

Any logical expression can be converted into SOP form by applying techniques of

Boolean Algebra

AB + B(CD + EF) = AB + BCD + BEF

(A + B)(B + C + D) = AB + AC + AD + B + BC + BD = AC + AD + B

(A + B) + C = (A + B)C = (A + B)C = AC + BC

Table of Contents: