Elevator Control System: Elevator State Diagram, State Table, Input and Output Signals, Input Latches

<< APPLICATIONS OF SHIFT REGISTERS: Serial-to-Parallel Converter

Traffic Signal Control System: Switching of Traffic Lights, Inputs and Outputs, State Machine >>

CS302 - Digital Logic & Design

Lesson No. 36

EXAMPLE4: 3-BIT UP/DOWN COUNTER

The 3-bit Up/Down Counter was earlier implemented using J-K flip-flops. A D flip-flop

based 3-bit Up/Down Counter is implemented by mapping the present state and next state

information in D Input table. Table 36.1. The Karnaugh maps and the simplified Boolean

expressions derived from the D Input table, table 36.2 are used to implement the 3-bit

Up/Down counter circuit. Figure 36.1

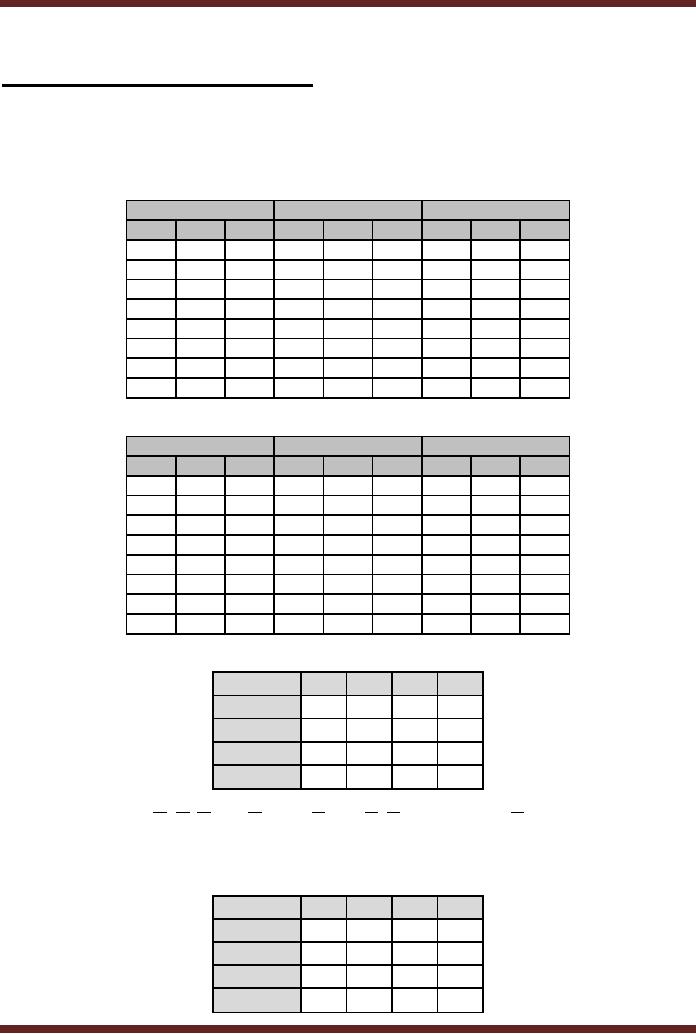
Present State

Next State X=0

D flip-flop inputs

Table 36.1a

D flip-flop input table for X=0

Present State

Next State X=1

D flip-flop inputs

Table 36.1b

D flip-flop input table for X=1

Q2Q1/Q0X

D 2 = Q 2 Q1 Q 0 X + Q 2Q1Q 0 X + Q 2 Q 0 X + Q 2Q1 X + Q 2 Q1Q 0

Table 36.2a

Boolean expression for D2 inputs

Q2Q1/Q0X

367

CS302 - Digital Logic & Design

D1 = Q1 Q 0 X + Q1 Q 0 X + Q1Q 0 X + Q1Q 0 X

D1 = (Q1 Q 0 + Q1Q 0 )X + (Q1 Q 0 + Q1Q 0 )X

D1 = Q 0 ⊕Q1 ⊕ X

Table 36.2b

Boolean expression for D1 inputs

Q2Q1/Q0X

D0 = Q0

Table 36.2c

Boolean expression for D0 inputs

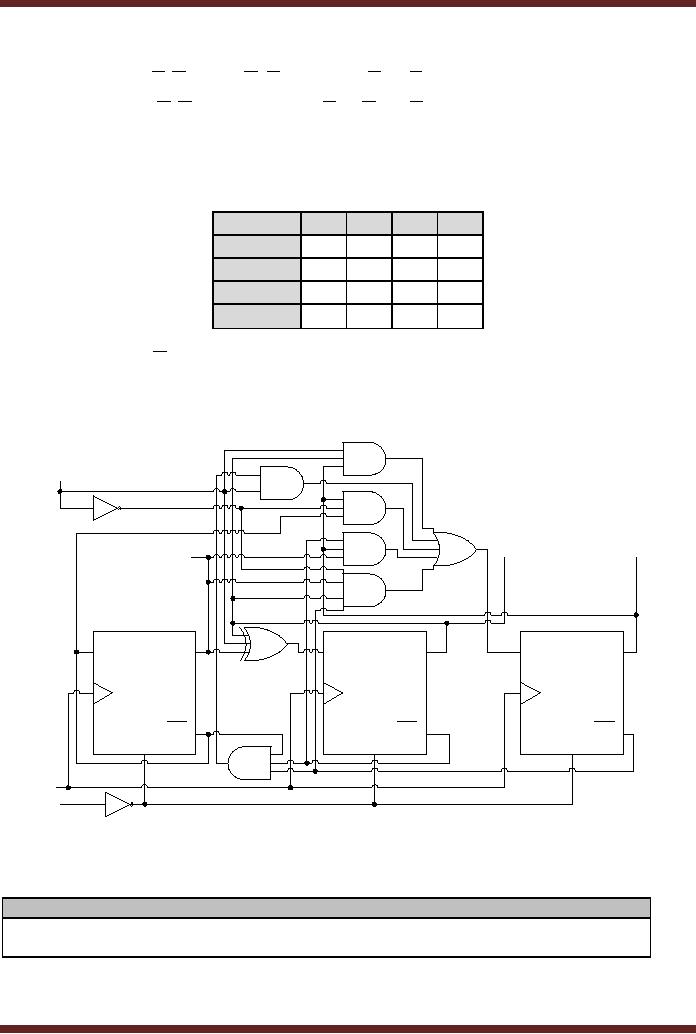
X=0 (up)

X=1 (down)

SET

flip-flop 2

flip-flop 1

flip-flop 3

CLR

CLK

Clear

Figure 36.1 3-bit Up/Down Counter

The main definitions and declarations of the ABEL input file for the Up/Down Counter is

shown. Table 36.3.

Pin Definition

CLOCK, CLEAR, X

pin 1, 2, 3;

Q0, Q1, Q2

pin 21, 22, 23 ISTYPE `reg,buffer';

Table 36.3a

Input/Output Pin Definition of 3-bit Up/Down Counter

368

CS302 - Digital Logic & Design

The Clock, Clear and X input variables applied at pins 1, 2 and 3 are used to provide the clock

signal, the asynchronous clear pulse and the external input to control the direction of the count

sequence. The Q0, Q1 and Q2 outputs are available from the D flip-flops of the three OLMCs

at pins 21, 22 and 23. Since these outputs are sequential outputs available from the flip-flops

therefore they are defined of type register `reg.buffer'. The three outputs are the State

variables that define the different states. Table 36.3a

Equations

Q0 := !Q0;

Q1 := Q0 $ Q1 $ X;

Q2 := !Q2 & !Q1 & !Q0 & X # !Q2 & Q1 & Q0 & !X # Q2 & !Q0 & !X

# Q2 & Q1 & X # Q2 & !Q1 & Q0;

[Q0, Q1, Q2].CLK = clock;

[Q0, Q1, Q2].AR = !clear;

Table 36.3b

Equation Definition of 3-bit Up/Down Counter

The ABEL Equations definition defines the Next state outputs for the three state variables. The

ABEL assignment equations represent the Boolean expressions derived for the three D flip-

flop inputs, table 36.2. Thus the next state output for variable Q0 depends upon the D0 input

which is defined by the Boolean expression as Q 0 . Similarly, for the next state output for

variable Q1 depends upon the D1 input which is defined by the Boolean expression

as Q 0 ⊕Q1 ⊕ X . The ABEL next state expression for state variable Q3 is similarly based on the

Boolean expression for Q3.

The ABEL statements [Q0, Q1, Q2].CLK = clock and [Q0, Q1, Q2].AR = !clear declare

the change from the present state to the next state on a clock transition and the Asynchronous

reset (AR) of all the three D flip-flops in the OLMC by the Clear input signal. Table 36.3b

The Test Vector definition defines the test vectors for all the three counter inputs and

the three counter outputs. Since the Asynchronous input overrides the Synchronous X input,

therefore in the first test vector when the Clear Asynchronous input is 0 the output is cleared to

000 irrespective of the X Synchronous input. When the Clear input is set to 1, the counter

functions normally, the X input set to 0 sets the counter to increment and the X input set to 1

sets the counter to decrement. Table 36.3c

Test Vector

([Clock, Clear, X] -> [Q2, Q1, Q0])

[ .c. , 0 ,.x.] -> [0 , 0 , 0 ];

[ .c. , 1 , 0 ] -> [0 , 0 , 1 ];

[ .c. , 1 , 0 ] -> [0 , 1 , 0 ];

[ .c. , 1 , 0 ] -> [0 , 1 , 1 ];

[ .c. , 1 , 0 ] -> [1 , 0 , 0 ];

[ .c. , 1 , 0 ] -> [1 , 0 , 1 ];

[ .c. , 1 , 0 ] -> [1 , 1 , 0 ];

[ .c. , 1 , 0 ] -> [1 , 1 , 1 ];

[ .c. , 1 , 0 ] -> [0 , 0 , 0 ];

[ .c. , 1 , 1 ] -> [1 , 1 , 1 ];

[ .c. , 1 , 1 ] -> [1 , 1 , 0 ];

[ .c. , 1 , 1 ] -> [1 , 0 , 1 ];

[ .c. , 1 , 1 ] -> [1 , 0 , 0 ];

369

CS302 - Digital Logic & Design

[

.c.

, 1 ] -> [0

1 ];

[

.c.

, 1 ] -> [0

0 ];

[

.c.

, 1 ] -> [0

1 ];

[

.c.

, 1 ] -> [0

0 ];

Table 36.3c Test Vector Definition of 3-bit Up/Down Counter

Using a Truth-Table to specify Sequential Circuit

The ABEL Input file can use a truth table instead of the equation to specify the Boolean

expressions. The Equation definition of the ABEL input file reduces to the two statements

defining the change in the output state based on the clock transition and the Asynchronous

reset of the D flip-flops through the Asynchronous Clear signal. Table 36.4a.

Equations

[Q0, Q1, Q2].CLK = clock;

[Q0, Q1, Q2].AR = !clear;

Table 36.4a

Equation Definition for Truth Table based Sequential Circuit definition

The 3-bit Up/Down Sequential circuit's complete operation can be described by a truth table

which has external inputs Clear, X and Present State variables Q0, Q1 and Q2. The output of

the counter circuit are the Next State variables Q0, Q1, Q2. The first statement of the truth

table definition indicates that when Clear is set to 0, the counter output is reset to 000

irrespective of the X input and the present state inputs. The next 16 statements define the 8

input combinations and the corresponding counter outputs when the counter is counting up

and the 8 input combinations and its corresponding outputs when the counter is counting

down. Table 36.4b.

Truth Table

Truth_Table

([Clear, X, Q2, Q1, Q0] :> [Q2, Q1, Q0])

[ 0 ,.x., .x. , .x. , .x. ] :> [ 0 , 0 , 0 ];

[ 1 , 0 , 0 , 0 , 0 ] :> [ 0 , 0 , 1 ];

[ 1 , 0 , 0 , 0 , 1 ] :> [ 0 , 1 , 0 ];

[ 1 , 0 , 0 , 1 , 0 ] :> [ 0 , 1 , 1 ];

[ 1 , 0 , 0 , 1 , 1 ] :> [ 1 , 0 , 0 ];

[ 1 , 0 , 1 , 0 , 0 ] :> [ 1 , 0 , 1 ];

[ 1 , 0 , 1 , 0 , 1 ] :> [ 1 , 1 , 0 ];

[ 1 , 0 , 1 , 1 , 0 ] :> [ 1 , 1 , 1 ];

[ 1 , 0 , 1 , 1 , 1 ] :> [ 0 , 0 , 0 ];

[ 1 , 1 , 0 , 0 , 0 ] :> [ 1 , 1 , 1 ];

[ 1 , 1 , 1 , 1 , 1 ] :> [ 1 , 1 , 0 ];

[ 1 , 1 , 1 , 1 , 0 ] :> [ 1 , 0 , 1 ];

[ 1 , 1 , 1 , 0 , 1 ] :> [ 1 , 0 , 0 ];

[ 1 , 1 , 1 , 0 , 0 ] :> [ 0 , 1 , 1 ];

[ 1 , 1 , 0 , 1 , 1 ] :> [ 0 , 1 , 0 ];

[ 1 , 1 , 0 , 1 , 0 ] :> [ 0 , 0 , 1 ];

[ 1 , 1 , 0 , 0 , 1 ] :> [ 0 , 0 , 0 ];

Table 36.4b

Truth Table definition for the 3-bit Up/Down Counter

370

CS302 - Digital Logic & Design

Using a State Diagram to specify Sequential Circuit

The ABEL Input file can also use a State diagram to specify the states of the Sequential

circuit. Before specifying the State diagram, the states have to be defined. After defining the

states the state diagram is defined by indicating how the present state changes to the next

state. ABEL, IF-THEN-ELSE statements or GOTO statements are used to specify the how the

present state changes to the next state.

State Definition

QSTATE

= [Q2, Q1, Q0];

= [ 0 , 0 , 0 ];

= [ 0 , 0 , 1 ];

= [ 0 , 1 , 0 ];

= [ 0 , 1 , 1 ];

= [ 1 , 0 , 0 ];

= [ 1 , 0 , 1 ];

= [ 1 , 1 , 0 ];

= [ 1 , 1 , 1 ];

Table 36.5a

State definition of the 3-bit Up/Down Counter

The QSTATE variable defines the eight states of the counter circuit. Each state defined by the

three state variables is identified by state names A, B to H. Table 36.5a.

State Diagram

State A:

if X then H else B;

State B:

if X then A else C;

State C:

if X then B else D;

State D:

if X then C else E;

State E:

if X then D else F;

State F:

if X then E else G;

State G:

if X then F else H;

State H:

if X then G else A;

Table 36.5b

Defining the next states using IF-THEN-ELSE

The ABEL, IF-THEN-ELSE statements are used to define the input conditions for which the

present state changes to the next state. For example, in the State Diagram, the State A

changes to state H if the input variable X=1 otherwise the next state is state B. Similar, if then

else statements are used to define the next states for each of the present states. If the present

state switches to the next state without checking any conditions then an ABEL, GOTO

statement is used. For example, for a 3-bit Up counter GOTO statements are used to specify

the next state without checking any condition. Table 36.5c

State Diagram

State A:

GOTO B;

State B:

GOTO C;

State C:

GOTO D;

State D:

GOTO E;

State E:

GOTO F;

371

CS302 - Digital Logic & Design

State F:

GOTO G;

State G:

GOTO H;

State H:

GOTO A;

Table 36.5c

Defining the next states using GOTO

The ABEL Input file which uses the State diagram instead of equations or the truth table

replaces the Equation part by State Definitions and State Diagram. The Equation definition

only defines the change in state on a clock transition and the Asynchronous input as shown in

table 36.4a

Design Example: Elevator Control System

An elevator is installed in a building that moves from one floor to the other. A person

going to the second floor from the first floor presses a request button on the first floor. When

the elevator arrives at the first floor, the doors open and the person walks in to the elevator.

The person presses the request button for the second floor. When the elevator reaches the

second floor, it stops and the doors open. The doors are opened for a specified time. A person

inside the elevator can keep the doors open for a longer duration of time if an `Open' button is

pressed. Inside the elevator and outside near each entrance to the elevator there is a 7-

segment display which displays the floor on which the elevator currently is. The direction, Up

or Down in which the elevator is moving is also displayed.

Input and Output Signals

Different inputs and outputs are required to control the operation of the elevator. The

operation of the elevator is based on a sequential state machine. A State diagram describes

all the operations of the elevator. The inputs that are received from the person in the form of

requests are

Request buttons to call the elevator, REQ1 and REQ2

Floor request buttons inside the elevator, FLOOR1 and FLOOR2

Open door button, OPEN

The duration for which the elevator doors are opened, and remain open, and the time it takes

for the elevator to move form one floor to the next is determined by a clock signal. When the

elevator arrives at a floor a floor sensor generates an ARRIVE signal. Thus the State machine

uses to additional input signals.

Clock signal, CLOCK

Arrive signal, ARRIVE

The elevator generates three output signals to indicate the doors OPEN/CLOSED, direction of

movement of elevator UP/DOWN and the motion of the elevator WAITING/MOVING.

Door Open/Close, DOOR=0 and DOOR=1

Direction Up/Down, DIR=0 and DIR=1

Motion Waiting /Moving, MOTION=0 and MOTION=1

In addition to the three output signals the elevator generates signals to display the floor

number and the direction in which the elevator is moving.

372

CS302 - Digital Logic & Design

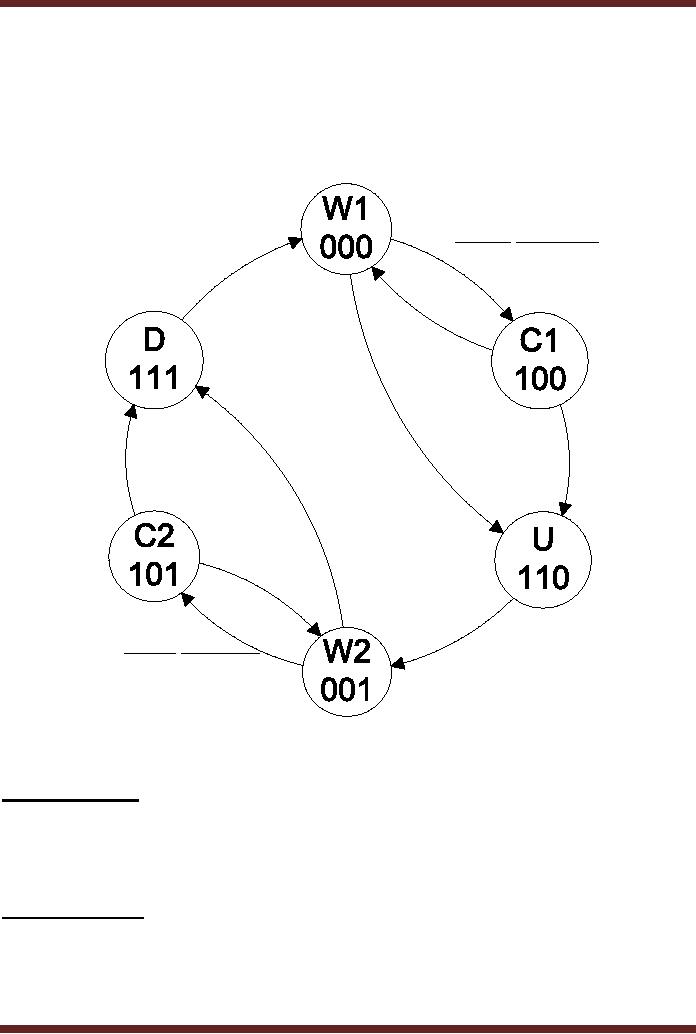
Elevator State Diagram

The State diagram of the elevator operation has six states. Figure 36.2. Three state variables

are required to define the six states. The state outputs directly determine the status of the

door, the direction of the motion and control the motion.

REQ2.FLOOR2

ARRIVE

REQ1 + OPEN

REQ2 + FLOOR2

REQ1 + FLOOR1

REQ2 + FLOOR2

REQ1 + FLOOR1

REQ2 + OPEN

ARRIVE

REQ1.FLOOR1

Figure 36.2

State Diagram of Elevator

Wait 1 State (W1): The initial state of the elevator is the Wait 1 State (W1), where the elevator

is waiting on the first floor with the door open. The W1 state 000 identifies the state outputs.

· Door=0

(open)

· Motion=0 (waiting)

· Dir=0

(up)

Close 1 State (C1): If for a fixed interval of time, a request from second floor is not received or

the button for floor 2 is not pressed, the system goes to state Close1 (C1). In this state the

elevator remains on the first floor with its doors closed. The C1 state 100 identifies the output

signals.

· Door=1

(close)

· Motion=0 (waiting)

373

CS302 - Digital Logic & Design

Dir=0

(up)

If at any time a person inside the elevator presses the Open door switch, OPEN or a

person waiting on first floor requests for the elevator REQ1, the system switches back to Wait

1 State, W1.

Up State (U): The system switches to Up state (U) when either a request is received from the

second floor REQ2 or a person presses the Floor2 switch F2. The U State represents the state

when the elevator is moving up. The outputs of this state are 110.

· Door=1

(close)

· Motion=1 (moving)

· Dir=0

(up)

Similarly when the system is in state W1, the system switches to Up state (U), when either a

request is received from the second floor REQ2 or a person presses the Floor2 switch F2.

Wait 2 State (W2): The system switches from Up state (U), to Wait 2 state (W2) when the

Arrive signal is received from the floor sensor. In the W2 state the elevator is waiting on floor 2

with its door open. The output of the W2 state are 001

· Door=0

(open)

· Motion=0 (waiting)

· Dir=1

(down)

Close 2 State (C2): The elevator waits with its door open on the second floor for a specified

period of time. If a request from first floor is not received or the button for floor 1 is not

pressed, the system switches to Close2 state (C2). In this state the elevator remains on the

second floor with its doors close. The output signals of C2 state are 101

· Door=1

(close)

· Motion=0 (waiting)

· Dir=1

(down)

If at any time a person inside the elevator presses the Open door switch, OPEN or a

person waiting on second floor requests for the elevator REQ2, the system switches back to

Wait 2 State, W2.

Down State (D): The system switches to Down state (D), when either a request is received

from the first floor REQ1 or a person presses the Floor1 switch F1. The D, Down represents

the state when the elevator is moving down. The outputs of this state are 111.

· Door=1

(close)

· Motion=1 (moving)

· Dir=1

(down)

Similarly when the system is in state W2, the system switches to state D, Down when either a

request is received from the first floor REQ1 or a person presses the Floor1 switch F1.The

system switches to the Wait 1 state (W1) when the Arrive signal is received from the floor

sensor on the first floor.

374

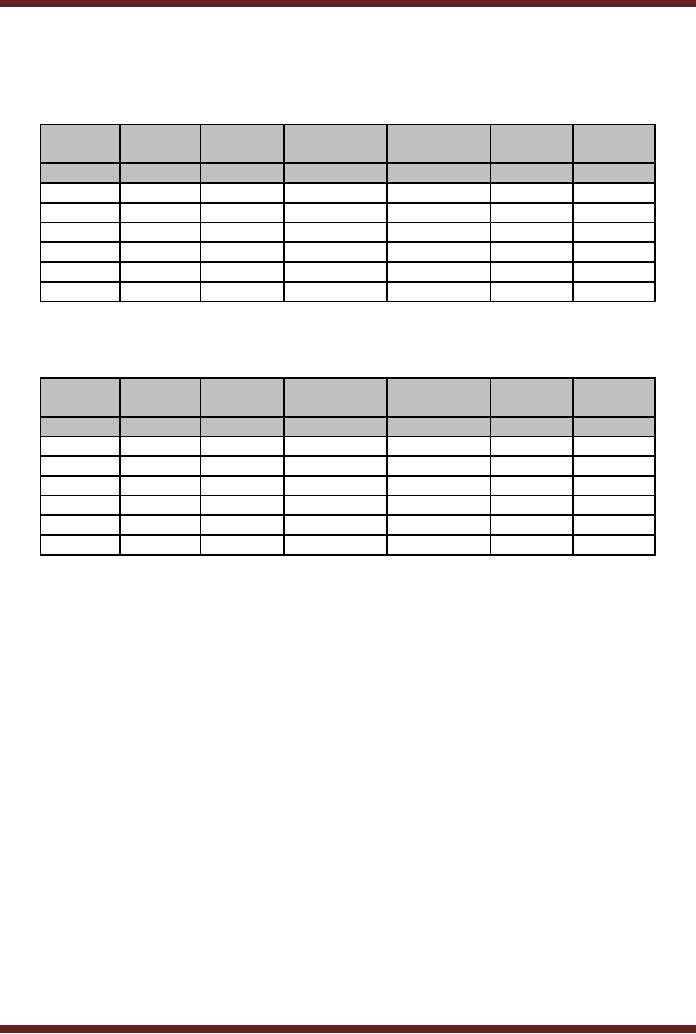
CS302 - Digital Logic & Design

State Table

Present

Next State

State

REQ1=0

REQ1=1

FLOOR1=0

FLOOR1=1

OPEN=0

OPEN=1

W1(000) x

C1(100) C1

UP(110) x

W2(001) C2

C2(101) C2

DO(111) x

Table 36.6a

State table for Elevator Control for REQ1, FLOOR1 and OPEN inputs

Present

Next State

State

REQ2=0

REQ2=1

FLOOR2=0

FLOOR2=1

OPEN=0

OPEN=1

W1(000) C1

C1(100) C1

UP(110) x

W2(001) x

C2(101) C2

DO(111) x

Table 36.6b

State table for Elevator Control for REQ2, FLOOR2 and OPEN inputs

The Next State tables for the Elevator Control are obtained directly from the State Diagram.

The State table show the present and the next states for each of the five external inputs that

are activated by people using the elevator. The ARRIVE external input is activated by a

separate sensor circuit and is not activated by press of a button. The next states when the

ARRIVE signal is active are not shown in the State Tables.

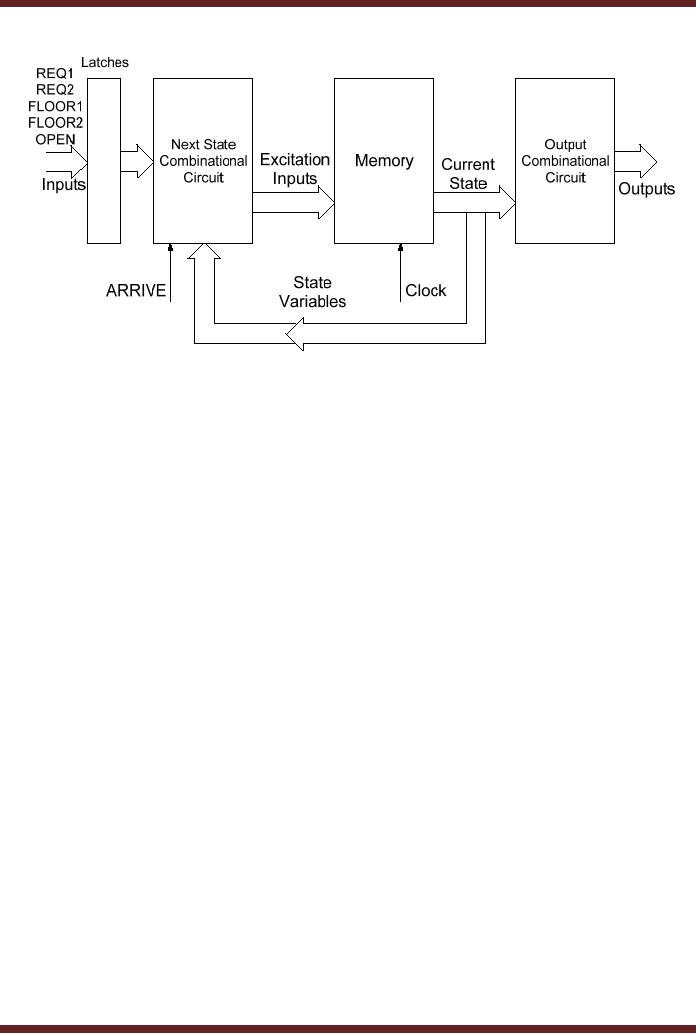
Input Latches

The request buttons to call the elevator, REQ1 and REQ2, the Floor request buttons

inside the elevator, F1 and F2 and the Open door button, OPEN can be pressed at any time. A

sequential circuit switches from one state to the next on the basis of its present state and

external input. Supposedly, a person presses and releases the REQ1 button to request for the

elevator in a time period between two consecutive clock transitions. At the clock transition, the

status of the REQ1 switch is inactive therefore the REQ1 is not entertained. It is therefore

important that all input buttons, REQ1, REQ2, F1, F2 and OPEN are connected through

latches which are not controlled by a clock signal. Figure 36.3. A total of 5 latches are required

to store the inputs received from the buttons REQ1, REQ2, F1, F2 and OPEN.

375

CS302 - Digital Logic & Design

Figure 36.3 Block diagram of the Elevator State Machine

376

Table of Contents: