AN OVERVIEW & NUMBER SYSTEMS

Binary to Decimal to Binary conversion, Binary Arithmetic, 1â€™s & 2â€™s complement >>

CS302 - Digital Logic & Design

Lesson No. 01

AN OVERVIEW & NUMBER SYSTEMS

Analogue versus Digital

Most of the quantities in nature that can be measured are continuous. Examples include

· Intensity of light during the day: The intensity of light gradually increases as the sun

rises in the morning; it remains constant throughout the day and then gradually decreases

as the sun sets until it becomes completely dark. The change in the light throughout the

day is gradual and continuous. Even with a sudden change in weather when the sun is

obscured by a cloud the fall in the light intensity although very sharp however is still

continuous and is not abrupt.

· Rise and fall in temperature during a 24-hour period: The temperature also rises and

falls with the passage of time during the day and in the night. The change in temperature is

never abrupt but gradual and continuous.

· Velocity of a car travelling from A to B: The velocity of a car travelling from one city to

another varies in a continuous manner. Even if it abruptly accelerates or stops suddenly,

the change in velocity seemingly very sudden and abrupt is never abrupt in reality. This

can be confirmed by measuring the velocity in short time intervals of few milliseconds.

The measurable values generally change over a continuous range having a minimum and

maximum value. The temperature values in a summer month change between 23 0C to 45 0C.

A car can travel at any velocity between 0 to 120 mph.

Digital representing of quantities

Digital quantities unlike Analogue quantities are not continuous but represent quantities

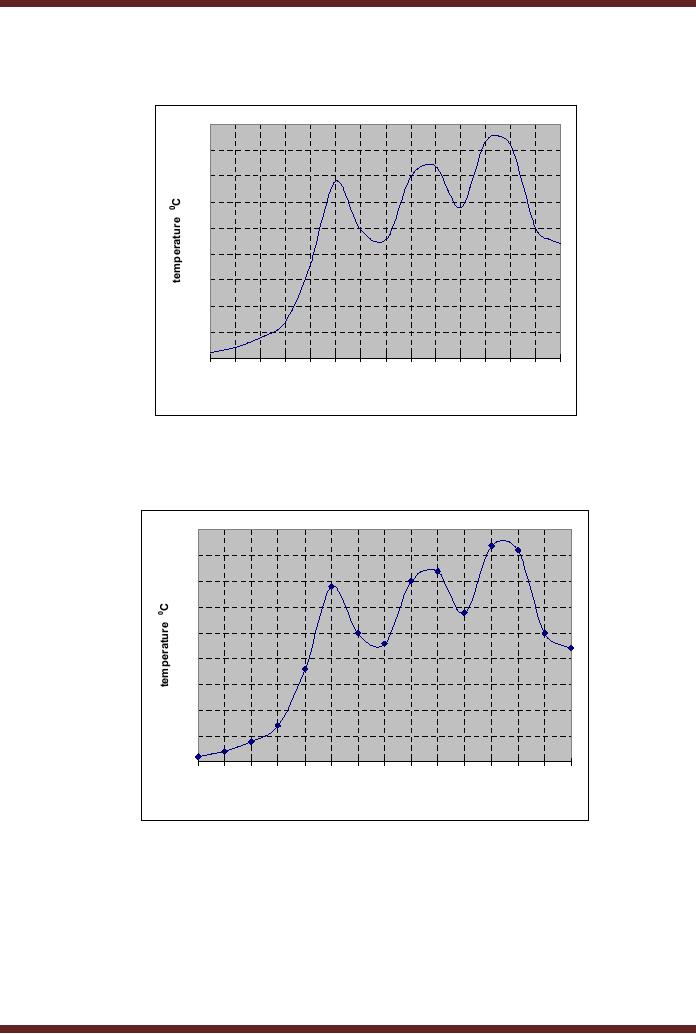
measured at discrete intervals. Consider the continuous signal as shown in the figure 1.1.

To represent this signal digitally the signal is sampled at fixed and equal intervals. The

continuous signal is sampled at 15 fixed and equal intervals. Figure 1.2. The set of values (1,

2, 4, 7, 18, 34, 25, 23, 35, 37, 29, 42, 41, 25 and 22) measured at the sampling points

represent the continuous signal. The 15 samples do not exactly represent the original signal

but only approximate the original continuous signal. This can be confirmed by plotting the 15

sample points. Figure 1.3. The reconstructed signal from the 15 samples has sharp corners

and edges in contrast to the original signal that has smooth curves.

If the number of samples that are collected is reduced by half, the reconstructed signal will

be very different from the original. The reconstructed signal using 7 samples have missing

peak and dip at 34 0C and 23 0C respectively. Figure 1.4. The reason for the difference

between the original and the reconstructed signal is due to under-sampling. A more accurate

representation of the continuous signal is possible if the number of samples and sampling

intervals are increased. If the sampling is increased to infinity the number of values would still

be discrete but they would be very close and closely match the actual signal.

CS302 - Digital Logic & Design

time

Figure 1.1 Continuous signal showing temperature varying with time

time

Figure 1.2 Sampling the Continuous Signal at 15 equal intervals

CS302 - Digital Logic & Design

samples

Figure 1.3 Reconstructed Signal by plotting 15 sampled values

samples

Figure 1.4 Reconstructed Signal by plotting 7 sampled values

Electronic Processing of Continuous and Digital Quantities

Electronic Processing of the continuous quantities or their Digital representation requires

that the continuous signals or the discrete values be converted and represented in terms of

voltages. There are basically two types of Electronic Processing Systems.

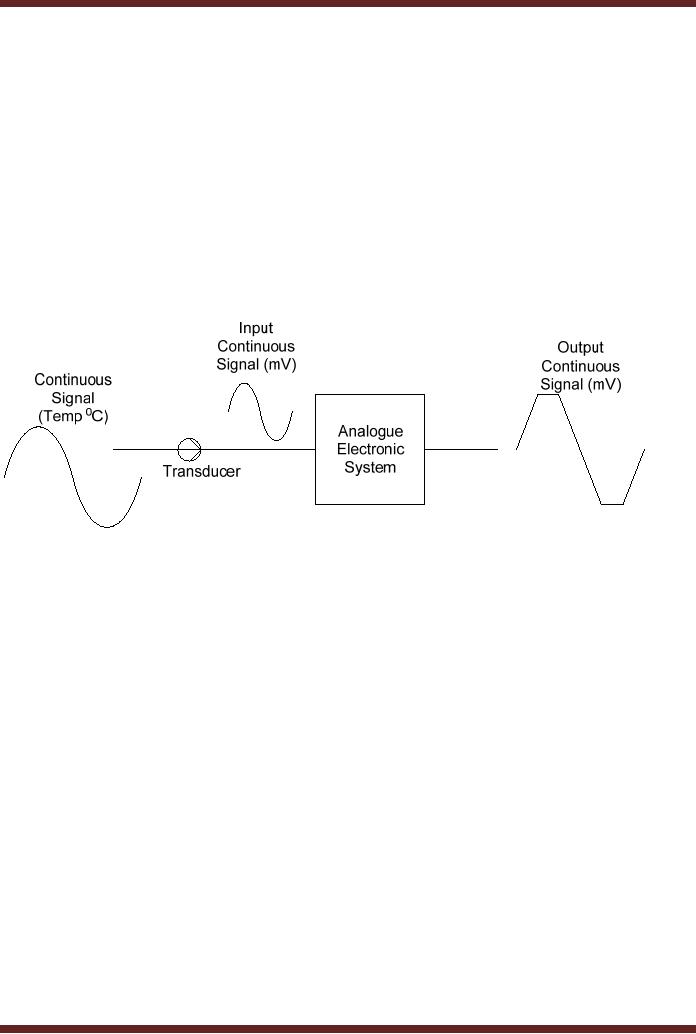
· Analogue Electronic Systems: These systems accept and process continuous signals

represented in the form continuous voltage or current signals. The continuous quantities

are converted into continuous voltage or current signals by transducers. The block diagram

describes the processing by an Analogue Electronic System. Figure 1.5.

CS302 - Digital Logic & Design

Digital Electronic Systems: These systems accept and process discrete samples

representing the actual continuous signal. Analogue to Digital Converters are used to

sample the continuous voltage signals representing the original signal.

Do the Digital Electronic Systems use voltages to represent the discrete samples of the

continuous signal? This question can be answered by considering a very simple example of a

calculator which is a Digital Electronic System. Assume that a calculator is calibrated to

represents the number 1 by 1 millivolt (mV). Thus the number 39 is represented by the

calculator in terms of voltage as 39 mV. Calculators can also represent large numbers such as

6.25 x 1018 (as in 1 Coulomb = 6.25 x 1018 electrons). The value in terms of volts is 6.25 x 1015

volts! This voltage value can not be practically represented by any electronic circuit. Thus

Digital Systems do not use discrete samples represented as voltage values.

Figure 1.5

Analogue Electronic System processing continuous quantities

Digital Systems and Digital Values

Digital systems are designed to work with two voltage values. A +5 volts represents a logic

high state or logic 1 state and 0 volts represents a logic low state or logic 0 state. The Digital

Systems which are based on two voltage values or two states can easily represent any two

values. For example,

· The numbers `0' and `1'

· The state of a switch `on' or `off'

· The colour `black' and `white'

· The temperature `hot' and `cold'

· An object `moving' or `stationary'

Representing two values or two states is not very practical, as many naturally occurring

phenomenons have values or state that are more than two. For example, numbers have

widely varying ranges, a colour palette might have 64 different shades of the colour red, the

temperature of boiling water at room temperature varies from 30 0C to 100 0C. Digital Systems

are based on the Binary Number system which allows more than two or multiple values to be

represented very conveniently.

Binary Number System

CS302 - Digital Logic & Design

The Binary Number System unlike the Decimal number system is based on two values.

Each digit or bit in Binary Number system can represent only two values, a `0' and a `1'. A

single digit of the Decimal Number system represents 10 values, 0, 1, 2 to 9. The Binary

Number System can be used to represent more than two values by combining binary digits or

bits. In a Decimal Number System a single digit can represent 10 different values (0 to 9),

representing more than 10 values requires a combination of two digits which allows up to 100

values to be represented (0 to 99). A Combination of Binary Numbers is used to represent

different quantities.

Represent Colours: A palette of four colours red, blue, green and yellow can be

represented by a combination of two digital values 00, 01, 10 and 11 respectively.

Representing Temperature: An analogue value such as 39oC can be represented in a

digital format by a combination of 0s and 1s. Thus 39 is 100111 in digital form.

Any quantity such as the intensity of light, temperature, velocity, colour etc. can be

represented through digital values. The number of digits (0s and 1s) that represents a quantity

is proportional to the range of values that are to be represented. For example, to represent a

palette of eight colours a combination of three digits is used. Representing a temperature

range of 00 C to 1000 C requires a combination of up to seven digits.

Digital Systems uses the Binary Number System to represent two or multiple values,

stores and processes the binary values in terms of 5 volts and 0 volts. Thus the number 39

represented in binary as 100111 is stored electronically in as +5 v, 0v, 0v, +5 v, +5 v and +5 v.

Advantages of working in the Digital Domain

Handling information digitally offers several advantages. Some of the merits of a digital

system are spelled out. Details of some these aspects will be discussed and studied in the

Digital Logic Design course. Other aspects will be covered in several other courses.

· Storing and processing data in the digital domain is more efficient: Computers are

very efficient in processing massive amounts of information and data. Computers process

information that is represented digitally in the form of Binary Numbers. A Digital CD stores

large number of video and audio clips. Sam number of audio and video clips if stored in

analogue form will require a number of video and audio cassettes.

· Transmission of data in the digital form is more efficient and reliable: Modern

information transmission techniques are relying more on digital transmission due to its

reliability as it is less prone to errors. Even if errors occur during the transmission methods

exist which allow for quick detection and correction of errors.

· Detecting and Correcting errors in digital data is easier: Coding Theory is an area

which deals with implementing digital codes that allow for detection and correction of multi-

bit errors. In the Digital Logic Design course a simple method to detect single bit errors

using the Parity bit will be considered.

· Data can be easily and precisely reproduced: The picture quality and the sound quality

of digital videos are far more superior to those of analogue videos. The reason being that

the digital video stored as digital numbers can be exactly reproduced where as analogue

video is stored as a continuous signal can not be reproduced with exact precision.

· Digital systems are easy to design and implement: Digital Systems are based on two-

state Binary Number System. Consequently the Digital Circuitry is based on the two-

voltage states, performing very simple operations. Complex Microprocessors are

implemented using simple digital circuits. Several simple Digital Systems will be discussed

in the Digital Logic course.

CS302 - Digital Logic & Design

Digital circuits occupy small space: Digital circuits are based on two logical states.

Electronic circuitry that implements the two states is very simple. Due to the simplicity of

the circuitry it can be easily implemented in a very small area. The PC motherboard having

an area of approximately 1 sq.ft has most of the circuitry of a powerful computer. A

memory chip small enough to be held in the palm of a hand is able to store an entire

collection of books.

Information Processing by a Digital System

A Digital system such as a computer not only handles numbers but all kinds of information.

· Numbers: A computer is able to store and process all types of numbers, integers, fractions

etc. and is able to perform different kinds of arithmetic operations on the numbers.

· Text: A computer in a news reporting room is used to write and edit news reports. A

Mathematician uses a computer to write mathematical equations explaining the dissipation

of heat by a heat sink. The computer is able to store and process text and symbols.

· Drawings, Diagrams and Pictures: A computer can store very conveniently complex

engineering drawings and diagrams. It allows real life still pictures or videos to be

processed and edited.

· Music and Sound: Musicians and Composers uses\ a computer to work on a new

compositions. Computers understand spoken commands.

A Digital System (computer) is capable of handling different types of information, which is

represented in the form of Binary Numbers. The different types of information use different

standards and binary formats. For example, computers use the Binary number system to

represent numbers. Characters used in writing text are represented through yet another

standard known as ASCII which allows alphabets, punctuation marks and numbers to be

represented through a combination of 0s and 1s.

Digital Components and their internal working

Digital system process binary information electronically through specialized circuits

designed for handling digital information. These circuits as mentioned earlier operate with two

voltage values of +5 volts and 0 volts. These specialized electronic circuits are known as Logic

Gates and are considered to be the Basic Building Blocks of any Digital circuit.

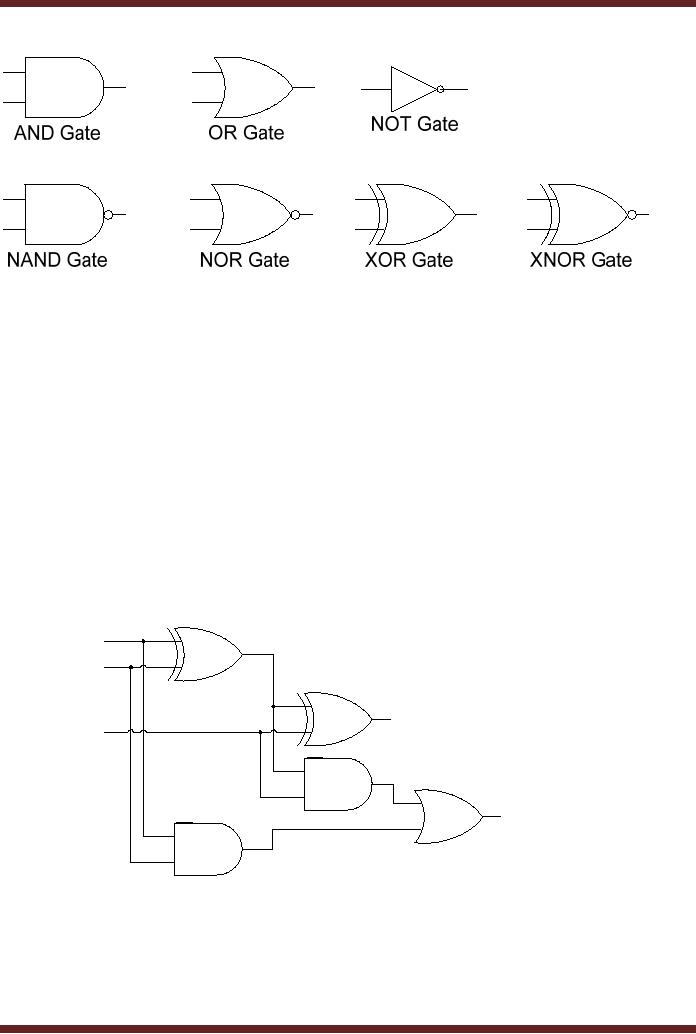
The commonly used Logic Gates are the AND gate, the OR gate and the Inverter or NOT

Gate. Other gates that are frequently used include NOR, NAND, XOR and XNOR. Each of

these gates is designed to perform a unique operation on the input information which is known

as a logical or Boolean operation.

Large and complex digital system such as a computer is built using combinations of these

basic Logic Gates. These basic building blocks are available in the form of Integrated Circuit or

ICs. These gates are implemented using standard CMOS and TTL technologies that

determine the operational characteristics of the gates such as the power dissipation,

operational voltages (3.3v or 5 v), frequency response etc.

CS302 - Digital Logic & Design

Figure 1.6 Symbolic representations of logic gates.

Combinational Logic Circuits and Functional Devices

The logic gates which form the basic building blocks of a digital system are designed to

perform simple logic operations. A single logic gate is not of much use unless it is connected

with other gates to collectively act upon the input data. Different gates are combined to build a

circuit that is capable of performing some useful operation like adding three numbers. Such

circuits are known as Combinational Logic Circuits or Combinational Circuits. An Adder

Combinational Circuit that is able to add two single bit binary numbers and give a single bit

Sum and Carry output is shown. Figure 1.7.

Implementing large digital system by connecting together logic gates is very tedious and

time consuming; the circuit implemented occupies large space, are power hungry, slow and

are difficult to troubleshoot.

∑

Cin

Cout

Figure 1.7 1-bit Full-Adder Combinational Circuit

Digital circuits to perform specific functions are available as Integrated Circuits for use.

Implementing a Digital system in terms of these dedicated functional units makes the system

more economical and reliable. Thus an adder circuit does not have to be implemented by

connecting various gates, a standard Adder IC is available that can be readily used. Other

CS302 - Digital Logic & Design

commonly used combinational functional devices are Comparators, Decoders, Encoders,

Multiplexers and Demultiplexers.

Sequential logic and implementation

Digital systems are used in vast variety of industrial applications and house hold electronic

gadgets. Many of these digital circuits generate an output that is not only dependent on the

current input but also some previously saved information which is used by the digital circuit.

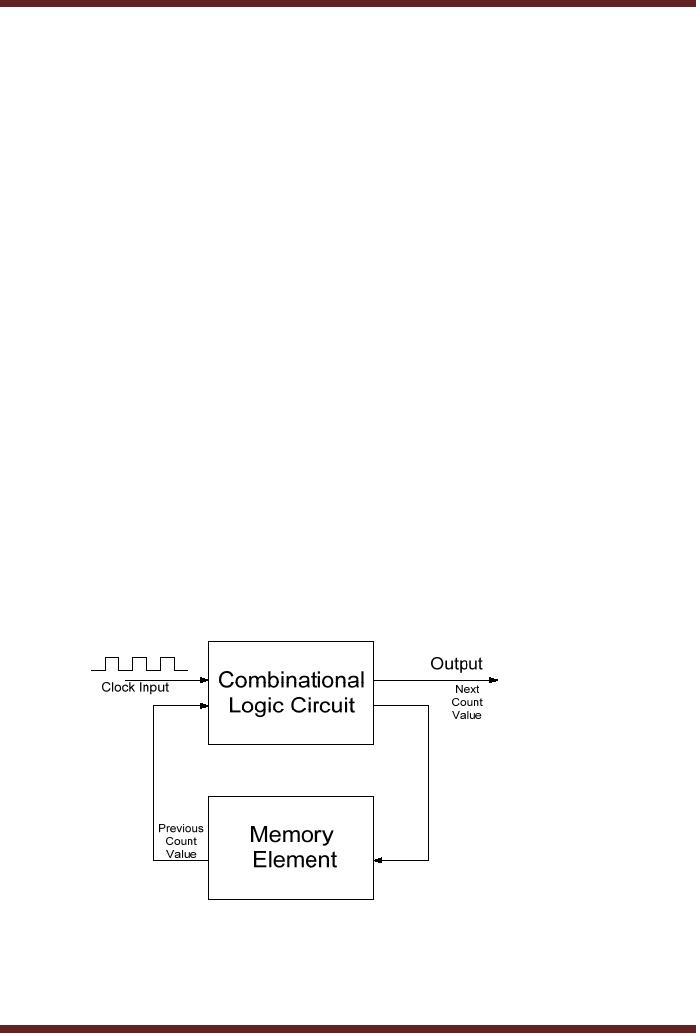
Consider the example of a digital counter which is used by many digital applications where a

count value or the time of the day has to be displayed. The digital counter which counts

downwards from 10 to 0 is initialized to the value 10. When the counter receives an external

signal in the form of a pulse the counter decrements the count value to 9. On receiving

successive pulses the counter decrements the currently stored count value by one, until the

counter has been decremented to 0. On reaching the count value zero, the counter could

switch off a washing machine, a microwave oven or switch on an air-conditioning system.

The counter stores or remembers the previous count value. The new count value is

determined by the previously stored count value and the new input which it receives in the

form of a pulse (a binary 1). The diagram of the counter circuit is shown in the figure. Figure

1.8.

Digital circuits that generate a new output on the basis of some previously stored

information and the new input are known as Sequential circuits. Sequential circuits are a

combination of Combinational circuits and a memory element which is able to store some

previous information. Sequential circuits are a very important part of digital systems. Most

digital systems have sequential logic in addition to the combinational logic. An example of

sequential circuits is counters such as the down-counter which generates a new decremented

output value based on the previous stored value and an external input. The storage element or

the memory element which is an essential part of a sequential circuit is implemented a flip-flop

using a very simple digital circuit known as a flip-flop.

Figure 1.8

A Counter Sequential Circuit

Programmable Logic Devices (PLDs)

The modern trend in implementing specialized dedicated digital systems is through

configurable hardware; hardware which can be programmed by the end user. A digital

CS302 - Digital Logic & Design

controller for a washing machine can be implemented by connecting together pieces of

combinational and sequential functional units. These implementations are reliable however

they occupy considerable space. The implementation time also increases. A general purpose

circuit that can be programmed to perform a certain task like controlling a washing machine

reduces the implementation cost and time.

Cost is incurred on implementing a digital controller for a washing machine which requires

that an inventory of all its components such as its logic circuits, functional devices and the

counter circuits be maintained. The implementation time is significantly high as all the circuit

components have to be placed on a circuit board and connected together. If there is a change

in the controller circuit the entire circuit board has to be redesigned. A PLD based washing

machine controller does not require a large inventory of components to be maintained. Most of

the functionality of the controller circuit is implemented within a single PLD integrated circuit

thereby considerably reducing the circuit size. Changes in the controller design can be readily

implemented by programming the PLD.

Programmable Logic Devices can be used to implement Combinational and Sequential

Digital Circuits.

Memory

Memory plays a very important role in Digital systems. A research article being edited by a

scientist on a computer is stored electronically in the digital memory whilst changes are being

made to the document. Once the document has be finalized and stored on some media for

subsequent printing the memory can be reused to work on some other document. Memory

also needs to store information permanently even when the electrical power is turned off.

Permanent memories usually contain essential information required for operating the digital

system. This important information is provided by the manufacturer of a digital system.

Memory is organized to allow large amounts of data storage and quick access. Memory

(ROM) which permanently stores data allows data to be read only. The Memory does not allow

writing of data. Volatile memory (RAM) does not store information permanently. If the power

supplied to the RAM circuitry is turned off, the contents of the RAM are permanently lost and

can not be recovered when power is restored. RAM allows reading and writing of data. Both

RAM and ROM are an essential part of a digital system.

Analogue to Digital and Digital to Analogue conversion and Interfacing

Real-world quantities as mention earlier are continuous in nature and have widely varying

ranges. Processing of real-world information can be efficiently and reliably done in the digital

domain. This requires real-world quantities to be read and converted into equivalent digital

values which can be processed by a digital system. In most cases the processed output needs

to be converted back into real-world quantities. Thus two conversions are required, one from

the real-world to the digital domain and then back from the digital domain to the real-world.

Modern digitally controlled industrial units extensively use Analogue to Digital (A/D) and

Digital to Analogue (D/A) converters to covert quantities represented as an analogue voltage

into an equivalent digital representation and vice versa. Consider the example of an industrial

controller that controls a chemical reaction vessel which is being heated to expedite the

chemical reaction. Figure 1.9. Temperature of the vessel is monitored to control the chemical

reaction. As the temperature of the vessel rises the heat has to be reduced by a proportional

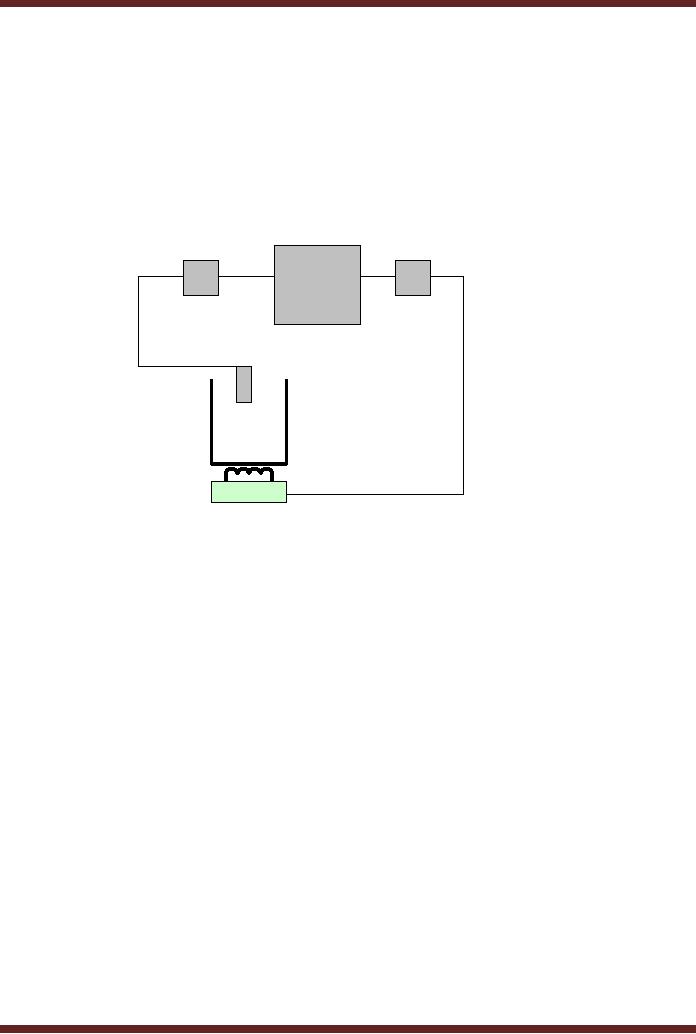
CS302 - Digital Logic & Design

level. An electronic temperature sensor (transducer) converts the temperature into an

equivalent voltage value. This voltage value is continuous and proportion to the temperature.

The voltage representing the temperature is converted into a digital representation which is fed

to a digital controller that generates a digital value corresponding to the desired amount of

heat. The digitized output representing the heat is converted back to a voltage value which is

continuous and is used to control a valve that regulates the heat. An A/D converter converts

the analogue voltage value representing the temperature into a corresponding digital value for

processing. A D/A converter converts back the digital heat value to its corresponding

continuous value for regulating the heater.

Digital

Controller

D/A

A/D

Converter

Transducer

Vessel

Heater

Figure 1.9

Digitally Controlled Industrial Heater Unit

A/D and D/A converters are an important aspect of digital systems. These devices serve

as a bridge between the real and digital world allow the two to communicate and interact

together.

Number Systems and Codes

Decimal Number System

The decimal number system has ten unique digits 0, 1, 2, 3... 9. Using these single digits,

ten different values can be represented. Values greater than ten can be represented by using

the same digits in different combinations. Thus ten is represented by the number 10, two

hundred seventy five is represented by 275 etc. Thus same set of numbers 0,1 2... 9 are

repeated in a specific order to represent larger numbers.

The decimal number system is a positional number system as the position of a digit

represents its true magnitude. For example, 2 is less than 7, however 2 in 275 represents 200,

whereas 7 represents 70. The left most digit has the highest weight and the right most digit

has the lowest weight. 275 can be written in the form of an expression in terms of the base

value of the number system and weights.

2 x 102 + 7 x 101 + 5 x 100 = 200 + 70 + 5 = 275

where, 10 represents the base or radix

102, 101, 100 represent the weights 100, 10 and 1 of the numbers 2, 7 and 5

CS302 - Digital Logic & Design

Fractions in Decimal Number System

In a Decimal Number System the fraction part is separated from the Integer part by a

decimal point. The Integer part of a number is written on the left hand side of the decimal

point. The Fraction part is written on the right side of the decimal point. The digits of the

Integer part on the left hand side of the decimal point have weights 100, 101, 102 etc.

respectively starting from the digit to the immediate left of the decimal point and moving away

from the decimal point towards the most significant digit on the left hand side. Fractions in

decimal number system are also represented in terms of the base value of the number system

and weights. The weights of the fraction part are represented by 10-1, 10-2, 10-3 etc. The

weights decrease by a factor of 10 moving right of the decimal point. The number 382.91 in

terms of the base number and weights is represented as

3 x 102 + 8 x 101 + 2 x 100 + 9 x 10-1 + 1 x 10-2 = 300 + 80 + 2 + 0.9 + 0.01 = 382.91

Caveman number system

A number system discovered by archaeologists in a prehistoric cave indicates that the

caveman used a number system that has 5 distinct shapes ∑, Ć, >, Ω and ↑. Furthermore it

has been determined that the symbols ∑ to ↑ represents the decimal equivalents 0 to 5

respectively.

Centuries ago a caveman returning after a successful hunting expedition records his

successful hunt on the cave wall by carving out the numbers Ć↑. What does the number Ć↑

represent? The table 1.1 indicates that the Caveman numbers Ć↑ represents decimal number

Decimal Number

Caveman Number

Decimal Number

Caveman Number

∑

>∑

>Ć

Ω

>Ω

↑

>↑

Ć∑

Ω∑

ĆĆ

ΩĆ

Ć>

Ω>

ĆΩ

ΩΩ

Ć↑

Ω↑

↑∑

Table 1.1

Decimal equivalents of the Caveman Numbers

The Caveman is using a Base-5 number system. A Base-5 number system has five

unique symbols representing numbers 0 to 4. To represent numbers larger than 4, a

combination of 2, 3, 4 or more combinations of Caveman numbers are used. Therefore, to

represent the decimal number 5, a two number combination of the Caveman number system is

used. The most significant digit is Ć which is equivalent to decimal 1. The least significant digit

is ∑ which is equivalent to decimal 0. The five combinations of Caveman numbers having the

most significant digit Ć, represent decimal values 5 to 9 respectively. This is similar to the

Decimal Number system, where a 2-digit combination of numbers is used to represent values

CS302 - Digital Logic & Design

greater than 9. The most significant digit is set to 1 and the least significant digit varies from 0

to 9 to represent the next 10 values after the largest single decimal number digit 9.

The Caveman number Ć↑ can be written in expression form based on the Base value 5

and weights 50, 51, 52 etc.

= Ć x 51 + ↑ x 50 = Ć x 5 + ↑ x 1

Replacing the Caveman numbers Ć and ↑ with equivalent decimal values in the expression

yields

= Ć x 51 + ↑ x 50 = 1 x 5 + 4 x 1 = 9

The number ĆΩ↑∑ in decimal is represented in expression form as

Ć x 53 + Ω x 52 + ↑ x 51 + ∑ x 50 = Ć x 125 + Ω x 25 + ↑ x 5 + ∑ x 1

Replacing the Caveman numbers with equivalent decimal values in the expression yields

= (1) x 125 + (3) x 25 + (4) x 5 + (0) x 1 = 125 + 75 + 20 + 0 = 220

Binary Number System

The Caveman Number system is a hypothetical number system introduced to explain

that number system other than the Decimal Number system can exist and can be used to

represent and count numbers. Digital systems use a Binary number system. Binary as the

name indicates is a Base-2 number system having only two numbers 0 and 1. The Binary digit

0 or 1 is known as a `Bit'. Table 1.2

Decimal Number

Binary Number

Decimal Number

Binary Number

1010

1011

1100

1101

100

1110

101

1111

110

10000

111

10001

1000

10010

1001

10011

10100

Table 1.2

Decimal equivalents of Binary Number System

Counting in Binary Number system is similar to counting in Decimal or Caveman

Number systems. In a decimal Number system a value larger than 9 has to be represented by

2, 3, 4 or more digits. In the Caveman Number System a value larger than 4 has to be

represented by 2, 3, 4 or more digits of the Caveman Number System. Similarly, in the Binary

Number System a Binary number larger than 1 has to be represented by 2, 3, 4 or more binary

digits.

CS302 - Digital Logic & Design

Any binary number comprising of Binary 0 and 1 can be easily represented in terms of

its decimal equivalent by writing the Binary Number in the form of an expression using the

Base value 2 and weights 20, 21, 22 etc.

The number 100112 (the subscript 2 indicates that the number is a binary number and

not a decimal number ten thousand and eleven) can be rewritten in terms of the expression

100112 = (1 x 24) + (0 x 23) + (0 x 22) + (1 x 21) + (1 x 20)

= (1 x 16) + (0 x 8) + (0 x 4) + (1 x 2) + (1 x 1)

= 16 + 0 + 0 + 2 + 1

= 19

Fractions in Binary Number System

In a Decimal number system the Integer part and the Fraction part of a number are

separated by a decimal point. In a Binary Number System the Integer part and the Fraction

part of a Binary Number can be similarly represented separated by a decimal point. The Binary

number 1011.1012 has an Integer part represented by 1011 and a fraction part 101 separated

by a decimal point. The subscript 2 indicates that the number is a binary number and not a

decimal number. The Binary number 1011.1012 can be written in terms of an expression using

the Base value 2 and weights 23, 22, 21, 20, 2-1, 2-2 and 2-3.

1011.1012 = (1 x 23) + (0 x 22) + (1 x 21) + (1 x 20) + (1 x 2-1) + (0 x 2-2) + (1 x 2-3)

= (1 x 8) + (0 x 4) + (1 x 2) + (1 x 1) + (1 x 1/2) + (0 x 1/4) + (1 x 1/8)

= 8 + 0 + 2 + 1 + 0.5 + 0 + 0.125

= 11.625

Computers do handle numbers such as 11.625 that have an integer part and a fraction

part. However, it does not use the binary representation 1011.101. Such numbers are

represented and used in Floating-Point Numbers notation which will be discussed latter.

Table of Contents: