ZeePedia

Inventory Control:Manufacturing model with no shortages

<< Inventory Control:Purchasing model with shortages
Inventory Control:Manufacturing model with shortages >>
img
Operations Research (MTH601)
63
Rs.5×747×0.5
=
Shortage cost
2×12
= Rs. 77.75 per order
Total cost per order
= 3857 + 400 + 77.75 + 322.14
= Rs. 4656.89
Annual cost = Number of orders/year × cost
= 4.66 × 4656.89
= Rs. 21701.
Model 3: Manufacturing model with no shortages
In this model the following assumptions are made:
(1)
Demand is at a constant rate (D).
All cost coefficients (C1, C2, C3) are constants.
(2)
(3)
There is no shortage cost, or C4 = 0.
(4)
The replacement rate is finite and greater than the demand rate. This is also called replenishment rate
or manufacturing rate, denoted by R.
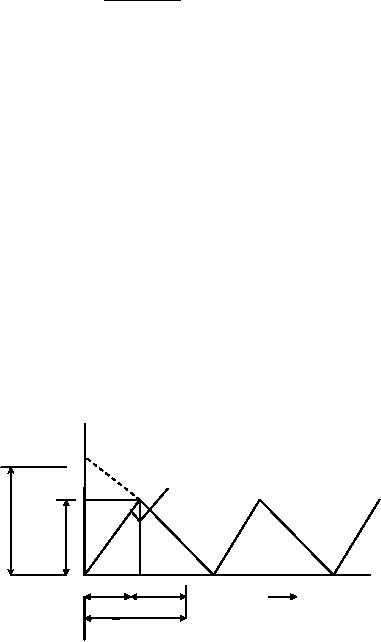
Schematically, this model is illustrated in fig 4
Slope= (R-D)
Slope = D
Q
Im
t2
Time
t1
t
Fig. 4
The total cost of inventory per period is the sum of three components: item cost, order cost and items holding cost.
Let Im be the maximum inventory, t1 be the time of manufacture and t2 be the time during which there is no supply.
In this model, all items required for a cycle are not stored at the beginning as in Wilson's Model. The items are
manufactured at a higher rate than the demand so that the difference (R­D) is the existing inventory till the items are
exhausted.
Item cost/period = C1Q
(32)
Order cost/period = C2
(33)
63
img
Operations Research (MTH601)
64
= C × I × (t1 + t2 ) 2
Item holding cost/period
(34)
m
3
= C3 I  m × t 2
(35)
= t ( R - D)
(36)
I
1
m
t =Q R
(37)
But
1
= (Q R)( R - D)
Therefore
(38)
I
m
Substituting the value of Im, we get the total cost of inventory per period.
C′ = C Q + C2 + C3 (Q R)( R - D) × t 2
(39)
1
Total cost of inventory per unit time
C = C′ t
(40)
t + C3 (Q R)( R - D) × t
= C Q t +C
(41)
2
1
2
But t = Q/D
Substituting the value of t we get
C = C D + C D Q + C3 (Q R) ( R-D) 2
(42)
1
2
Differentiating C with respect to Q and setting equal to zero for minimum C, we get,
C D  C ( R-D)
dC
= 0-  2  +  3
=0
(43)
dQ
2R
Q2
Solving equation (43), we get
2C2D
R
Q* =
(44)
R-D
C3
This gives the economic order quantity and is a balance between holding and set up costs.
64
img
Operations Research (MTH601)
65
Example: The demand for an item in a company is 18000 units/year and the company can produce at the rate of
3000 per month. The cost of one set up is Rs. 500 and the holding cost of 1 unit per month is 15 paisas. Determine:
(a)
The optimum manufacturing quantity.
(b)
The maximum inventory.
(c)
The time between orders.
(d)
The number of orders/year.
(e)
The time of manufacture.
(f)
The optimum annual cost if the cost of the item per unit is Rs. 2.
Assume no shortages.
Solution
C1 = Rs. 2 per item.
C2 = Rs. 500 per order.
C3 = Rs. 0.15 per item per month
D = 18000/year = 1500/month
R = 3000/month
a)
Optimum manufacture quantity
2C2D
R
Q* =
R-D
C3
2×500×1500×3000
=
= 4470 units
C 0.15(3000-1500)
b)
The maximum inventory
= Q ( R-D) R = 4470 × 1500 3000 = 2235 units
I
m
c)
The time between orders
t = Q D = 4470 1500 = 2.98 months
3 months
d)
The number of orders/year
N = 12 3 = 4
e)
The time of manufacture
t = Q R = 4470 3000 = 1.490 months
1
f)
The optimum annual cost
= Item cost + Ordering cost + Holding cost
65
Table of Contents:
  1. Introduction:OR APPROACH TO PROBLEM SOLVING, Observation
  2. Introduction:Model Solution, Implementation of Results
  3. Introduction:USES OF OPERATIONS RESEARCH, Marketing, Personnel
  4. PERT / CPM:CONCEPT OF NETWORK, RULES FOR CONSTRUCTION OF NETWORK
  5. PERT / CPM:DUMMY ACTIVITIES, TO FIND THE CRITICAL PATH
  6. PERT / CPM:ALGORITHM FOR CRITICAL PATH, Free Slack
  7. PERT / CPM:Expected length of a critical path, Expected time and Critical path
  8. PERT / CPM:Expected time and Critical path
  9. PERT / CPM:RESOURCE SCHEDULING IN NETWORK
  10. PERT / CPM:Exercises
  11. Inventory Control:INVENTORY COSTS, INVENTORY MODELS (E.O.Q. MODELS)
  12. Inventory Control:Purchasing model with shortages
  13. Inventory Control:Manufacturing model with no shortages
  14. Inventory Control:Manufacturing model with shortages
  15. Inventory Control:ORDER QUANTITY WITH PRICE-BREAK
  16. Inventory Control:SOME DEFINITIONS, Computation of Safety Stock
  17. Linear Programming:Formulation of the Linear Programming Problem
  18. Linear Programming:Formulation of the Linear Programming Problem, Decision Variables
  19. Linear Programming:Model Constraints, Ingredients Mixing
  20. Linear Programming:VITAMIN CONTRIBUTION, Decision Variables
  21. Linear Programming:LINEAR PROGRAMMING PROBLEM
  22. Linear Programming:LIMITATIONS OF LINEAR PROGRAMMING
  23. Linear Programming:SOLUTION TO LINEAR PROGRAMMING PROBLEMS
  24. Linear Programming:SIMPLEX METHOD, Simplex Procedure
  25. Linear Programming:PRESENTATION IN TABULAR FORM - (SIMPLEX TABLE)
  26. Linear Programming:ARTIFICIAL VARIABLE TECHNIQUE
  27. Linear Programming:The Two Phase Method, First Iteration
  28. Linear Programming:VARIANTS OF THE SIMPLEX METHOD
  29. Linear Programming:Tie for the Leaving Basic Variable (Degeneracy)
  30. Linear Programming:Multiple or Alternative optimal Solutions
  31. Transportation Problems:TRANSPORTATION MODEL, Distribution centers
  32. Transportation Problems:FINDING AN INITIAL BASIC FEASIBLE SOLUTION
  33. Transportation Problems:MOVING TOWARDS OPTIMALITY
  34. Transportation Problems:DEGENERACY, Destination
  35. Transportation Problems:REVIEW QUESTIONS
  36. Assignment Problems:MATHEMATICAL FORMULATION OF THE PROBLEM
  37. Assignment Problems:SOLUTION OF AN ASSIGNMENT PROBLEM
  38. Queuing Theory:DEFINITION OF TERMS IN QUEUEING MODEL
  39. Queuing Theory:SINGLE-CHANNEL INFINITE-POPULATION MODEL
  40. Replacement Models:REPLACEMENT OF ITEMS WITH GRADUAL DETERIORATION
  41. Replacement Models:ITEMS DETERIORATING WITH TIME VALUE OF MONEY
  42. Dynamic Programming:FEATURES CHARECTERIZING DYNAMIC PROGRAMMING PROBLEMS
  43. Dynamic Programming:Analysis of the Result, One Stage Problem
  44. Miscellaneous:SEQUENCING, PROCESSING n JOBS THROUGH TWO MACHINES
  45. Miscellaneous:METHODS OF INTEGER PROGRAMMING SOLUTION