 |
Applications of Basic Mathematics Part 5:DECREASE IN RATE |
| << Applications of Basic Mathematics Part 4:PERCENTAGE CHANGE |
| Applications of Basic Mathematics:NOTATIONS, ACCUMULATED VALUE >> |

MTH001
Elementary Mathematics
LECTURE
17
Applications of
Basic Mathematics
Part
5
OBJECTIVES
The
objectives of the lecture
are to learn about:
�
�
Discount
�
Simple
and compound
interest
�
Average
due date, interest on
drawings and
calendar
REVISION
LECTURE 5
A
chartered bank is lowering
the
interest
rate on its
loans
from
9%
to
7%.
What
will be the percent
decrease in the
interest rate on a
given
balance?
A
chartered bank is increasing
the
interest
rate on its
loans from
7%
to
9%
What
will be the percent
increase in the
interest rate on a
given
balance?
As
we learnt in lecture 5, the
calculation will be as
follows:
Decrease
in interest rate = 7-9 = -2
%
%
decrease = -2/9 x 100 =
-22.2 %
Increase
in interest rate = 9-7 = 2
%
%
decrease = 2/7 x 100 = 28.6
%
The
calculations in Excel are
shown in the following
slides:
DECREASE
IN RATE
Data
entry
Cell
F4 = 9
Cell
F5 = 7
Formulas
Formula
for decrease in Cell F6: =
=F5-F4
Formula
for % decrease in Cell F7:
=F6/F4*100
Results
Cell
F6 = -2%
Cell
F7 = -22.2%
INCREASE
IN RATE
Data
entry
Cell
F14 = 7
Cell
F15 = 9
Formulas
Formula
for increase in Cell F16:
=F15-F14
Formula
for % increase in Cell F17:
=F16/F14*100
Results
Cell
F16 = 2%
Cell
F17= 28.6%
Page
111
MTH001
Elementary Mathematics
The
Definition of a Stock
Plain
and simple, stock is a share
in the ownership of a company.
Stock
represents
a claim on the company's assets
and
earnings. As you
acquire more
Page
112

MTH001
Elementary Mathematics
stock,
your ownership stake in the
company becomes greater.
Whether you say
shares, equity, or stock, it
all means the same
thing.
Stock
yield
With
stocks, yield can refer to
the rate of income generated
from a stock in the
form
of
regular dividends. This is
often represented in percentage
form, calculated as
the
annual
dividend payments divided by
the stock's current share
price.
Earnings
per share (EPS)
The
EPS is the total profits of
a company divided by the
number of shares. A company
with
$1
billion in earnings and 200
million shares would have
earnings of $5 per
share.
Price-earnings
ratio
A
valuation ratio of a company's
current share price compared
to its per-share
earnings.
Calculated
as:
For
example, if a company is currently
trading at $43 a share and
earnings over the
last
12 months were $1.95 per
share, the P/E ratio
for the stock would be
22.05
($43/$1.95).
Outstanding
shares
Stock
currently held by investors,
including restricted shares
owned by the
company's
officers and insiders, as
well as those held by the
public. Shares that
have
been
repurchased by the company
are not considered
outstanding stock.
Net
current asset value per
share(NCAVPS)
NCAVPS
is calculated by taking a company's
current assets and
subtracting the total
liabilities,
and then dividing the
result by the total number
of shares outstanding.
Current
Assets
The
value of all assets that
are reasonably expected to be
converted into cash within
one
year
in the normal course of
business. Current assets
include cash, accounts
receivable,
inventory,
marketable securities, prepaid
expenses and other liquid
assets that can be
readily
converted to cash.
Liabilities
A
company's legal debts or
obligations that arise
during the course of
business operations.
Market
value
The
price at which investors buy
or sell a share of stock at a
given time
Face
value
Page
113

MTH001
Elementary Mathematics
Original
cost of a share of stock
which is shown on the
certificate. Also referred to as
"par
value."
Face
value is usually a very
small amount that bears no
relationship to its market
price.
Dividend
Usually,
a company distributes a part of
the profit it earns as
dividend.
For
example: A company may have
earned a profit of Rs 1 crore in
2003-04. It
keeps
half that amount within
the company. This will be
utilised on buying
new
machinery
or more raw materials or
even to reduce its borrowing
from the bank.
It
distributes the other half
as dividend.
Assume
that the capital of this
company is divided into
10,000 shares. That
would
mean half the profit -- ie
Rs 50 lakh (Rs 5 million) --
would be divided by
10,000
shares; each share would
earn Rs 500. The dividend
would then be Rs
500
per share. If you own
100 shares of the company,
you will get a cheque
of
Rs
50,000 (100 shares x Rs 500)
from the company.
Sometimes,
the dividend is given as a
percentage -- i e the company
says it has
declared
a dividend of 50 percent. It's
important to remember that
this dividend is
a
percentage of the share's
face value. This means, if
the face value of
your
share
is Rs 10, a 50 percent dividend
will mean a dividend of Rs 5
per share
BUYING
SHARES
If
you buy 100 shares at
Rs. 62.50 per share
with a 2% commission,
calculate
your
total cost.
Calculation
100
* Rs. 62.50 = Rs.
6,250
0.02
* Rs. 6,250 =
125
Total
=
Rs. 6,375
RETURN
ON INVESTMENT
Suppose
you bought 100 shares at
Rs. 52.25 and sold
them after 1 year at
Rs.
68.
With a 1% commission rate of
buying and selling the
stock and 10 %
dividend
per share is due on these
shares. Face value of each
share is 10Rs.
What
is your return on
investment?
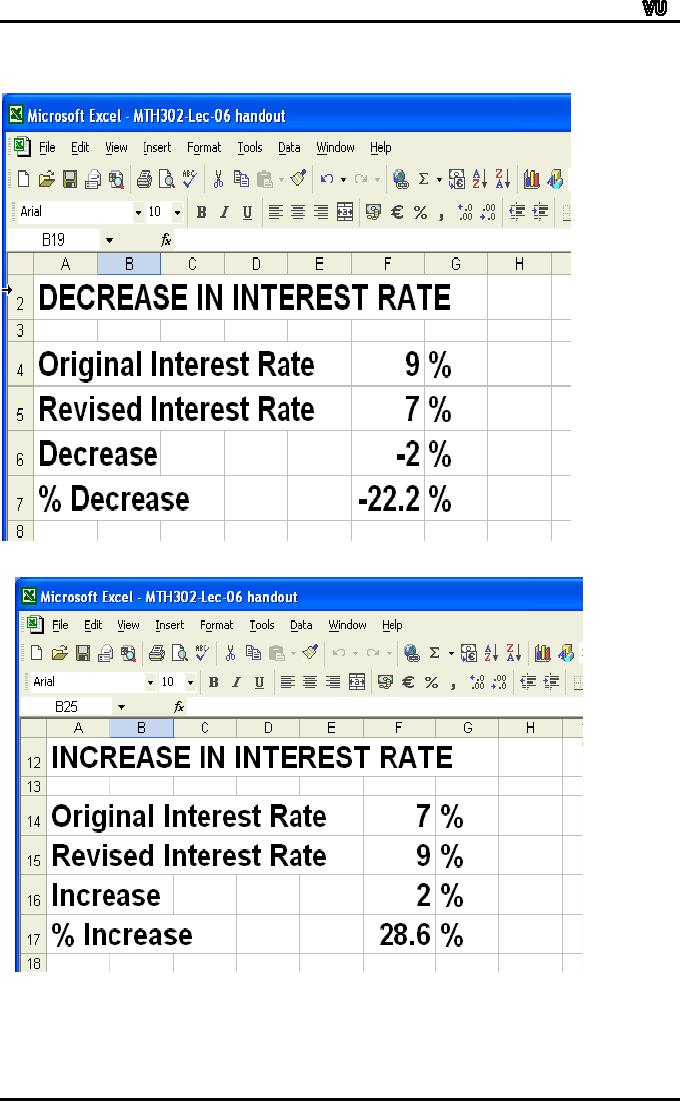
Bought
Page
114

MTH001
Elementary Mathematics
100
shares at Rs. 52.25 =
5,225.00
Commission
at 1%
=
52.25
Total
Costs
=5,225
+ 52.25 = 5,277.25
Sold
100
shares at Rs. 68
=
6,800.00
Commission
at 1%
=
- 68.00
Total
Costs Sale
=
6,800 68 = 6,732.00
Gain
Net
receipts
=
6,732.00
Total
cost
=
- 5,277.25
Net
Gain
=
6,732 5,277.25
=1,454.75
Dividends
(100*10/10)
=
100.00
Total
Gain
=
1,454.75 + 100 =
1,554.75
Return
on investment
=
1,554.75/5,277.25*100
=
29.46 %
The
calculations using Excel
were made as follows:
BOUGHT
Data
entry
Cell
B21: 100
Cell
B22: 52.25
Formulas
Formula
for Cost of 100 shares at
Rs. 52.25 in Cell B23:
=B21*B22
Formula
for Commission at 1% in Cell
B24: =B23*0.01
Formula
for Total Costs in Cell
B25: =B23+B24
Results
Cell
B23 = 5225
Cell
B24 = 52.25
Cell
B25 = 5277.25
Page
115
MTH001
Elementary Mathematics
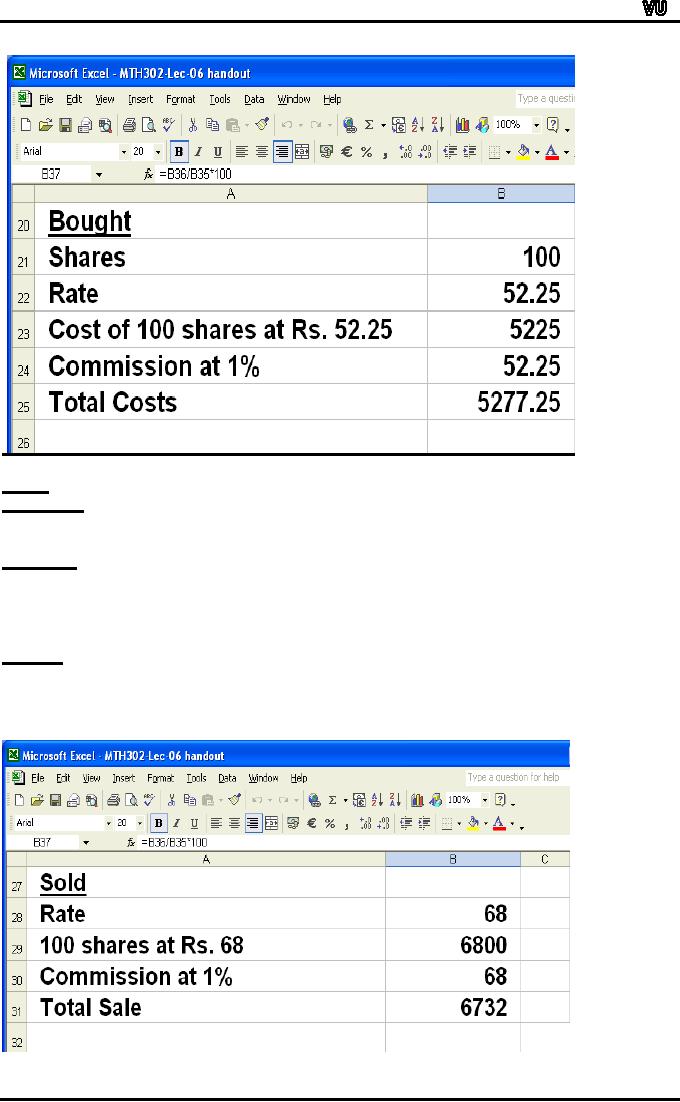
SOLD
Data
entry
Cell
B28: 68
Formulas
Formula
for sale of 100 shares at
Rs. 68 in Cell B29:
=B21*B28
Formula
for Commission at 1% in Cell
B30: =B29*0.01
Formula
for Total Sale in Cell
B31: =B29-B30
Results
Cell
B29 = 6800
Cell
B30 = 68
Cell
B31 = 6732
Page
116
MTH001
Elementary Mathematics
GAIN
Formulas
Formula
for Net receipts in Cell
B34: =B31
Formula
for Total cost in Cell
B35: =B25
Formula
for Net Gain in Cell
B36: =B31-B25
Formula
for % Gain in Cell B37:
=B36/B35*100
Results
Cell
B34 = 6732
Cell
B35 = 5277.25
Cell
B36 = 1454.75
Cell
B37 = 27.57
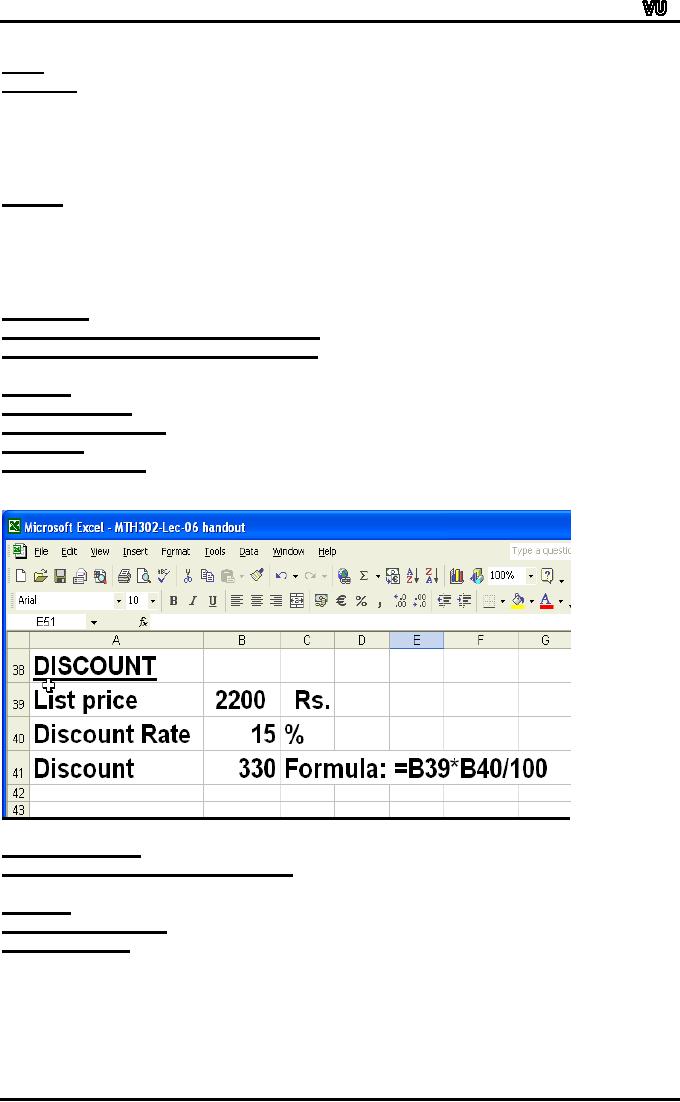
DISCOUNT
Discount
is Rebate or reduction in
price.
Discount
is expressed as % of list
price.
Example
List
price = 2200
Discount
Rate = 15%
Discount?
=
2200 x 0.15= 330
Calculation
using Excel along with
formula is given in the
following slide:
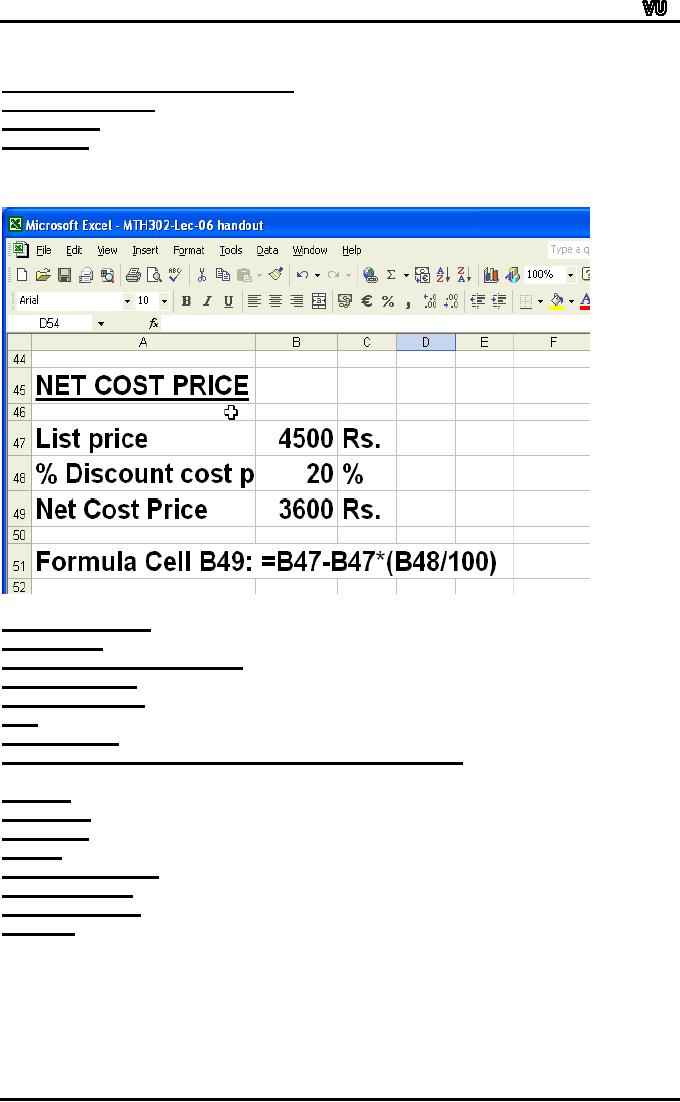
NET
COST PRICE
Net
Cost Price = List price -
Discount
Example
List
price = 4,500 Rs.
Discount
= 20 %
Netcost
price?
Page
117
MTH001
Elementary Mathematics
Net
cost price = 4,500 20 % of
4,500
=
4,500 0.2
x4,500
=4,500
900
=
3,600 Rs.
Calculation
using Excel along with
formula is given in the
following slide:
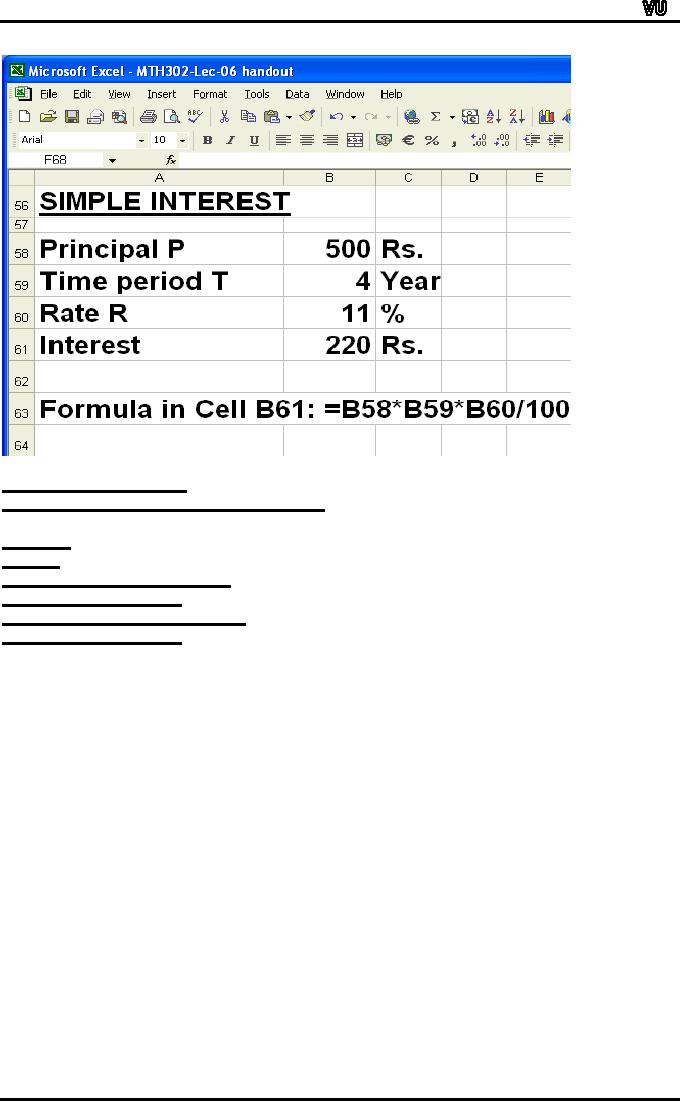
SIMPLE
INTEREST
P
= Principal
R
= Rate of interest per
annum
T
= Time in years
I
= Simple interest
then
I
= P. R. T / 100
Thus
total amount A to be paid at
the end of T years = P +
I
Example
P
= Rs. 500
T
= 4 years
R
=11%
Find
simple interest
I
= P x T x R /100
=
500 x 4 x 11/100
=
Rs. 220
Calculation
using Excel along with
formula is given in the
following slide:
Page
118
MTH001
Elementary Mathematics
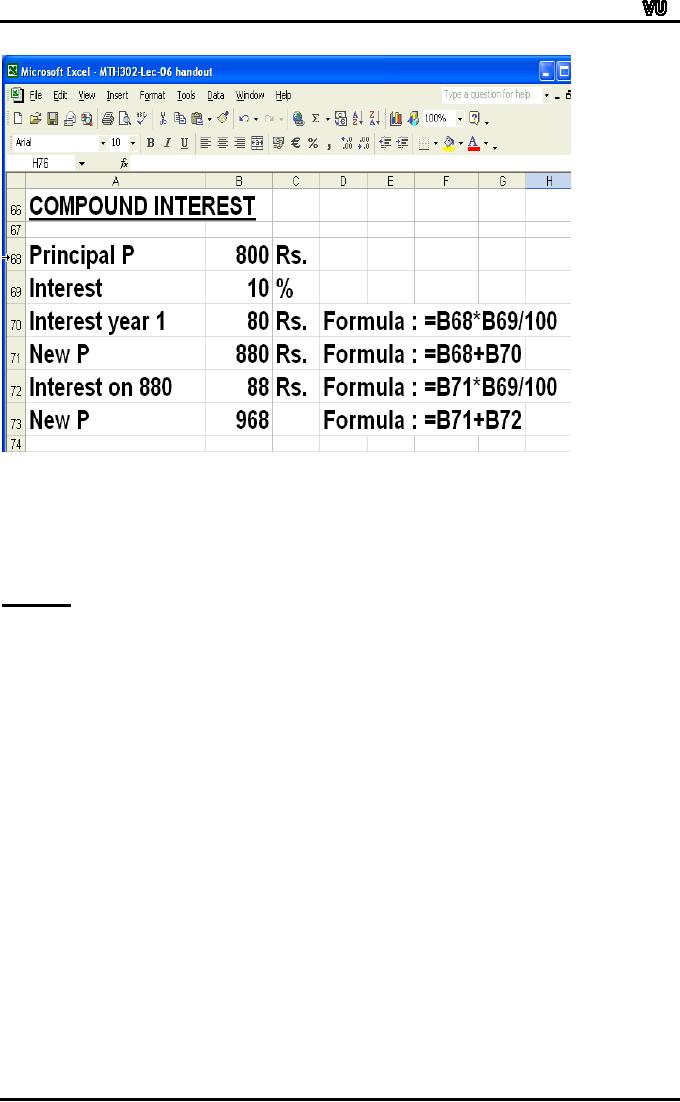
COMPOUND
INTEREST
Compound
Interest also attracts
interest.
Example
P
= 800
Interest
year 1= 0.1 x 800=
80
New
P = 800 + 80 = 880
Interest
on 880 = 0.1 X 880 =
88
New
P = 880 + 88 = 968
Calculation
using Excel along with
formula is given in the
following slide:
Page
119
MTH001
Elementary Mathematics
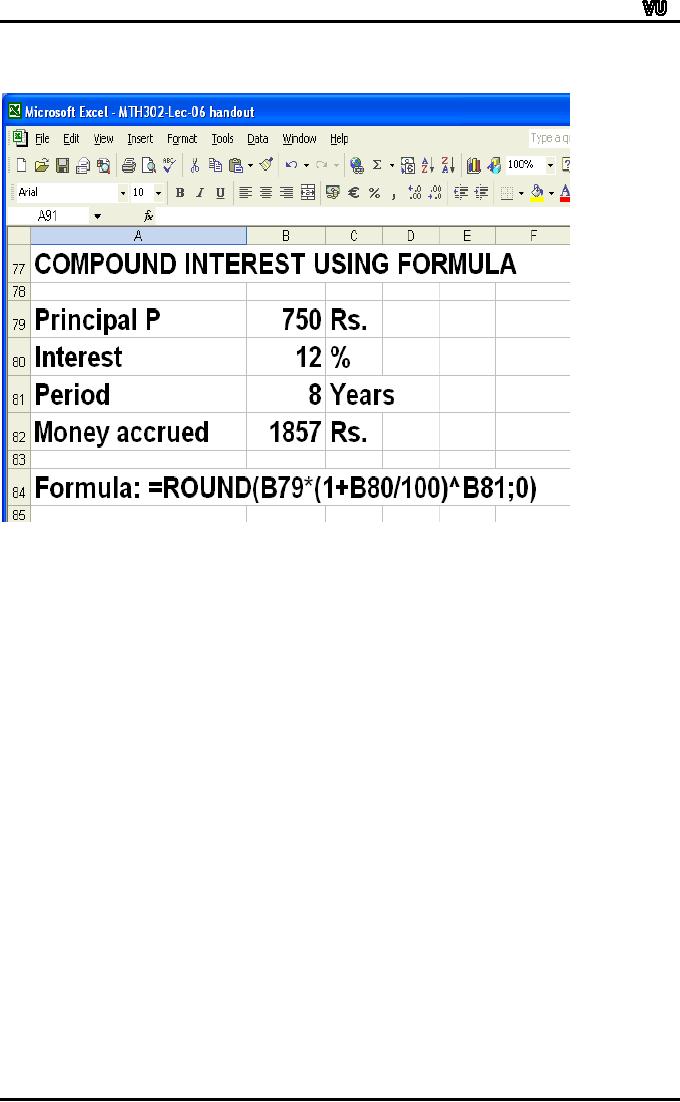
Co
mpound
Interest Formula
S
= Money accrued after n
years also called compound
amount
P
= Principal
r
= Rate of interest
n
= Number of periods
S
= P(1 + r/100)^ n
Compound
interest = S - P
Example
Calculate
compound interest earned on
Rs. 750 invested at 12%
per annum for
8
years.
S=
P(1+r/100)^8
=
750(1+12/100)^8
=1857
Rs
Compound
interest = 1857 750 =
1107 Rs
Page
120
MTH001
Elementary Mathematics
Calculation
using Excel along with
formula is given in the
following
slide
Page
121
Table of Contents:
- Recommended Books:Set of Integers, SYMBOLIC REPRESENTATION
- Truth Tables for:DE MORGAN’S LAWS, TAUTOLOGY
- APPLYING LAWS OF LOGIC:TRANSLATING ENGLISH SENTENCES TO SYMBOLS
- BICONDITIONAL:LOGICAL EQUIVALENCE INVOLVING BICONDITIONAL
- BICONDITIONAL:ARGUMENT, VALID AND INVALID ARGUMENT
- BICONDITIONAL:TABULAR FORM, SUBSET, EQUAL SETS
- BICONDITIONAL:UNION, VENN DIAGRAM FOR UNION
- ORDERED PAIR:BINARY RELATION, BINARY RELATION
- REFLEXIVE RELATION:SYMMETRIC RELATION, TRANSITIVE RELATION
- REFLEXIVE RELATION:IRREFLEXIVE RELATION, ANTISYMMETRIC RELATION
- RELATIONS AND FUNCTIONS:FUNCTIONS AND NONFUNCTIONS
- INJECTIVE FUNCTION or ONE-TO-ONE FUNCTION:FUNCTION NOT ONTO
- SEQUENCE:ARITHMETIC SEQUENCE, GEOMETRIC SEQUENCE:
- SERIES:SUMMATION NOTATION, COMPUTING SUMMATIONS:
- Applications of Basic Mathematics Part 1:BASIC ARITHMETIC OPERATIONS
- Applications of Basic Mathematics Part 4:PERCENTAGE CHANGE
- Applications of Basic Mathematics Part 5:DECREASE IN RATE
- Applications of Basic Mathematics:NOTATIONS, ACCUMULATED VALUE
- Matrix and its dimension Types of matrix:TYPICAL APPLICATIONS
- MATRICES:Matrix Representation, ADDITION AND SUBTRACTION OF MATRICES
- RATIO AND PROPORTION MERCHANDISING:Punch recipe, PROPORTION
- WHAT IS STATISTICS?:CHARACTERISTICS OF THE SCIENCE OF STATISTICS
- WHAT IS STATISTICS?:COMPONENT BAR CHAR, MULTIPLE BAR CHART
- WHAT IS STATISTICS?:DESIRABLE PROPERTIES OF THE MODE, THE ARITHMETIC MEAN
- Median in Case of a Frequency Distribution of a Continuous Variable
- GEOMETRIC MEAN:HARMONIC MEAN, MID-QUARTILE RANGE
- GEOMETRIC MEAN:Number of Pupils, QUARTILE DEVIATION:
- GEOMETRIC MEAN:MEAN DEVIATION FOR GROUPED DATA
- COUNTING RULES:RULE OF PERMUTATION, RULE OF COMBINATION
- Definitions of Probability:MUTUALLY EXCLUSIVE EVENTS, Venn Diagram
- THE RELATIVE FREQUENCY DEFINITION OF PROBABILITY:ADDITION LAW
- THE RELATIVE FREQUENCY DEFINITION OF PROBABILITY:INDEPENDENT EVENTS