APPLICATION OF PRESENT VALUE CONCEPTS:Compound Annual Rates

<< TIME VALUE OF MONEY:Future Value, Present Value

BOND PRICING & RISK:Valuing the Principal Payment, Risk >>

Money & Banking MGT411

Lesson 9

APPLICATION OF PRESENT VALUE CONCEPTS

Application of Present Value Concept

Compound Annual Rate

Interest Rates vs. Discount Rate

Internal Rate of Return

Bond Pricing

Important Properties of Present Value

Present Value is higher:

The higher the future value (FV) of the payment

The shorter the time period until payment (n)

The lower the interest rate (i)

The size of the payment (FVn)

Doubling the future value of the payment (without changing time of the payment or interest

rate), doubles the present value

At 5% interest rate, $100 payment has a PV of $90.70

Doubling it to $200, doubles the PV to $181.40

Increasing or decreasing FVn by any percentage will change PV by the same percentage in the

same direction

The time until the payment is made (n)

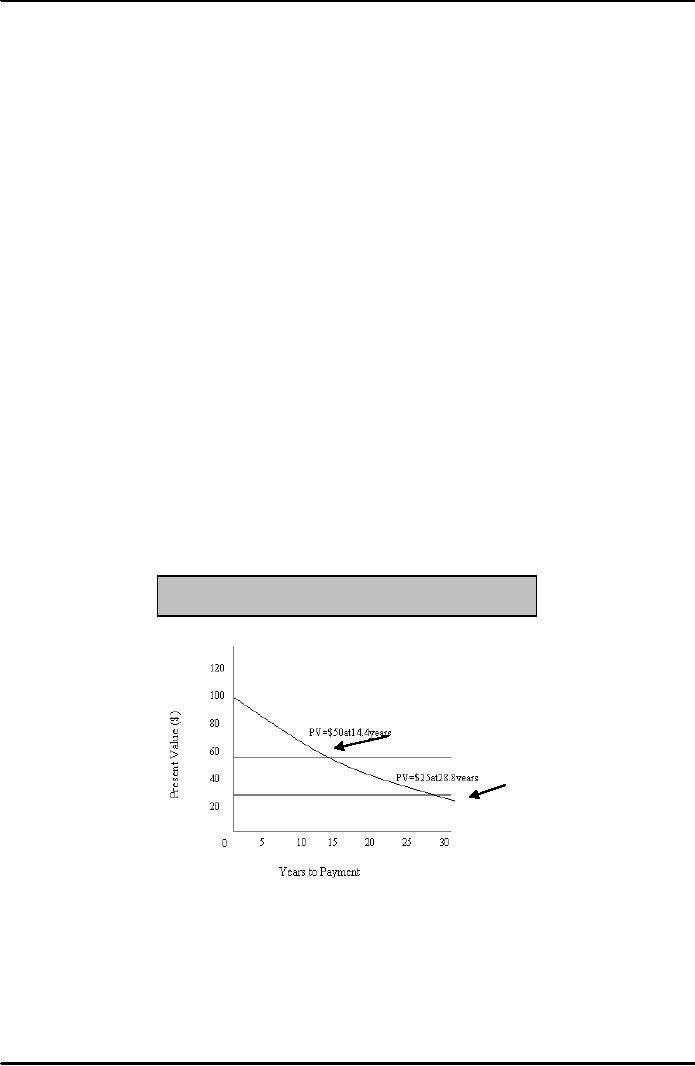
Continuing with the previous example of $100 at 5%, we allow the time to go from 0 to 30

years.

This process shows us that the PV payment is worth $100 if it is made immediately, but

gradually declines to $23 for a payment made in 30 years

Figure: Present value of $100 at 5% interest rate

The rule of 72

For reasonable rates of return, the time it takes to double the money, is given approximately by

t = 72 / i%

If we have an interest rate of 10%, the time it takes for investment to double is:

t = 72 / 10 = 7.2 years

This rule is fairly applicable to discount rates in 5% to 20% range.

Money & Banking MGT411

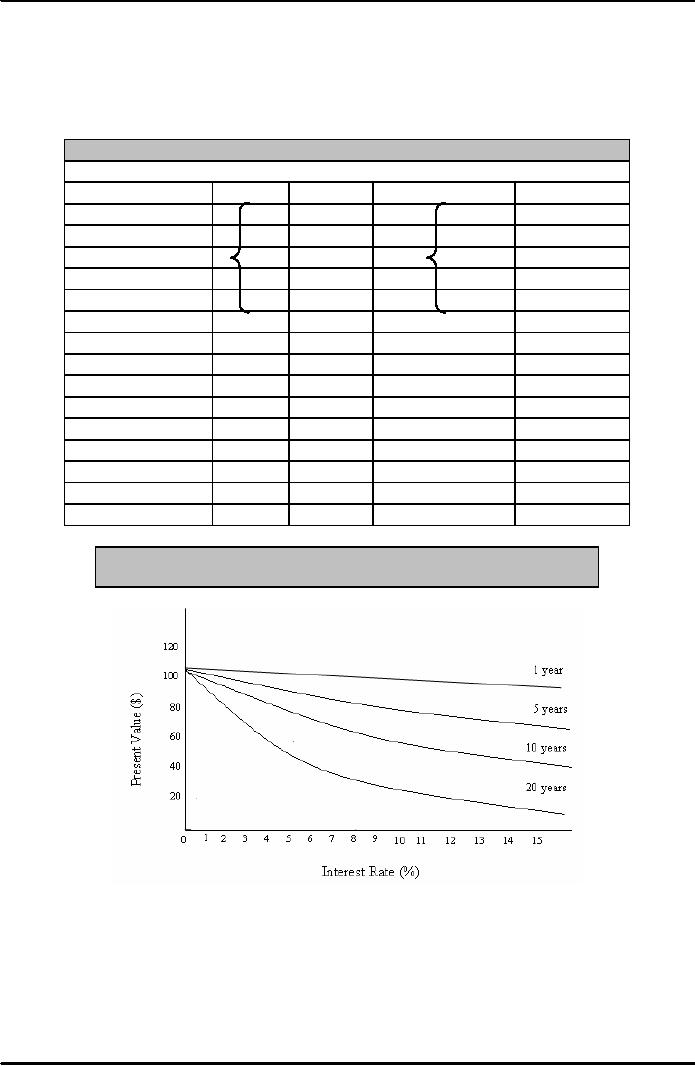
The Interest rate (i)

Higher interest rates are associated with lower present values, no matter what size or timing of

the payment

At any fixed interest rate, an increase in the time until a payment is made reduces its present

value

Table: Present Value of a $100 payment

Payment due in

Interest rate

1 Year 5 Years

10 Years

20 Years

$99.01

$95.15

$90.53

$81.95

2% $3.77 $98.04

$90.57 $29.14 $82.03

$67.30

3% 4% $97.09

$86.26 32%

$74.41

$55.37

$96.15

$82.19

$67.56

$45.64

$95.24

$78.35

$61.39

$37.69

$94.34

$74.73

$55.84

$31.18

$93.46

$71.30

$50.83

$25.84

$92.59

$68.06

$46.32

$21.45

$91.74

$64.99

$42.24

$17.84

10%

$90.91

$62.09

$38.55

$14.86

11%

$90.09

$59.35

$35.22

$12.40

12%

$89.29

$56.74

$32.20

$10.37

13%

$88.50

$54.28

$29.46

$8.68

14%

$87.72

$51.94

$26.97

$7.28

15%

$86.96

$49.72

$24.72

$6.11

Figure: The relationship between Present value and Interest Rates

Compound Annual Rates

Comparing changes over days, months, years and decades can be very difficult.

The way to deal with such problems is to turn the monthly growth rate into compound-annual

rate.

An investment whose value grows 0.5% per month goes from 100 at the beginning of the month

to 100.5 at the end of the month:

We can verify this as following

Money & Banking MGT411

100 (100.5 - 100) = [(100.5/100) 1] = 0.5%

100

What if the investment's value continued to grow at 0.5% per month for next 12 months?

We cant simply multiply 0.5 by 12

Instead we need to compute a 12 month compound rate

So the future value of 100 at 0.5 %( 0.005) per month compounded for 12 months will be:

FVn = PV (1+i) n = 100(1.005)12 = 106.17

An increase of 6.17% which is greater than 6%, had we multiplied 0.5% by 12

The difference between the two answers grows as the interest rate grows

At 1% monthly rate, 12 month compounded rate is12.68%

Another use for compounding is to compute the percentage change per year when we know how

much an investment has grown over a number of years

This rate is called average annual rate

If an investment has increased 20%, from 100 to 120 over 5 years

Is average annual rate is simply dividing 20% by 5?

This way we ignore compounding effect

Increase in 2nd year must be calculated as percentage of the investment worth at the end of 1st

year

To calculate the average annual rate, we revert to the same equation:

FVn = PV (1+i) n

120 = 100(1 + i) 5

Solving for i

i = [(120/100)1/5 - 1] = 0.0371

5 consecutive annual increases of 3.71% will result in an overall increase of 20%

Interest Rate and Discount Rate

The interest rate used in the present value calculation is often referred to as the discount rate

because the calculation involves discounting or reducing future payments to their equivalent

value today.

Another term that is used for the interest rate is yield

Saving behavior can be considered in terms of a personal discount rate;

People with a low rate are more likely to save, while people with a high rate are more likely to

borrow

We all have a discount rate that describes the rate at which we need to be compensated for

postponing consumption and saving our income

If the market offers an interest rate higher than the individual's personal discount rate, we would

expect that person to save (and vice versa)

Higher interest rates mean higher saving

Applying Present Value

To use present value in practice we need to look at a sequence or stream of payments whose

present values must be summed. Present value is additive.

To see how this is applied we will look at internal rate of return and the valuation of bonds

Internal Rate of Return

The Internal Rate of Return is the interest rate that equates the present value of an investment

with it cost.

It is the interest rate at which the present value of the revenue stream equals the cost of the

investment project.

Money & Banking MGT411

In the calculation we solve for the interest rate

A machine with a price of $1,000,000 that generates $150,000/year for 10 years

$ 150 , 000

$ 1, 000 , 000 =

+ ...... +

(1 + i ) 1

(1 + i ) 2

(1 + i ) 3

(1 + i ) 10

Solving for i, i=.0814 or 8.14%

The internal rate of return must be compared to a rate of interest that represents the cost of funds

to make the investment.

These funds could be obtained from retained earnings or borrowing. In either case there is an

interest cost

An investment will be profitable if its internal rate of return exceeds the cost of borrowing

Table of Contents: