 |
Money
& Banking MGT411
VU
Lesson
17
TAX
EFFECT & TERM STRUCTURE OF
INTEREST RATE
Tax
Effect
Term
Structure of Interest Rate
Expectations
Hypothesis
Liquidity
Premium
Tax
Effect
The
second important factor that
affects the return on a bond is
taxes
Bondholders
must pay income tax on the interest
income they receive from
privately issued
bonds
(taxable bonds), but government
bonds are treated
differently
Interest
payments on bonds issued by
state and local governments,
called "municipal" or
"tax-
exempt"
bonds are specifically exempt
from taxation
A
tax exemption affects a bond's
yield because it affects how
much of the return the
bondholder
gets
to keep
Tax-Exempt
Bond Yield = (Taxable Bond
Yield) x (1- Tax
Rate).
Term
Structure of Interest Rates
The
relationship among bonds with the
same risk characteristics
but different maturities
is
called
the term structure of interest rates.
A
plot of the term structure, with the
yield to maturity on the vertical
axis and the time to
maturity
on the horizontal axis, is called the
yield curve.
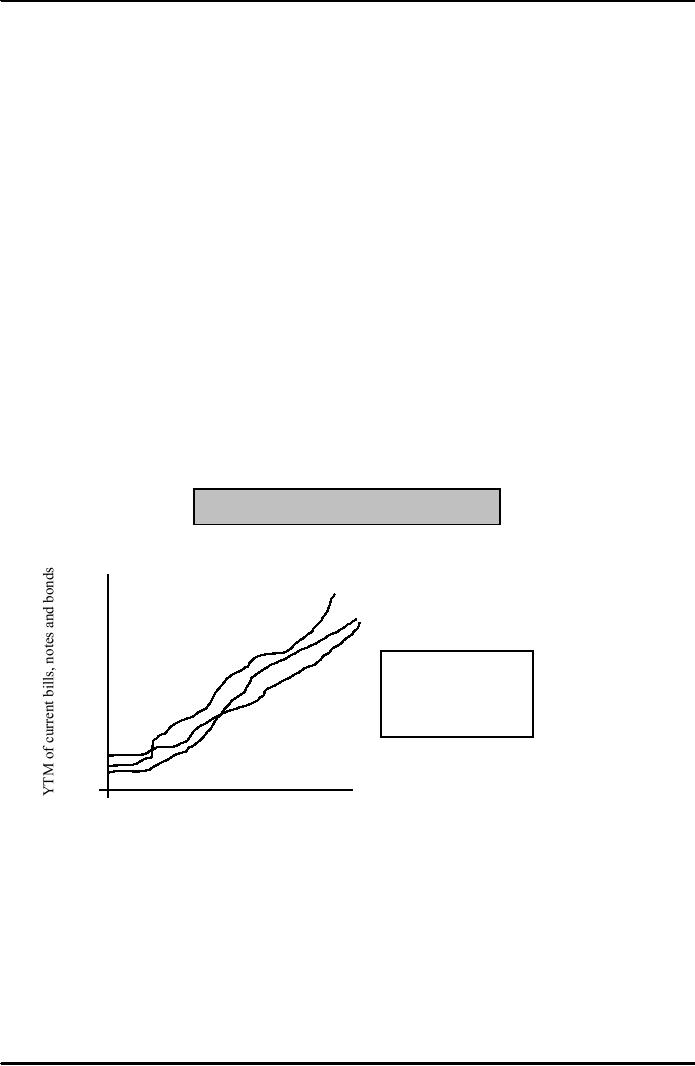
Figure:
The U.S. Treasury Yield
Curve
___Yesterday
6.0%
___1
month ago
___1
year ago
5.0
The
figure plots the
4.0
yields
on Treasury
bills
and bonds for
3.0
August
27, 2004.
2.0
1.0
0
1
3
6
2
5
10
30
Months
Years
________
Maturity________
55
Money
& Banking MGT411
VU
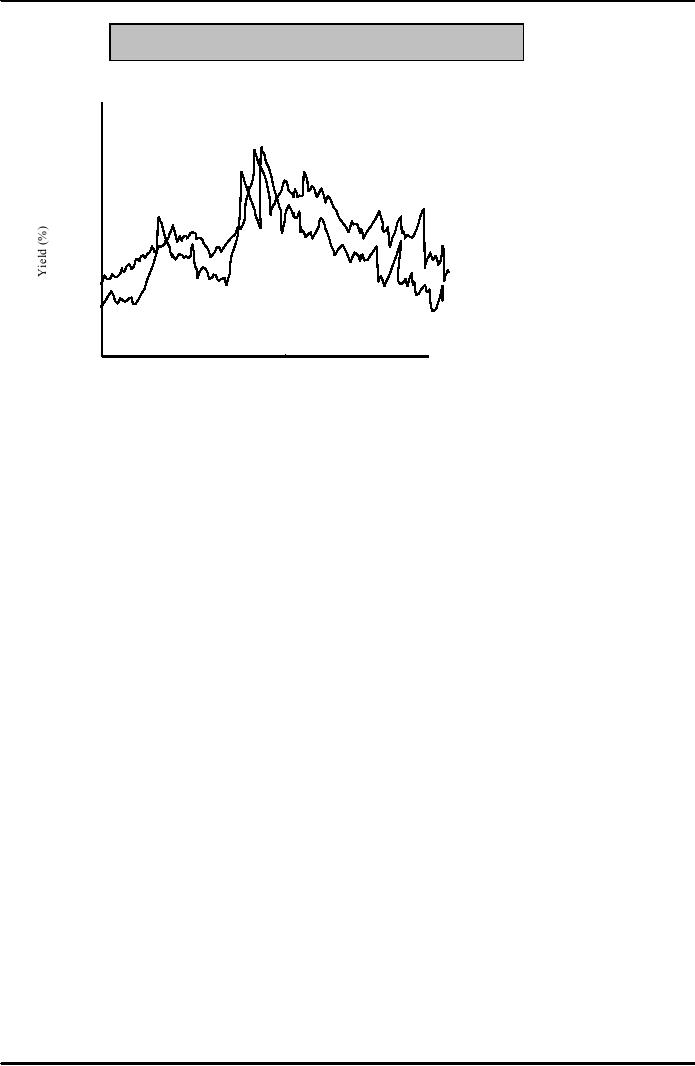
Figure:
The Term Structure of Treasury Interest
Rates
18
16
14
12
10
8
6
4
2
0
1979
1983
1987
1991
1995 1999
1971
11975
2003
___3
months T.Bills_____10 years
T-Bonds
Term
Structure "Facts"
Interest
Rates of different maturities
tend to move together
Yields
on short-term bond are more volatile
than yields on long-term
bonds
Long-term
yields tend to be higher
than short-term yields.
Expectations
Hypothesis
The
risk-free interest rate can be computed,
assuming that there is no uncertainty
about the
future
Since
certainty means that bonds
of different maturities are
perfect substitutes for each
other, an
investor
would be indifferent between
holding
A
two-year bond or
A
series of two one-year
bonds
Certainty
means that bonds of
different maturities are
perfect substitutes for each
other
Assuming
that current 1-year interest rate is
5%. The expectations hypothesis implies
that the
current
2-year interest rate should equal the
average of 5% and 1-year interest rate one
year in
future.
If
future interest rate is 7%, then
current 2-year interest rate will be
(5+7) / 2 = 6%
Therefore,
when interest rates are expected to rise
long-term rates will be
higher than short-term
rates
and the yield curve will
slope up (and vice versa)
56
Money
& Banking MGT411
VU
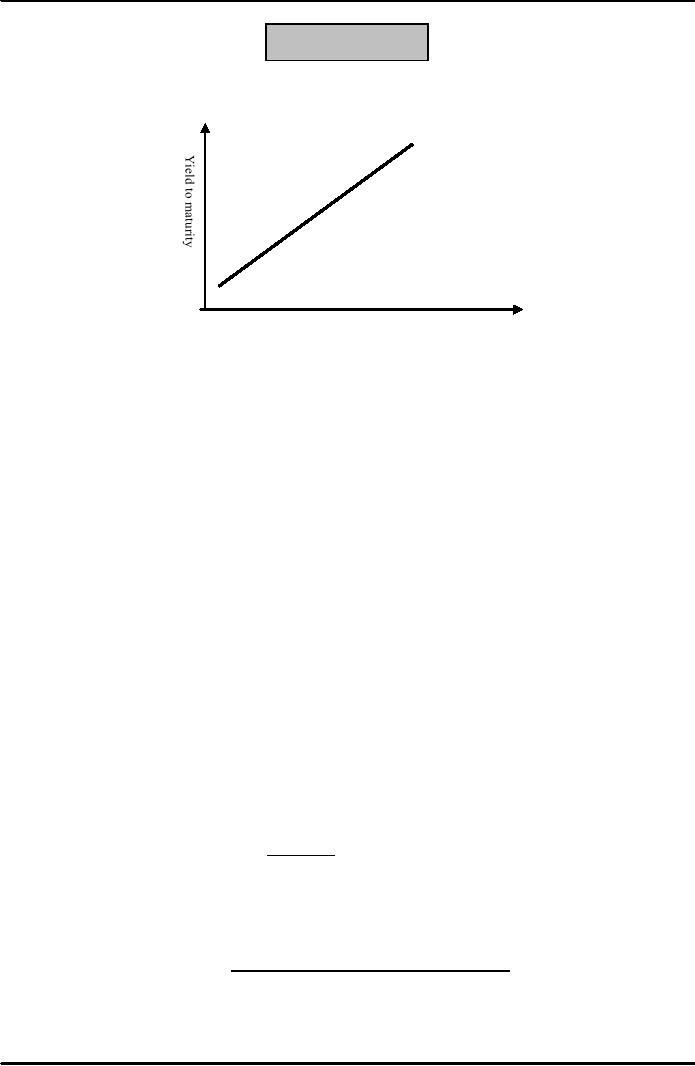
Figure:
Yield Curve
Yield
curve when interest rates
are expected to
rise
Time
to maturity
From
this we can construct investment
strategies that must have the
same yield.
Assuming
the investor has a two-year
horizon, the investor
can:
Invest
in a two-year bond and hold it to
maturity
Interest
rate will be i2y
Investment
will yield (1 + i2y)
(1 + i2y) two years
later
Invest
in a one-year bond today and a
second one a year from now
when the first one
matures
Interest
rate will be iey+1
Investment
will yield (1 + i1y)
(1 + iey+1) in two years
The
hypothesis tells us that investors will
be indifferent between the two
strategies, so the
strategies
must have the same
return
Total
return from 2 year bonds
over 2 years
(1
+
i
2y )(1 +
i
2y )
Return
from one year bond and then
another one year bond
(1
+
i
1y )(1 +
i
1y )
e
If
one and two year bonds are
perfect substitutes,
then:
(1
+
i
2y )(1 +
i
2y ) =
(1
+
i
1y )(1 +
1y
)
e
Or
i1y +
i1y
2
i
2y =
2
Or
in general terms
i1
t
+
i1et
+
1
+
i1et
+
2
+
....
+
i1et
+
n
-
1
=
i
nt
n
Therefore
the rate on the two-year bond
must be the average of the current
one-year rate and the
expected
future one-year rate
57

Money
& Banking MGT411
VU
Implications
would be the same old
Interest
rates of different maturities
tend to move
together.
Yields
on short-term bonds are more volatile
than those on long-term
bonds.
Long-term
yields tend to be higher
than short-term yields
However,
expectations theory can not
explain why long-term rates
are usually above short term
rates
In
order to explain why the
yield curve normally slopes
upward, we need to extend
the
hypothesis
to include risk
58
Table of Contents:
- TEXT AND REFERENCE MATERIAL & FIVE PARTS OF THE FINANCIAL SYSTEM
- FIVE CORE PRINCIPLES OF MONEY AND BANKING:Time has Value
- MONEY & THE PAYMENT SYSTEM:Distinctions among Money, Wealth, and Income
- OTHER FORMS OF PAYMENTS:Electronic Funds Transfer, E-money
- FINANCIAL INTERMEDIARIES:Indirect Finance, Financial and Economic Development
- FINANCIAL INSTRUMENTS & FINANCIAL MARKETS:Primarily Stores of Value
- FINANCIAL INSTITUTIONS:The structure of the financial industry
- TIME VALUE OF MONEY:Future Value, Present Value
- APPLICATION OF PRESENT VALUE CONCEPTS:Compound Annual Rates
- BOND PRICING & RISK:Valuing the Principal Payment, Risk
- MEASURING RISK:Variance, Standard Deviation, Value at Risk, Risk Aversion
- EVALUATING RISK:Deciding if a risk is worth taking, Sources of Risk
- BONDS & BONDS PRICING:Zero-Coupon Bonds, Fixed Payment Loans
- YIELD TO MATURIRY:Current Yield, Holding Period Returns
- SHIFTS IN EQUILIBRIUM IN THE BOND MARKET & RISK
- BONDS & SOURCES OF BOND RISK:Inflation Risk, Bond Ratings
- TAX EFFECT & TERM STRUCTURE OF INTEREST RATE:Expectations Hypothesis
- THE LIQUIDITY PREMIUM THEORY:Essential Characteristics of Common Stock
- VALUING STOCKS:Fundamental Value and the Dividend-Discount Model
- RISK AND VALUE OF STOCKS:The Theory of Efficient Markets
- ROLE OF FINANCIAL INTERMEDIARIES:Pooling Savings
- ROLE OF FINANCIAL INTERMEDIARIES (CONTINUED):Providing Liquidity
- BANKING:The Balance Sheet of Commercial Banks, Assets: Uses of Funds
- BALANCE SHEET OF COMMERCIAL BANKS:Bank Capital and Profitability
- BANK RISK:Liquidity Risk, Credit Risk, Interest-Rate Risk
- INTEREST RATE RISK:Trading Risk, Other Risks, The Globalization of Banking
- NON- DEPOSITORY INSTITUTIONS:Insurance Companies, Securities Firms
- SECURITIES FIRMS (Continued):Finance Companies, Banking Crisis
- THE GOVERNMENT SAFETY NET:Supervision and Examination
- THE GOVERNMENT'S BANK:The Bankers' Bank, Low, Stable Inflation
- LOW, STABLE INFLATION:High, Stable Real Growth
- MEETING THE CHALLENGE: CREATING A SUCCESSFUL CENTRAL BANK
- THE MONETARY BASE:Changing the Size and Composition of the Balance Sheet
- DEPOSIT CREATION IN A SINGLE BANK:Types of Reserves
- MONEY MULTIPLIER:The Quantity of Money (M) Depends on
- TARGET FEDERAL FUNDS RATE AND OPEN MARKET OPERATION
- WHY DO WE CARE ABOUT MONETARY AGGREGATES?The Facts about Velocity
- THE FACTS ABOUT VELOCITY:Money Growth + Velocity Growth = Inflation + Real Growth
- THE PORTFOLIO DEMAND FOR MONEY:Output and Inflation in the Long Run
- MONEY GROWTH, INFLATION, AND AGGREGATE DEMAND
- DERIVING THE MONETARY POLICY REACTION CURVE
- THE AGGREGATE DEMAND CURVE:Shifting the Aggregate Demand Curve
- THE AGGREGATE SUPPLY CURVE:Inflation Shocks
- EQUILIBRIUM AND THE DETERMINATION OF OUTPUT AND INFLATION
- SHIFTS IN POTENTIAL OUTPUT AND REAL BUSINESS CYCLE THEORY