CAPITAL BUDGETING AND CAPITAL BUDGETING TECHNIQUES:Techniques of capital budgeting, Pay back period

<< DISCOUNTING CASH FLOW ANALYSIS, ANNUITIES AND PERPETUITIES:Multiple Compounding

NET PRESENT VALUE (NPV) AND INTERNAL RATE OF RETURN (IRR):RANKING TWO DIFFERENT INVESTMENTS >>

Financial Management MGT201

Lesson 08

CAPITAL BUDGETING AND CAPITAL BUDGETING TECHNIQUES

Learning Objective:

After going through this lecture, you would be able to have an understanding of the following concepts.

· Capital Budgeting

Techniques of Capital Budgeting

Today, we will discuss Capital Budgeting--one of the most important topics in financial

management.

Capital budgeting is about investment in fixed assets. In addition, another type of investment could

be in working capital, which we would study later. Fixed assets are the part of long-term assets in the

balance sheet and working capital is net position of current assets and current liabilities on the balance

sheet.

We need to understand why capital budgeting is so important and why do we have to invest in fixed

assets? The answer is simple; the equipment or machinery and other fixed assets depreciate over a

period, they lose their productivity and get obsolete after sometime. These assets need to be replaced

with new assets. This replacement involves investment in fixed assets.

Moreover, if a company intends to start a new project, Capital Budgeting techniques are employed

to assess the financial viability of the project. Suppose, for instance, a company wants to introduce a

new soap and launching of the new product demands changes in the manufacturing process, the

company will have to purchase new equipment in the form of fixed assets. Capital budgeting is a

technique used to evaluate the value of investment and projects in fixed assets. It is also used to assess

the working capital requirements. Combined together it helps the company management to decide

whether the new venture should be taken up or not.

Capital budgeting is a decentralized function. In big corporations, this function is not an individual's

job, rather, different departments and teams are assigned to work on different aspects of capital

budgeting. Department managers prepare the budget for fixed assets in coming years, which is quite

helpful in capital budgeting. Besides, there are project managers who make the budget for a new project;

the cost accountants `count the cost' and assess the expenses to be incurred; the market researches

provide their input about the consumer psychology and sales potential. There may be as many

departments involved in capital budgeting, as there are present in an organization.

The biggest challenge in capital budgeting is to keep finding the valuable projects, i.e., projects that

may add to the value of the firm. You must be familiar with the basic objective of financial management

by now, which is to maximize shareholders' wealth. This is possible only by investing in the projects,

which have positive net present value, which in effect will increase the shareholders' wealth.

Most of the developed companies operate in an efficient market environment. We will discuss

efficient markets at length after studying the concept of risk akin to financial decisions. However, to

give you an idea, efficient markets can be described as highly competitive markets where good

business ideas are taken up immediately.

For instance, in Pakistan, about ten to fifteen years ago there was a video game craze. It was initially

a good business idea, as it required a very low-level investment, good profit margins, and short payback

periods. However, since the markets in Pakistan are quite efficient, the information about the business

spread quickly. More and more people started entering into the business and as a result, the profit

margins started shrinking and the lucrative business opportunity faded out in three or four years.

The same situation comes across the departmental heads of different companies. They may start a

new lucrative project, which may sound more than feasible at a given time. However, the competitors

get to know the new business opportunity, and because of market efficiency, those lucrative profits do

not remain lucrative anymore.

Financial Management MGT201

Techniques of capital budgeting:

Capital budgeting is a mathematical concept in the sense that we have to use different

quantitative investments criteria to evaluate whether an opportunity is worth investing in or not.

Some of these techniques of capital budgeting are as under

1. Pay back period

2. Return on investment (ROI)

3. Net Present Value (NPV)

4. Profitability Index (PI)

5. Internal Rate of Return (IRR)

We will assume that the interest rate, or the discount rate, or the required of return, which we use in

calculating the net present value is given, later on, when we will discuss the concept of risk, we would

see how the discount rate is calculated .

For now, let us talk about the pay back period.

Pay back period:

In this technique, we try to figure out how long it would take to recover the invested capital

through positive cash flows of the business.

Reverting back to the cafe example, an initial investment of Rs. 200,000 is required to start the

business; Rs 10,000 per month are expected to be earned for the first year, and Rs 20,000 would be

earned every month in the second year.

Now according to the aforementioned assumptions, in the first year, you earn Rs.10, 000 per

month, which make Rs. 120,000 for the year (twelve months). Since you had invested Rs. 200,000

initially of which Rs. 120,000 have been recovered in the first year, you are still Rs.80, 000 short of

recovering your initial investment. In the second year, you would be earning Rs. 20,000 per month, so

the remaining Rs. 80,000 can be recovered in the next four months. We can say that the initial invested

capital can be recovered in 16 months, or the payback period for this investment is 16 months. The

shorter the payback period of a project, the more an investor would be willing to invest his money in the

project.

While the payback period is a simple and straightforward method for analyzing a capital

budgeting proposal, it has certain limitations. First and the foremost problem is that it does not take into

account the concept of time value of money. The cash flows are considered regardless of the time in

which they are occurring. You must have noticed that we have not used any interest rate while making

calculation.

Now, let us talk about the next budgeting criteria called return on investment.

Return on Investments:

The concept of return on investment loosely defined, as there are a number of ratios that can be

used to analyze return on investment. However, in capital budgeting it implies the annual average cash

flow a business is making as a percentage of investment. In other words, it is an average percentage of

investment recovered in cash every year.

The formula for return on investment is as follows:

ROI= (∑CF/n)/IO

Dividing the average annual cash flow by the initial investment, we can calculate the return on

investment.

Example:

Taking the same example of a café, the initial investment of Rs.200,000, Rs 10,000 per month

profit in the 1st year in Rs 20,000 per month profit for the second year, we can easily calculate the ROI.

Financial Management MGT201

ROI= ((120,000+240,000)/2)/200,000= 0.90 = 90%

Where, Rs 120,000=cash flow for 1st year at Rs 10,000 per month

Rs 240,000=cash flow for the 2nd year at Rs 20,000 per month.

n=2 years

Return on Investment is also very easy to calculate, but like payback period, it does not take into

account the time value of money concept.

A high ROI ratio is considered better and 90% is a very good rate of return but before

deciding whether or not this project should be taken up, we should compare this project with the

alternative opportunities on hand. It is also important to take into consideration the prevailing rate of

inflation in the country so that the returns could be adjusted accordingly. However, we would talk about

the inflation rate and market interest rate in more detail later.

The next and the most important criteria for evaluating a capital budgeting proposal is net present value.

Net Present Value (NPV):

NPV is a mathematical tool which uses the discounting process, something that we have found

missing in the aforementioned capital budgeting techniques. Net Present Value is defined as the value

today of the Future Incremental After-tax Net Cash Flows less the initial investment.

The formula for calculating NPV is as follows:

NPV=-IO+∑CFt/ (1+i) t

Where,CFt=cash flows occurring in different time periods

-IO= Initial cash outflow

i=discount /interest rate

t=year in which the cash flow takes place

Initial cash outflow, being an outflow, is always expressed as a negative figure.

NPV is considered one of the most popular capital budgeting criteria. The disadvantage with the

NPV is that it is difficult to calculate since these calculations are based on too many estimates.

In order to calculate the NPV we need to forecast the future cash flows and sales; the discount factor is

also an estimate. If the NPV of a project is more than zero, it should be accepted. If two or more projects

under contemplation, then the one with the higher NPV, should be accepted. When a company invests in

projects with positive NPV, they raise the shareholders' wealth or company's value. This would also

increase the market value added and the economic value added for the firm.

Example:

Taking the same example of a café, an initial investment of Rs.200, 000, Rs 10,000 per month

profit in the 1st year in Rs 20,000 per month profit for the second year. However, for the calculation of

the NPV we would be requiring another important input--the discount rate. Assume the discount rate is

10 percent. Ten percent is what you at least expect to earn from the business. This is the rate of return,

which you can get by simply putting your money with a bank. If the business cannot yield more than 10

percent, then it is pointless to take unnecessary headache of setting up a business and running it, since

ten percent can be earned with a no-sweat-effort of placing the money with a bank.

Where,CFt=cash flows occurring in different time periods, i.e., Rs 120,000 in the first year and Rs

240,000 in the second year

-IO= Initial cash outflow = -200,000

i=discount /interest rate = 10 percent

t= 2 years

Putting in the values in the formula

Financial Management MGT201

NPV=-IO+∑CF/i

=-200,000+120,000/(1+0.10)+240,000(1+0.10)2

= - 200,000 +

109,091

198,347

=+Rs.107438

At the end of 2nd year, the NPV is +ve, you can also solve this example by monthly

compounding if you want to have a more precise answer.

The cash flows at the end of the first year and second year will have to be brought back to the present.

The present value of the cash flows occurring at the end of the first year can be calculated by dividing

the cash flows by 1 plus discount factor as under.

120000/(1+0.10) = 109,091

The cash flow occurring at the end of the second year can be calculated by dividing the cash

flow by one plus discount factor squared.

240,000/1+(0.10)2 = 198,347

NPV=-2000000+120000/(1+0.10)+240000/(1+0.10)2

=-200000+109091+198347

=+Rs.107438 at the end of second year NPVis +ve

In other words, according to your cash flow forecast and required return, two years of running this

business is worth Rs 107,438 in cash to you today. The following diagram can explain the point further.

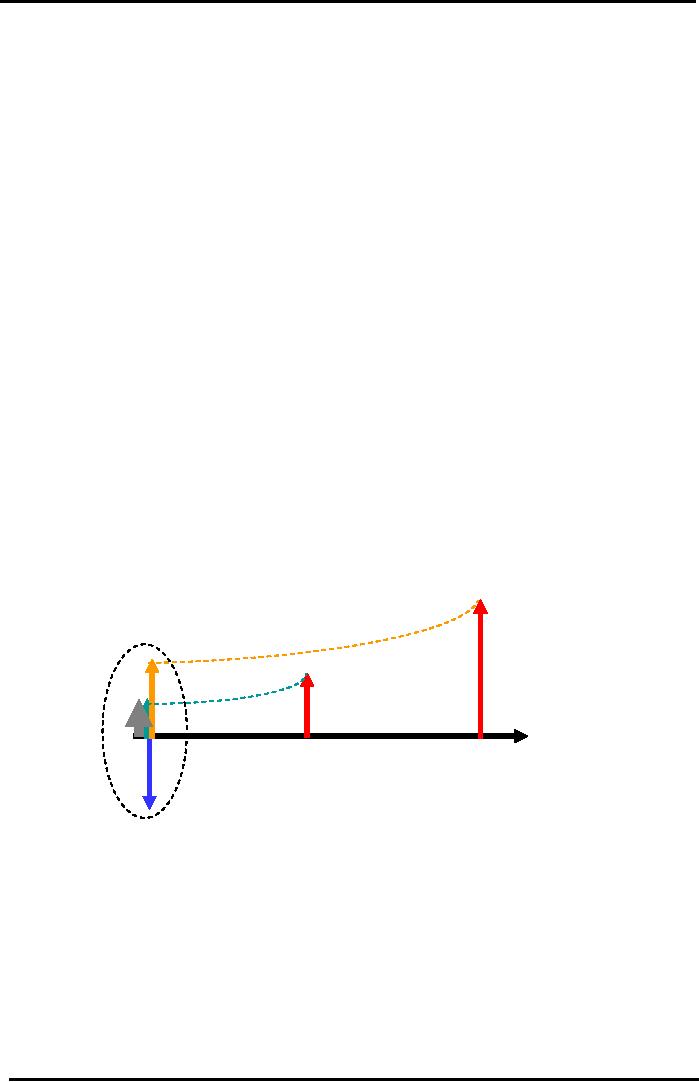
Investment Criteria

N.P.V (Café Example Cash Flow Diagram)

CF2 = Rs

240,000

Rs 198,347

CF1=Rs 120,000

Rs 109,091

NPV = Rs

107,438

i = 10%

Yr 0 (Today)

Yr 1

Yr 2

Io = Rs

200,000

The next criterion that we would talk about here is the profitability index, or the cost-benefit ratio.

Probability Index:

It is quite similar to the NPV in terms of concept and calculation. Profitability index

may be defined as the ratio of the present value of future cash flows to the initial investment.

The profitability index can be calculated using the following formula.

PI = [Σ CFt / (1+ i) t ]/ IO

Financial Management MGT201

Those projects with a profitability index ratio of more than one (PI >= 1.0) are considered

acceptable. Here it is important to mention that those projects, which are ranked as acceptable using the

NPV method, would also be acceptable on the profitability index criteria.

Example: The profitability index for the café example can be calculated as under.

PI = [120,000]/ (1+ 0.1) + [240,000 / (1+ 0.1)2]/200,000

= (109,091 + 198,347) / 200,000 = 1. 54

PI = 1.54 > 1.0

Therefore, the project is acceptable. Notice that we have taken into consideration the annualized

return. The same can be calculated using the monthly returns with a slight adjustment in the formula as

we have studied in the previous lectures. If there were two or more projects that need ranking, the one

with the highest profitability index would be acceptable.

Let us now talk about the fifth and the final capital budgeting criteria of our course, known as Internal

Rate of Return (IRR).

Internal Rate of Return (IRR):

IRR is a widely used and an important measure, which is more common in practice than the

NPV. IRR, unlike NPV that is expressed in dollar amounts, is always quoted in terms of percentage,

which makes it comparable to the other market interest rates or the inflation rate.

RR calculation involves the same equation that we have earlier used for the calculation of NPV.

The only difference is that while calculating IRR we would set the value of NPV equal to zero and then

solve the equation for the value if `i'. In other words, the value of `i', at which the net present value of

the project equals zero would be considered as the internal rate of return of the project.

his is important to remember that unlike NPV calculation, the value of IRR is constant in every

year for the life of the project. While working out the NPV, we can change the discount rate for every

single, but for IRR you would come up with a rate that is constant and fixed for every single year in the

life of the project. Another simplistic explanation of IRR can be that it is the break-even rate of return.

In other words, at this rate of return, we would be able to recover the initial investment in project's

lifetime.

RR is calculated by a trial and error method or iteration. Finding the value of an unknown

variable may involve solving of higher degree polynomial equations and the easiest way to go about it is

to use trial and error method.

n a trial-and-error method, we tryout a value of `i', and see if the equation comes to the value of

zero; if it does not, try another value, even if the second value does not bring the equation down to zero

and so on. The higher the IRR, the better it is considered, however, which value of the IRR can be

considered as acceptable is difficult to measure. We would discuss more details of it in the coming

lectures.

Another important distinction needs to clarification here is that the internal rate of return is

different from the discounting rate that we use in the calculation of the NPV. In the NPV formula, we

used the discount rate as the required rate of return that we expected the project to generate. In case of

IRR, we used the existing cash flows to find the forecasted return. These two different interpretations of

`i' should be kept in mind while calculating NPV and IRR.

We can calculate the IRR for the café project in the following manner. Using the same formula of NPV,

we can put the values in the formula

IRR Equation:

NPV= -IO +CF1/ (1+IRR) + CF2/ (1+IRR) 2

= 0= -200,000 + 120,000/ (1+0.1) + 240,000/ (1+0.1)2

Solving the equation assuming IRR to be 10 percent, we have obtained a figure of 107,483, which was

calculated as our NPV for the café project. However, in order to bring the NPV down to zero, we need

Financial Management MGT201

to apply a higher rate as an assumed IRR. If we assume IRR to be 50 percent the equation can be solved

as follows.

NPV= -IO +CF1/ (1+IRR) + CF2/ (1+IRR) 2

= 0= -200,000 + 120,000/(1+0.5) + 240,000/(1+0.5)2

The calculation gives us a figure of -13,333, which is lesser than zero. In order to bring the value equal

to zero we would use a rate lesser than 50 percent.

Trying out various IRR rates, we can finally reach a rate of 43.6 percent at which the value of

NPV would come down to -48 which is close to zero. If we try out IRR with more decimal places, we

can bring the value of NPV equal to zero. However, with approximation, 43.6 percent is the actual IRR

of the project.

Send you query to registrar.

More details about the IRR and the NPV would be discussed in the next chapter.

Table of Contents: