PRESENT VALUE AND DISCOUNTING:Interest Rates for Discounting Calculations

<< FINANCIAL FORECASTING AND FINANCIAL PLANNING:Planning Documents, Drawback of Percent of Sales Method

DISCOUNTING CASH FLOW ANALYSIS, ANNUITIES AND PERPETUITIES:Multiple Compounding >>

Financial Management MGT201

Lesson 06

PRESENT VALUE AND DISCOUNTING

Learning objectives:

After studying this lecture, you would be able to have a better understanding of the following.

· Present Value and Discounting

The Topics of this lecture are covered in the chapter 6 of our text book called Financial

Management theory and practice by Eugene F. Brigham & Louis C. Gapenski.

Objectives of present value:

The objective of calculating the present value is to translate the future cash flows in to present

terms. The basic principle is to compare apples with apples. For instance, if you have Rs.10 in your

pocket today and then you have may as many rupees ten years after, how can you compare the two. You

can do it only by comparing both amounts at the Present time.

We choose the present (today) as the most convenient point in time where we could compare all

the cash flows taking place at various points in time in future. We must compare everything at the

SAME point in time otherwise; we would be neglecting the Time Value of money concept.

For example, Rs 105 is more than Rs 100 BUT; Rs105 after 1 year may not necessarily be

more than Rs100 today! We first have to first bring all cash flows to the Present, or Discount them, and

then compare them. The concept of present value says that we can compare both the amounts in the date

of today we will bring back future cash flows to the present

Discounting:

"Discounting is defined as bringing the future cash flow to the present time".

Before answering which amount is greater in the aforementioned example, we need to have

some concept of interest rates or the cost of money. An interest rate can also be understood as an

opportunity cost.

One of the simple ways of estimating what opportunity cost or interest rate should be for our

discounting calculations, we can use interest rate given on the PLS accounts by the banks. For example,

if money is deposited in a bank and getting 10% per annum then it is interest or opportunity cost for you.

This interest on PLS account becomes minimum rate of return which any investment should be able to

generate. Therefore, the investment project should offer higher rate of return than the returns on the PLS

account.

Now let's see the answer of the question that Rs.105 will be more one year later or Rs.100 today,

and for this, we need interest or opportunity cost. It is important to understand why interest rate is called

opportunity cost? Because, opportunity cost essentially means the cost of taking up one option while

sacrificing the other. For instance, when you deposit your money in the bank and get interest, you are

sacrificing by

(1) Not consuming the money to buy something for yourself and

(2) Not investing your money elsewhere at a higher return than the bank interest.

Usually when an investment option is taken up, investors forgo the option of depositing the

money in a bank account and earn interest on that. The opportunity lost in this case is the opportunity

cost. Now the question is that what kind of interest rate should we use? There are many interest rates

quoted in the schedules of the bank but for discounting, the most commonly used rate is the nominal

interest rate, or APR.

Interest Rates for Discounting Calculations

· Nominal (or APR) Interest Rate = i nom

· It is usually published in newspapers .Annual Nominal Interest Rate is quoted for 1

year by Credit Card Companies and Leasing Companies because it understates the

actual (or Effective) interest you have to pay, these companies want to create an

impression that the interest charged by them is the minimum in the market.

· Periodic Interest Rate = i per

Periodic interest rate is used in FM for Discounting and Present Value (PV) calculations.

It is defined as

iper = ( i nominal Interest rate) / m

Where

m = no. of times compounding takes place in 1 year i.e.

If semi-annual compounding then m = 2

Financial Management MGT201

Effective Interest Rate = i eff

It is very useful to compare securities and investments with different life or

compounding cycles but not used for Discounting and PV.

i eff = [1 + ( i nom / m )]m 1

Where m = no. of times compounding takes place in 1 year, the compounding cycle. The shorter the

compounding cycle more frequently money compounded & faster the money grows.

Coming back to our earlier example where we were trying to figure out whether or not Rs 100 of

today are worth more than Rs 105 a year after, while the periodic interest rate is 10 percent per annum. The

interest rate used here would be the nominal interest rate, i.e., 10 percent. When we are going to solve for

the present value we are discounting from the future to the present

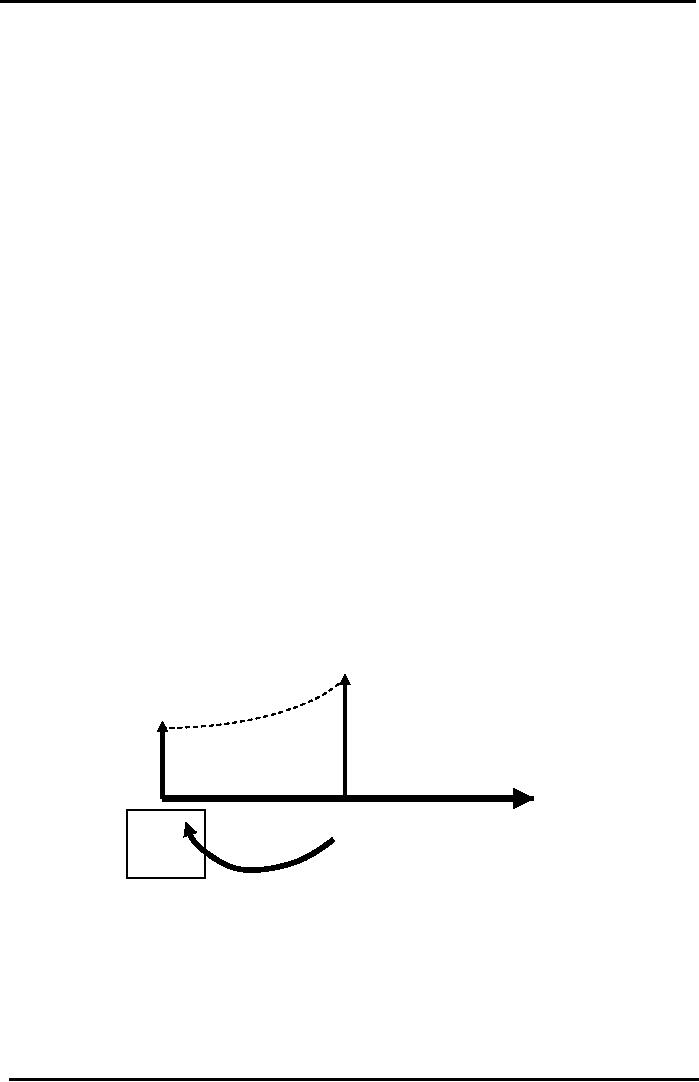
PV=FV/(1+i)n

Where, i=interest rate

N=no. of years if we plug in the values

PV=105/(1+0.10)1=Rs.95.45

Now we can see that if we discount Rs.105 from future to the present that is only the worth of

Rs.95.45 which is less than Rs.100. The amount offered in the future is seemingly more but when

converted to present value, the worth it has today, it come out to be less than Rs 100. Thus, it is clear

that Rs100 today worth more to Rs. 105 one year later. This conclusion is drawn on the assumption that

interest rate is 10%, but if we change the interest rate, the answer might be different.

With the help of the following diagram, we can observe the effect of discounting the cash flows.

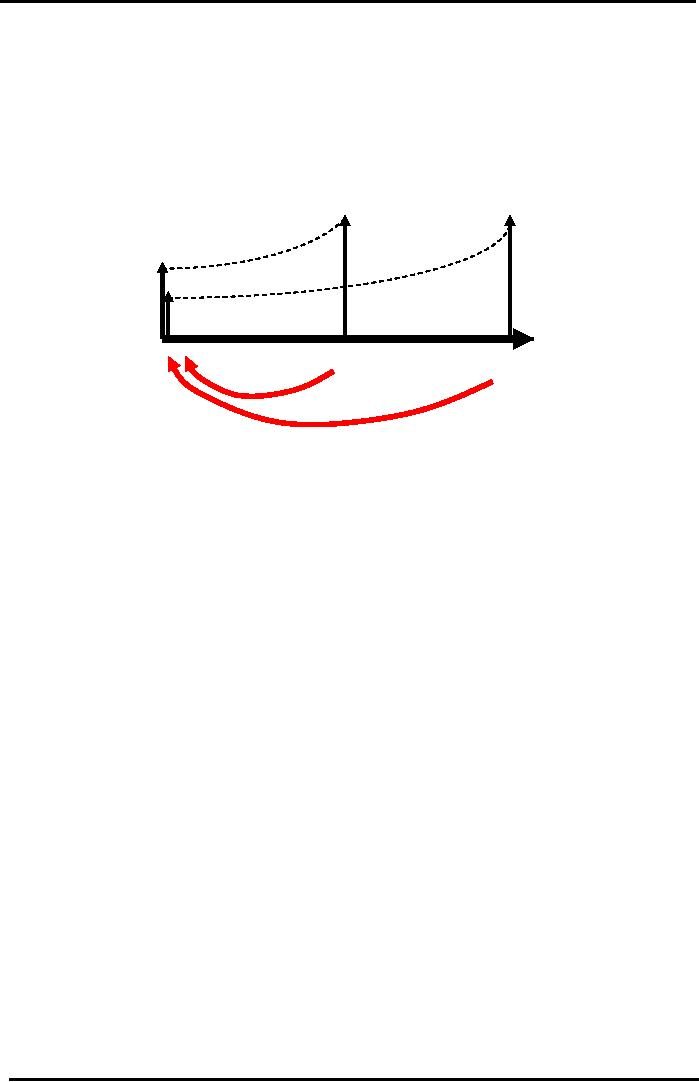
Now the point to understand is that if we discount back this money from 2 years back we would have

only approximate Rs.87 in other words if Rs 105 are to be received after two years, the present value of

would be even lesser

If you discount Rs 105 two years from now, you will have lesser amount than you have one

year from today. It is clear from the slide that as more future cash flow occurs distant in time, the more

its present value decreases.

Time & Arrow Diagram

1 Year Discounting of Future Value

Time & Arrow Diagrams are important in visualizing the concept of Discounting

FV = Rs. 105

PV = Rs 95.45

Interest: 10% pa

Time (Years)

Yr 1

Yr 0

Today

Present

Discounting

Financial Management MGT201

Time & Arrow Diagram

2 Year Discounting of Future Value

Notice how Discounting Rs 105 (FV2) from Year 2 gives a smaller Present

Value (PV2 = Rs 87) than Discounting Rs 105 from Year 1

FV1 = Rs. 105

FV2 = Rs. 105

PV1= Rs 95.45

PV2= Rs 87

Interest: 10% pa

Time (Years)

Yr 1

Yr 2

Yr 0

Today

Present

Discounting

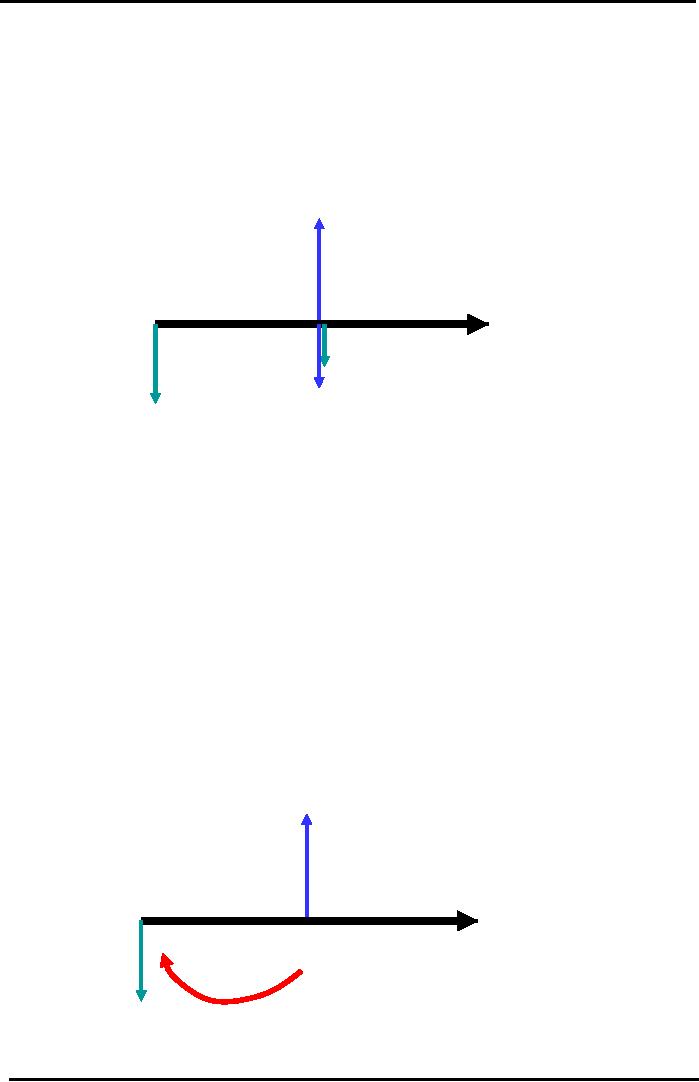
Cash inflows (Income, other income & cash profits) shown with upward pointing arrow

Cash outflows (expense) shown with downward pointing arrow

Discounting Cash Flows of a Business, Investment, or Project:

Let's talk about the discounting cash flow of the business as to how we would estimate the cash

flow business can generate? How can we calculate the market value and intrinsic value of a business or

working asset? There are two steps involved

1) Forecast future cash flows of any business, investment, or project by using percent of sales

method.

2) Discount the net cash flows back to the present time.

The two-step process here can be elaborated by the following hypothetical example.

Cafe Case Study:

Suppose you are thinking about starting a small café or canteen inside a university campus. You

make a simple feasibility report showing the estimated initial investment and the forecasted cash flows

for the first Year (based on expected cash receipts from sales and cash payments for expenses).

The Key Financial Data is as follows:

· Initial Investment = Rs 100,000

· Forecasted Cash Receipts (end Year 1) = Rs 200,000

· Forecasted Cash Payments (end Year 1) = Rs 50,000

· Forecasted Future Investment (end Year 1)=Rs30,000

· Periodic Interest Rate (Opportunity Cost) = 10% p.a.

First step is to represent the phenomenon through a diagram in the form of cash out flows.

Financial Management MGT201

Cash Flow Diagram

Café Example

Use Downward Pointing Arrows to show Cash Outflows (Cash

Payments or Investments). Use Upward Pointing Arrows to show

Cash Inflows (Cash Receipts)

Receipts = Rs. 200,000

Interest: 10% pa

Yr 0

Yr 1

Future Investment = Rs 30,000

Payments = Rs 50,000

Initial Investment = Rs 100,000

First of all, we can see the initial investment represented by the downward arrow. We have also

forecasted the sales one year from now that is Rs.200, 000. This is a cash inflow for the business and is

represented by an upward arrow; similarly, the expenses and investments (cash outflows) that we expect

in future, will be shown by the downward arrows. In the diagram there are three arrows, the upward one

is showing forecasted sales (cash inflow) and two arrows downward show expenses of Rs.50, 000 and

invest outlay of Rs.30, 000 respectively. Now the combined effect of the three arrows can be

represented by a single arrow. We can see that cash inflow of Rs.200,000 is having a +ve sign and

expenses of Rs.50,000 and investment out lay of Rs.30,000 have ve signs and finally, by deducting the

negative signed figures from the positive one we can arrive at the net effect of the cash inflows and

outflows, which is given as under

200,000-50,000-30,000 =Rs 120,000.

These different arrows can be added or subtracted because they are occurring at the same point

of time and Rs.120, 000 can be shown by one arrow sign. In order to calculate the present value of

Rs.120, 000, rate of interest as discount factor should be 10% per year.

Simplified Cash Flow Diagram

Café Example

Net Cash Receipts = CF1 = FV1

=200,00050,00030,000 = Rs 120,000

Interest: 10% pa

Yr 1

Yr 0

After combining all Cash Flows

for Year 2 into one Net Cash

Flow Figure (CF1), you are

ready to now Discount it to the

Initial Investment = Rs 100,000

Present...

Financial Management MGT201

Calculating the NPV

of the Café Business for 1st Year:

NPV = Net Present Value (taking Investment outflows into account)

NPV = -Initial Investment + Sum of Net Cash Flows from Each Future Year.

NPV = - Io +PV (CF1) + PV (CF2) + PV (CF3) + PV (CF4) + ...+ ∞

Note that PV (CF1) means the Present Value of Future Net Cash Flow (CF) taking place at the

end of Year 1. CF is like the FV in our interest formulas. Our compounding cycle is 1 year so the

Periodic Interest Rate is 10%.

Present Value of Net Cash Flow from Year 1 =

PV (CF1) = CF1 / (1+ i) n = 120,000 / (1+0.1)1 = Rs 109,000

The value of money has shrink from Rs.120, 000 to 109,000 as the concept of time value of the

money suggests and now we are in position to calculate the net present value of the money:

NPV = - Io + PV (CF1) = -100,000 + 109,000 = + Rs 9,000

The NPV of our Business after 1 Year is Positive Rs 9,000 which is a good sign. We will

discuss this topic in more detail in capital budgeting.

Table of Contents: