TIME VALUE OF MONEY:Discounting & Net Present Value (NPV), Interest Theory

<< ANALYSIS OF FINANCIAL STATEMENTS:Basic Financial Statements, Profit & Loss account or Income Statement

FINANCIAL FORECASTING AND FINANCIAL PLANNING:Planning Documents, Drawback of Percent of Sales Method >>

Financial Management MGT201

Lesson 04

TIME VALUE OF MONEY

Learning Objectives:

After going through this lecture, you would be able to have an understanding of the following

concepts.

· Main Concepts of FM.

· Time Value of Money

· Interest Theory and its determinants

· Yield curve theory and its dynamics

FM Concepts:

There are certain financial management concepts that should be kept in mind, while making an analysis

of a financial decision. The one-liners given here would help you in committing these concepts to your

memory.

· A rupee today is worth more than a rupee tomorrow.

Time Value of Money & Interest

· A safe rupee is worth more than a risky rupee.

- Risk and Return

· Don't compare apples to oranges

- Discounting & NPV

· Don't put all your eggs in one basket.

- Portfolio Diversification

· Get insurance because you will break some eggs.

- Hedging & Risk Management

Time Value of Money:

The first concept, time value of money, says that a rupee in your hand today is worth more than

the rupee that you are going to get tomorrow or the day after. This is because if you have a rupee in

hand, you can put it into a bank (invest it) and can earn interest (return) on it, and tomorrow you are

going to have more than rupee one, which of course, is more desirable than having just one rupee.

Risk and Return:

Investors want to earn maximum return on their investment; however, risk is a constraint to this

objective. Investors dislike risk-bearing, unless they are adequately compensated for that. Now the risk

and return concept states that a safe rupee in your hand is better than a risky rupee which is not in your

hand. This may imply that the investors would be willing to bear risk if they are offered more than a

rupee i.e., a certain premium for risk bearing. However, in the absence of this additional compensation,

a safe rupee is better than a risky rupee. The details about the concepts of risk and return would be

discussed in the middle of the course.

Discounting & Net Present Value (NPV):

The third concept is of discounting and net present value of money. This is a fundamental

mathematical concept and students need to practice it to perfection. Whether discounting for an asset or

a company, we have to see what cash flow would it generate during its future life and then we bring

back those future cash flow to the present, i.e., we discount the future cash flows to obtain their present

value. This exercise is done to make comparison of cash flows occurring in different time periods, i.e.,

comparing apples to apples, rather than oranges. This concept is relentlessly used throughout the course

in comparing different investment options in different time periods.

Portfolio Diversification:

The fourth concept is of portfolio diversification i.e. how to select different investment options

so as to reduce risk of losing the invested money. For instance if an investor has a million rupees and he

invests his total wealth in a single company's share, he would be exposed to greater risk. If the company

goes out of business or faces serious loss, the investor is likely to lose all his investment. However, if

that investor puts his total wealth into shares of ten different companies, the chances that all the ten

companies would face loss is comparatively lesser and hence the risk for the investor is diversified and

reduced. The rule of finance says do not put all your eggs in one basket, because if you drop the basket

accidentally, you are likely to lose all the eggs.

Financial Management MGT201

Hedging & Risk Management:

Finally, there is this fifth concept of hedging and risk management. Hedging is a strategy of

risk management that is employed by investors to reduce or minimize the chances of loss. Insurance is

said to be an effective tools used to manage risk. The concept of hedging and risk management says that

whether you put your eggs in one basket or in different baskets, chances are there that you will break

your eggs so it is better to get the eggs insured Insurance is the best way to avoid loss so that even if the

loss occurs you may get a claim on your damages.

Now, let's discuss the concept of interest in detail, first major & technical area in financial

management. Remember, that the basic objective in financial management is to maximize shareholders

wealth.

Interest Theory:

· Economic Theory:

Interest rate is an equilibrium price, expressed in percentage terms, at which demand and

supply of funds (or capital) meet, i.e., the rate at which the lenders are ready to lend and buyers are

ready to buy. But equilibrium price (Interest rate) varies from one market to another. For example,

the "price" of capital in the property market is different from the "price" of capital in the cotton

market. Markets have different interest rates guided by the supply & demand of funds in that market.

Although the interest rates in different markets may differ, however, all the markets in the country

and the interest rates prevailing there are interlinked.

Now, we come to the factors that determine the interest rates. It is important to understand the

factors that make up an interest rate in the present day business environment. In business when we talk

about the interest, we usually refer to nominal rate of interest which is determined with the help of

following factors.

Factors

i = iRF + g + DR + MR + LP + SR

i is the nominal interest rate generally quoted in papers. The "real" interest rate = i g

Here i = market interest rate

g = rate of inflation

DR = Default risk premium

MR = Maturity risk premium

LP = Liquidity preference

SR = Sovereign Risk

The explanation of these determinants of interest rates is given as under:

Risk Free Interest Rate (RF):

Factually speaking, there is no such thing as a risk-free rate of return because no investment can

be entirely risk-free. All the investments and securities include a certain amount of risk. A company

may go bankrupt or close down. However, if we talk about the relevant risk involved in different

securities, the government-issued securities are considered as risk-free, since the chances of default of a

government are minimal. These government issued securities provide a benchmark for the

determination of interest rates. Internationally the US T-Bills are considered as risk free rate of return.

In Pakistan, Government of Pakistan T-Bills can be used as a proxy for risk free rate of return, however,

since Pakistan faces some sovereign risk, the T-bills would not be considered entirely risk-free in the

true sense.

Inflation (g):

The expected average inflation over the life of the investment or security is usually inculcated in

the nominal interest rate by the issuer of security to cover the inflation risk. For instance, consider a

bond with a maturity of 5 years. If the inflation rate in Pakistan is 8 % and the bond is also offering 8%

percent interest rate, the investors would not be willing to invest in the bond since the gains from the

interest rate would be exactly offset by the inflation rate which is actually eroding the wealth of the

investor. To secure the investor against inflation the issuers, while quoting nominal interest rates, add

the rate of inflation to the real interest rate.

Default Risk Premium (DR):

Default risk refers to the risk that the company might go bankrupt or close down & bonds, or

shares issued by the company may collapse. Default Risk Premium is charged by the investor, as

compensation, against the risk that the company might goes bankrupt. Companies may also default on

Financial Management MGT201

interest payments, something not very unusual in the corporate world. In USA, rating agencies like

Moody's and S&P grade securities (debt and equity instruments) according to their financial health and

thus identify those companies which have a good ability to pay off their principal lending and interest

charges and those which might default on the payments. The rating from best to worst is: AAA, AA, A,

BBB, BB, B, CCC, CC, C. In Pakistan, Pakistan Credit Rating Agency (PACRA) and Vital Information

Services (VIS) are actively conducting analysis of corporate securities and grading them.

Maturity Risk Premium (MR):

The maturity risk premium is linked to the life of that security. For example, if you purchase the

long term Federal Investment Bonds issued by the government of Pakistan, you are assuming certain

risk, because changes in the rates of inflation or interest rates would depreciate the value of your

investment. These changes are more likely in the long term and less likely in the short term. Maturity

Risk Premium is linked to life of the investment. The longer the maturity period, the higher the maturity

risk premium.

Sovereign Risk Premium (SR):

Sovereign Risk refers to the risk of government default on debt because of political or economic

turmoil, war, prolonged budget and trade deficits. This risk is also linked to foreign exchange (F/x),

depreciation, and devaluation. Now-a-days the individuals as well as institutions are investing billions

of rupees globally. If a bank wants to invest in Pakistan, it will have to take view of Pakistan's political,

economic, and financial environment. If the bank sees some risk involved it would be willing to lend at

a higher interest rate. The interest rate would be high since the bank would add sovereign risk premium

to the interest rate. Here it may be clarified that Pakistan is not considered as risky as many other

countries of Africa and South America.

Liquidity Preference (LP):

Investor psychology is such that they prefer easily encashable securities. Moreover, they charge

the borrower for forgoing their liquidity. A higher liquidity preference would always push the interest

rates upwards.

Yield Curve Theory:

Term Structure and Yield Curve:

Interest rates for any security vary across time horizon. The supply & demand for funds vary

depending on how long the funds are required. Normally, short term interest rates are lower than long

term rates, or we can say that the interest rates depend on their term structure. Based on the maturity, the

securities can be classified into three categories, although, these classes have been loosely defined.

Short Term: Short term means for the period of one year or less.

Medium Term: For the period of any where between one year to five years.

Long Term: Any where between 15 years to 20 years some people say that medium term is

from 5 year to ten years and long term from 10 years to 20 years and plus.

Nominal or upward sloping yield curve:

The supply & demand of funds or capital varies depending upon how long funds are required. For

example, today the supply and demand for short term money might be different from supply and

demand of the long term money. In another words, the number of borrowers to take loan for one week

may be different from the borrowers of loan for one year. Short term interest may be different than the

long term interest; normally, short term interest rates are lower than long term than interest rates

because investors think that inflation is going to increase. This phenomenon results in nominal or

upward sloping yield curve.

Abnormal or downward sloping yield curve:

Sometimes, the reverse is true. This is known as the Abnormal (or Downward Sloping) Yield

Curve. It is the case where the short term raters are higher than long term interest tares. You can also

have a mixed or Humped Back Curve.

N o rm a l

A bno rm a l

Yr 1

Yr 3

Yr 5

Y r 10

Yr 20

Financial Management MGT201

Now, we go into the reason why the curves have either upward slope or downward slope. Following are

some of the factors that determine the slope of the yield curves.

Expectations Theory:

Investors normally expect inflation (and interest) to rise with time thereby giving rise to a

normal shaped yield curve.

Liquidity Preference Theory:

Investors prefer easily encashable securities with short maturities. The only problem is that

short term securities are easy to encash but at maturity there is no guarantee that you can renew it .so,

you can find a security today which will give you 25 %or 30% per annum they are not always

renewable hence unpredictable.

Market Segmentation:

The demand/supply for Short Term securities is different from that of Long Term securities.

This can easily give rise to an Abnormal Yield Curve.

Now let's talk about the practical types of interest there three kinds of interest we will talk about

1-simple interest

2-discrete compound interest

3-continuous compound interest

1. Simple Interest (or Straight Line):

Simple interest incurs only on the principal. While calculating simple interest we keep the

interest and principal separately, i.e., the interest incurred in one year is not added to the principal while

calculating interest of the next period. Simple interest can be calculated using the following formula.

F V = PV + (PV x i x n)

Example: Assume that you have Rs 100 today and you want to invest the amount with a bank for five

years. The bank is offering an interest rate of 7 percent. We can obtain the simple interest on the

investment using the aforementioned formula

F V = PV + (PV x i x n)

Here FV is the simple interest accrued for the term of the investment

PV is the amount invested, i.e., Rs 100 in our example

i stands for the interest rate offered by the bank, i.e., 7 % = 0.07

n is the term of the investment, which is assumed to be 5 years

Putting these values in the formula, we get

FV = 100 + (100 x 0.07 x 5)

FV = 100 + (7 x 5)

FV = 100 + (35)

FV = Rs 135

Here Rs 135 is the future value of investment after five years and Rs 35 is the interest accrued during

five years on the initial investment of Rs 100.

2. Discrete Compound Interest:

Discrete compound interest is the most commonly used tool in Financial Management

Discounting and NPV calculations. Unlike simple interest, compound interest takes into account the

principal as well as interest accrued for a term, while calculating interest incurred during the next term.,

i.e., interest incurred for one year would be added to the principal to calculate the interest for the next

period. However, this compounding of interest takes place in a discrete manner, i.e., the compounding

takes place yearly, semi-annually, quarterly, or monthly. For computing the annual compounding, we

use the following formula

Annual (yearly) compounding:

F V = PV x (1 + i) n

However, a slight modification in the formula is need if the compounding takes place monthly.

Such a compounding would be calculated using the following formula.

F V = PV x (1 + (i / m) m x n

Here `m' refers to the compounding intervals during the term of the investment. In order to

calculate monthly compounding, the value of `m' would be 12; however, for quarterly compounding

calculation m would be equal to 4

Financial Management MGT201

Example:

Assume that the investor in our previous example is offered a compound return (interest) on his same

investment, at the same interest rate and term. The future value of the investment is given as under

F V = PV x (1 + i) n

FV = 100 x (1+0.07)5

FV = 100 x (1.07)5

FV = 100 x (1.40255)

FV = 140.255

Here the interest accrued on the five year investment is more than what we found out in simple interest.

However if the compounding is done every month the future value of investment would be

F V = PV x (1 + (i / m)) m x n

FV = 100 x (1 + (0.07/12)) 12 x 5

FV = 100 x (1 + 0.005833)) 60

FV = 100 x (1.005833) 60

FV = 100 x 1.4176

FV = 141.76

With more frequent compounding, the wealth of the investor increases to a greater degree.

3. Continuous (or Exponential) Compound Interest:

The other type of compound interest is exponential compound interest. In this compound interest an

infinite number of times per year at intervals of microseconds.

F V (Continuous compounding) = PV x e i x n

Here e is a constant the derived value of which is 2.718

Example:

Assume that the same investor has now the opportunity of investing at continuous compounding with

the same term and interest rate. His future wealth after five years is given as under

F V = PV x e i x n

FV = 100 x 2.718(0.07x5)

FV = 100 x 1.419

FV = 141.9

We can see that the wealth of the investor is the highest, when he decided to invest in a scheme which

offers continuous compounding.

The difference between simple and compound interest can increase manifold if the term of the

investment is increased. As we see in the following example

Example:

Suppose you deposit Rs 10 in a bank today. The bank offers you 10% per annum (or per year)

interest. How much money will you have in the bank after 15 years?

If the bank is offering simple interest:

F V = PV + (PV x i x n) = 10+ (10x0.10x15) = Rs. 25

If the bank is offering discrete compounding:

F V = PV x (1+ i) n = 10 x (1+0.10)15 = Rs. 42 approx.

Banks do not offer continuous compounding but if they did:

F V = PV x e ixn = 10 x (2.718) 0.10x15 = Rs. 45 approx

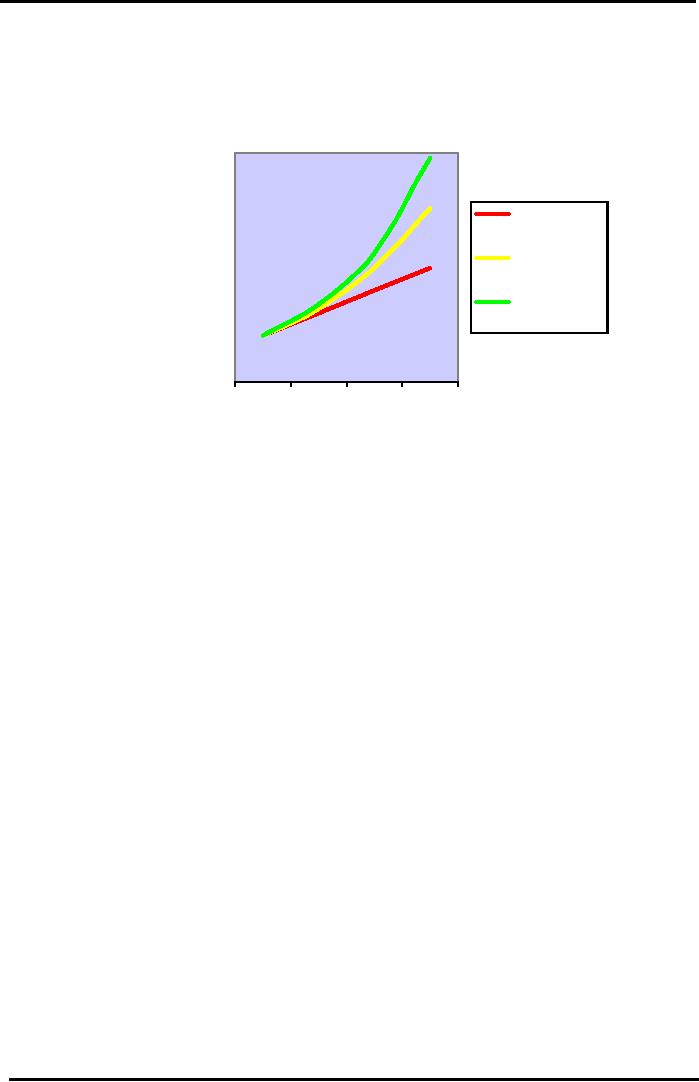
Financial Management MGT201

Graphical View of Compounding

The miracle of compounding you earn interest on

interest & principal!

Simple

Amount

Discre te

(Rupees)

Compound

Continuous

Compound

Yr 1

Yr 5

Yr 10

Yr 15

Note: After 15 years, Continuous Compounding gives you almost two times more money

than Simple Interest. Compound Interest gives you about one-and-a-half times as much!

Table of Contents: