RISK AND PORTFOLIO THEORY, CAPM, CRITICISM OF CAPM AND APPLICATION OF RISK THEORY:Think Out of the Box

<< SML GRAPH AND CAPITAL ASSET PRICING MODEL:NPV Calculations & Capital Budgeting

INTRODUCTION TO DEBT, EFFICIENT MARKETS AND COST OF CAPITAL:Real Assets Markets, Debt vs. Equity >>

Financial Management MGT201

Lesson 27

RISK AND PORTFOLIO THEORY, CAPM, CRITICISM OF CAPM AND APPLICATION OF

RISK THEORY

Learning Objectives:

After going through this lecture, you would be able to have an understanding of the following topics

· Risk, Portfolio Theory, CAPM

· Criticisms of CAPM

· Applications of Risk Theory

Today we will review some concepts about risk portfolio theory and capital asset pricing model.

There is saying in English that fortune favours the brave.

Summary of Single Stock (Stand Alone) Risk & Return:

The first thing that we studied was how to calculate the expected rate of return or risk for a

single stock that is what we call stand alone investment. Uncertainty comes along as we are not what is

price of the stock will be at any time in future? Because of this uncertainty there are possible outcomes

of such investment and we attach probability or their likely hood and we calculate a weighted average in

order to come up with expected rate of return on the investment.

Expected Return Formula (Weighted Average of Many Possible Future Outcomes for Returns of that

one Stock)

<r>=

∑ (p i x r i) (where p = probability of future outcome and r is the rate

of return from that outcome

So, if there are three possible outcomes attached with it the formula will be

<r>=PArA+PBrB+PCrC

Where, p=possible outcomes. There is a probablity distribution of such outcomes and this

distribution is the measure of the spread or range of possible values or uncertainty. if we a look at the

graph of the normal distribution curve then the width of the curve is the measure of the risk or

uncertainty. We measure risk mostly by standard deviation.

Stand Alone Total Risk Formula (Standard Deviation or Spread of Distribution of Possible Future

Returns)

Sigma = σ = (

∑ ( r i - < r i > )2 p i )) 0. 5 = (Var) 0. 5

This formula which represents the total risk of a single stock can be used for the portfolio of

stocks.

Portfolio Risk & Return:

We then spoke about the collection of many stocks. Why do sensible people invest in many

stocks the logic is very simple that do not put all your eggs in one basket. The experimental studies have

shown that if some one has 40 different stocks or investments which are not correlated to each other

then half of the risk can be eliminated. What kind of risk has been eliminated? This is the company's

specific risk that has been eliminated because of company's random events in the life of the company.

So, the diversification or investment in increasing number of stocks reduces the overall or total risk and

even if you diversified in 7 uncorrelated stocks it is possible to reduce the large portion of the

company's own risk .once you have known the return of the individual stock you have known about the

portfolio stock in collection of investments. Expected rate of return for 3 stocks on a portfolio is:

Expected Portfolio Return Formula (Weighted Average of Returns of Stocks in the Portfolio)

rP * = rA xA + rB xB + rCxC + (for a 3-Stock Portfolio)

Portfolio Risk Formula

σp =

XA 2σ

+ XB2 σ

+ 2 (XA XB σ Aσ

)

(2 Stocks)

If 2 stocks move in the same direction together then correlation coefficient is 1.0. if the exactly

move in the opposite direction then the correlation coefficient -1.0 and if there is no relation between the

movement of the stocks then the correlation coefficient is zero.

If More than 2 Stocks, then use RISK MATRIX

116

Financial Management MGT201

3-Stock Portfolio Risk Formula

3 x 3 Matrix Approach

Stock A

Stock B

Stock C

XA2

Stock

XA XB

XA XC

XB2

Stock

XB XA

XB XC

XC2

Stock

XC XA

XC XB

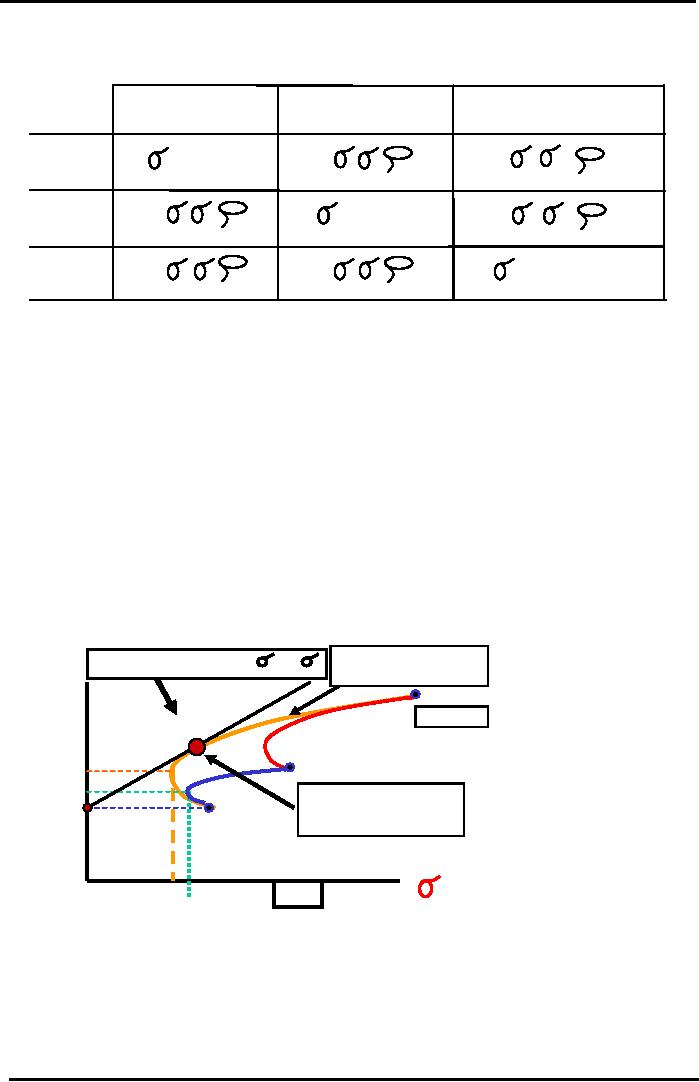
The portfolio theory told us there is direct relationship between risk and the return and as risk of the

investment goes up then return also increase .it is mentioned that if correlation co-efficient is less then

or equal to zero (ve) then the risk and return relationship between 2 stocks exhibits shaped curve what

it tells us is that it is possible that when we added the 3rd stock then it not only increase the overall return

but it also reduce the total risk of the portfolio and that is ideal . We found the parachute curve for the

portfolio which consist of more then 2 stocks and this large hook is the efficient frontier which

represents the most efficient combination of the different stocks in that portfolio. By adding stock C we

came up with parachute curve and we also came up with curve that envelops both of these curves which

is known as the efficient frontier. So, let's see how can we derive CML or the capital market line and

the equation of CML from this efficient market curve .portfolio return is with y- axis and risk along

with x-axis we see the line from risk free rate of return which is assumed to be 10% and is represented

by rRF .

Picking the Most Efficient Portfolio

Capital Market Line (CML) & T-Bill Portfolio

P Efficient Frontier

rP* = rRF + [ (rM - rRF) /

rP*

for 3-Stock Portfolio

Portfo

30%

Capital Market Line

Stock C

lio

"The Parachute"

Retur

Stock A

20%

Optimal Portfolio Mix

(50%A, 30% B, 20%C) if

10%=

Stock B

rRF

Risk Free T-Bill ROR = 10%

40%

20%

2.5%

Portfolio with Negative

Risk

3.4%

or Zero Correlation

Coefficient

Parachute Graph and Efficient Frontier (Hook Shaped Curve) shows ALL possible Risk-Return

Combinations for ALL combinations of stocks in the Portfolio whether efficient or not.

CML Straight Line Equation (T-Bill Portfolio and Optimal Portfolio Mix on Efficient Frontier Curve)

connects rRF (Risk-free or T-Bill return) to the Tangent Point on the Efficient Frontier Curve.

117

Financial Management MGT201

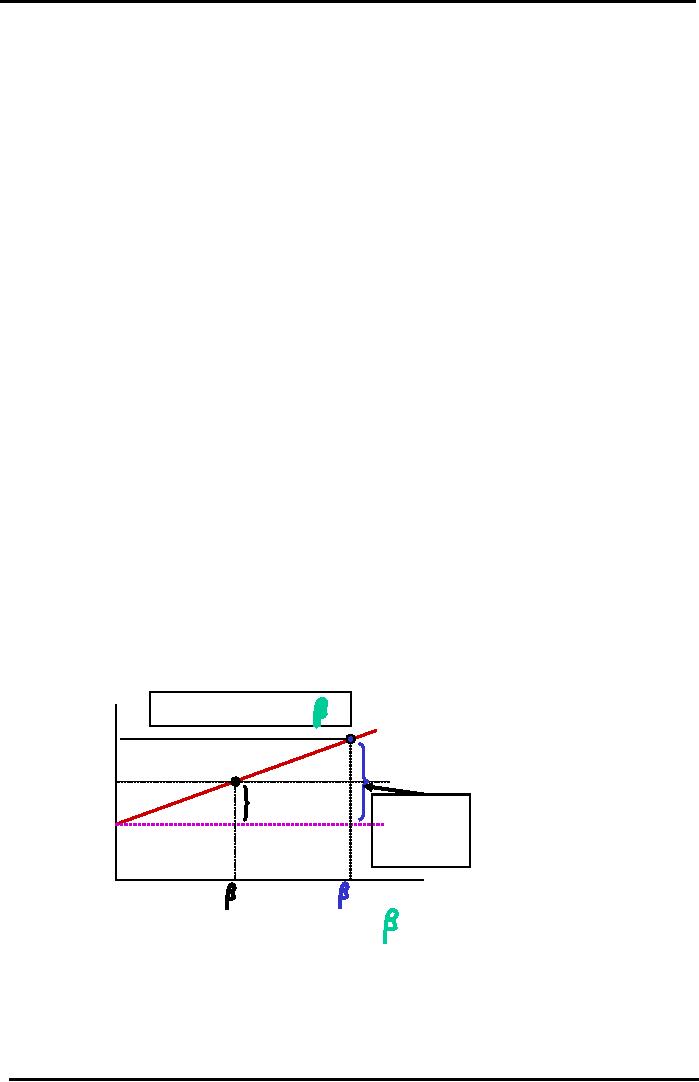
It represents all Risk-Return Combinations for Efficient Portfolios in the Capital Market. We

assume easy access to risk-free T-Bill Portfolio. Portfolio Risk measured using Standard Deviation.

There is an optimal point of the line where

Stock A=50%

Stock B=30%

Stock C=20%

Summary of Beta:

Market Risk and Beta Coefficient (CAPM)

Single Stock Beta (=Slope of Best Fit Regression Line which passes through data points)

= Percent Change in Stock ROR / Percent Change in Market Index ROR

Portfolio Beta Risk Formula (Weighted Average Formula)

Stock Beta Formula in terms of Stock Standard Deviation & Covariance

= σ A σ M ρ AM / σ 2 M

= σ A ρ AM / σ M = market risk

Security Market Line (SML)

SML (Security Market Line) - Cornerstone of CAPM:

It represents all Risk-Return Combinations for ALL Efficient Stocks in the Capital Market. Stock

risk measured using Beta. Market Price of a Stock is determined by Required Return on Stock which

depends on Market Risk (not Total Risk).You can not expect to receive extra return (or compensation)

for taking on Company-Specific Risk which Rational Investors have eliminated! Efficient Market

Prices are based on Market Risk Only and NOT Total Risk.

The Efficient Market will only offer you a Return (and a Share Price) which is the bare minimum acceptable

to rational diversified Investors.

Required ROR vs Expected ROR

SML Linear Equation & Graphical Interpretation

Security Market Line (SML)

ALL Efficient Stocks in Efficient Markets

Required

rA = rRF + (rM - rRF )

Return (r*)

rA= 30%

Security Marketrket Risk

Line

rM= 20%

Premium for

Risky Stock A's

Avg Stock = 10%

rRF= 10%

Total Risk

Premium =

30-10 = 20%

A =+ 2.0

M =+ 1.0

Beta Risk (

)

Criticisms of CAPM & Alternatives:

Weakness in SML:

· Not All Investors are rich or well-informed enough to hold Fully Diversified Portfolios

therefore Market Risk (and Betas) is NOT the only relevant factor in estimating Required

Return and Stock Prices. Other Efficient Market Assumptions.

118

Financial Management MGT201

Taxes and Brokerage Costs that affect Investor's analysis and estimation of Returns have

been ignored

Weakness in CML:

Not All Investors are influential enough to be able to Borrow at the T-Bill Rate. Generally the

Borrowing Rate is higher than the Lending Rate.

Fama & French:

CAPM ignores 2 important determinants of Higher Required ROR (1) smaller firms and (2)

Low Market-to-Book Ratio.

Arbitrage Pricing Model:

Accounts for several factors that affect risk i.e. Tax, inflation, oil price,...

Financial Management Applications of Risk-Return Theory (CAPM):

· Practical Real Asset Investment Decisions and Capital Budgeting

The most important NPV (and PV) Equations uses REQUIRED ROR (and NOT

Expected ROR)

· Actual Share Pricing and Investment in Securities

Gordon's Formula for Share Pricing uses PV of Dividends which uses REQUIRED

ROR

Risk & Return - Must Consider both:

In this course we have studied the following concepts of financial management.

· First Part of this Course - Valuation or Calculating NPV and PV which are measures of

Return. We ignored Risk and origins of Required ROR.

· Second Part of this Course Application of PV Concept to Valuation or Pricing of Bonds

(Debt) and Shares (Equity). Again we ignored Risk and origins of Required ROR.

· Part 3 of the Course Introduced Risk and how it determines the Required Return used in

NPV and Share Price Formulas.

· In Perfect Markets, Value depends on Required Return which depends on Market Risk (and

not Total Risk).

· BUT, in Real Markets which are Imperfect and Inefficient, Total Risk is important. It can

be calculated using the Sigma (Standard Deviation) Formulas, probabilities, and Expected

Return.

· Total Risk and Expected Return must BOTH be considered in Comparing Investments.

· Market Risk and Required Return are Related to one another

Common Life Applications of Risk and Return Theory:

Concepts of Risk & Return Theory have Wide Practical Applications that require a Creative Mind.

Expected Value or Expected ROR or Expected Payoff

Total Risk or Standard Deviation (based on Spread or Range of Breadth of Possible ROR

outcomes) = Unique + Market Risk

Systematic (or Market or No diversifiable) Risk (= Beta A x Sigma M). Individual Risk relative

to Market or Industry.

· Think Out of the Box:

Social Cost-Benefit Analysis of Power Plant:

Environmental and Village Relocation Risk, Uncertain Savings

Court Case Payoff: Claims & Penalties

Uncertain likelihood of success and Opponent, Uncertain Payoff

Likelihood of War: Capability & Intent (Game Theory)

Magnitude of Capability vs Uncertainty of Intent

119

Table of Contents: