STOCK BETA, PORTFOLIO BETA AND INTRODUCTION TO SECURITY MARKET LINE:MARKET, Calculating Portfolio Beta

<< EFFICIENT PORTFOLIOS, MARKET RISK AND CAPITAL MARKET LINE (CML):Market Risk & Portfolio Theory

STOCK BETAS &RISK, SML& RETURN AND STOCK PRICES IN EFFICIENT MARKS:Interpretation of Result >>

Financial Management MGT201

Lesson 24

STOCK BETA, PORTFOLIO BETA AND INTRODUCTION TO SECURITY MARKET LINE

(SML)

Learning Objectives:

After going through this lecture, you would be able to have an understanding of the following

topics.

· Stock Beta

· Portfolio Beta

· Introduction to SML (CAPM)

First, we would recap some of the concepts which we have studied in the previous lectures.

It is mentioned in the efficient capital markets the investors would take on extra risk only if they are

compensated in the form of extra return. The market only compensates the investor to the extent that he

will receive extra return for extra market risk he takes on by investing in a new stock. However, the

market will not pay the investor any extra return for taking on unnecessary risk in the form of

company's own risk. Therefore, it is best for investors to act rationally and to maintain diversified

portfolios of many stocks and in this manner they can eliminate the company's own risk and they can

make investments in stocks at a lower required rate of return. Market portion of risk can be represented

through the `Beta' coefficient and it is the corner stone for Capital Asset Pricing Model (CAMP).

Beta:

It is a tendency of a Stock to move with the Market (or Portfolio of all Stocks in the Stock

Market).it is the building block of CAPM.

Total Risk = Diversifiable Risk + Market Risk

Total Stock Return = Dividend Yield + Capital Gain Yield

Stock Risk Vs Stock Beta:

Stock Risk:

It is a statistical spread of possible returns (or Volatility) for that Stock

Stock Beta:

It is a statistical spread of possible returns (or Volatility) for that Stock relative to the

market spread i.e. spread (or Volatility) of the fully diversified market portfolio or index.

Beta Coefficients of Individual Stocks are published in "Beta Books" by Stock Brokerages & Rating

Agencies

MARKET:

It is the overall Stock Market. For Example, Karachi Stock Exchange. KSE 100 Index represents Value

of "Portfolio" of Highest Volume Stocks but NOT ALL stocks. Therefore it is not fully perfect

diversified portfolio.

Market carries Risk. It moves up and down because of macroeconomic factors (inflation,

general interest rates) and political changes. Therefore the market has some expected rate of return

which changes with time because of this there is possibility of different outcomes. There are no fully

diversified portfolios in reality. The CAMP based on the promise market Beta to be Equal to + 1.0. We

can then look at the different beta and compare them with the markets.

Meaning of Beta for Share ABC in Karachi Stock Exchange (KSE):

· If Share A's Beta = +2.0 then that Share is Twice as risky (or volatile) as the KSE Market i.e. If

the KSE 100 Index moved up 10% in 1 year, then based on historical data, the Price of Share B

would move up 20% in 1 year.

· If Share B's Beta = +1.0 then that Share is Exactly as risky (or volatile) as the KSE Market

· If Share C's Beta = +0.5 then that Share is only Half as risky (or volatile) as the KSE Market

· If you could find a Share D with Beta = -1.0 then that share would be exactly as volatile as the

KSE Market BUT in the opposite way i.e. If the KSE 100 Index moved UP 10% then the price

of the Share D would move DOWN by 10%!

· The Beta of most Stocks ranges between + 0.5 and + 1.5

· The Average Beta for All Stocks = Beta of Market = + 1.0 Always.

Our approach for calculating the beta will be straight forward. Let us assume that we analyze he

movements in KSE index for period of three years and we also analyze the price movements for the

Stock A in KSE for the same period of time of three years. We look at the change in the price of the

103

Financial Management MGT201

stock and compare it to the change in price in the market for each one of the three years separately.

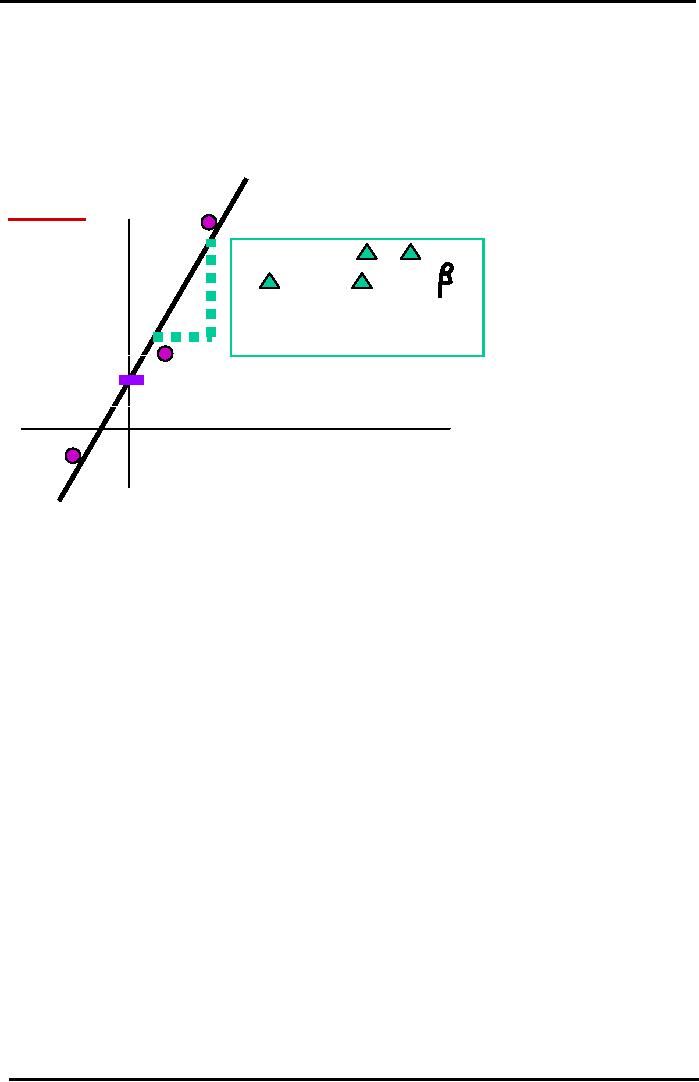
And then we plot those points on a graph where the expected return on the stock on the y- axis and

the expected return on the market on x-axis. We are using the price of the stock and value of the

index as representative measures for the expected return.

Calculating Stock Beta Graphically

Linear Regression or Least Squares Fit through Experimental

Data Collected for 3 Years

Year 2

Expected

Return on

Slope = Beta = Y / X =

Stock A

(Historical) %

% rA* / % rM =

A=Risk Relative to Market =

rA* - rRF

(rA* - rRF) / (rM* - rRF)

Y-Intercept =

Year 1

Alpha =

Company Specific Risk

rM* - rRF

Expected Return on KSE 100 Market Index

Year 3

(Historical) %

If you look at the graph, we have plotted three years of data for the changes in the Karachi stock

exchange 100 market index and the expected return on the stock A. The expected return on A is on y-

axis and it is represented by rA* and the expected return on stock in KSE 100 market index is

represented by rM* the `*' represented the expected part of the rate of return. In both cases the expected

returns have been benched marked against the risk free rate of return. That is because we pick the risk

free rate of return as the starting point for the changes in the expected return. Three points are shown on

the graph on for each year in the analysis and after plotting these points on the graph we can then do

what is known as the linear regression of straight line which best fit through points. You have studied

this concept in the course of "Statistics and Probability" .The slope of the line represents the beta

coefficient.

Slope = Beta = Δ Y / Δ X = % Δ rA* / % Δ rM*

= A =Risk Relative to Market = (rA* - rRF) / (rM* - rRF)

Beta represents the risk of stock relative to the return of the market and in terms of risk free rate

of return we can define the Beta as the expected rate of return for stock A minus risk free rate of return

divided by the expected rate of return for the market minus risk free rate of return. We use historical

data of expected rate of return and we graphed it against changes in the overall market index

Calculating Portfolio Beta (CAPM):

There are two ways of calculating portfolio beta

· Analyze Historical Data for Portfolio Returns and Market Index Returns like in the case of

Stock Beta, plot Least Squares Fit Line, and compute Portfolio Line Slope or Beta directly.

· Use the Published Data for Individual Stock Betas from the "Beta Book"

Portfolio beta can be calculated as the sample weighted average of the stock beta's in that portfolio.

Portfolio Beta = β P =

XA β A+

XB β B + XC β C +.....

In the formula

βA represents the Beta (or Market Risk) of Stock A.

XA represents the Weight of Stock (fractional value of investment in A to total

portfolio value).The simple formula for calculating the portfolio beta is as follows.

Portfolio Beta (or Market Risk) Formula is a Simple Weighted Average unlike the portfolio risk formula

104

Financial Management MGT201

σP =

XA2 σ A

+XB2 σ

+ 2 (XA XB σ Aσ B ρ AB )

Example:

· Complete 2-Stock Investment Portfolio Data:

Value Exp Return (r*) Tot Risk

Beta

Stock A

Rs.30 20%

20%

2.0

Stock B

Rs.70 10%

0.5

Total Value =Rs 100

Correlation Coefficient

= + 0.6

Portfolio Mean Expected Return = 13% = rP*

Portfolio Risk (Total) = 8.57% = P (relative to rP*)

Now we can calculate the portfolio beta which is measure of the market portion of portfolio risk.

Portfolio Beta = XaBa + XbBb = (30/100) (2.0) + (70/100) (0.5) = 0.6 + 0.35

= +0.95 = β P (relative to Market Risk or Volatility)

It means that the Portfolio of A & B is slightly less risky than the totally diversified KSE 100

Market Portfolio whose Beta = +1.0

Effect of New Stock Investment on Portfolio:

Now we will see the case that what will happen to portfolio beta if we add another stock to it.

Suppose, you add a 3rd Investment Stock C, to your Old 2-Stock Portfolio.

Value

Exp Return (r*)

Beta

Stock A

Rs.30

20%

2.0

Stock B

Rs.30

10%

0.5

Stock C

Rs.40

30%

1.5

Total Value

Rs.100

3-Stock Portfolio Beta = β P = XaBa + XbBb + XcBc

= (30/100) (2.0) + (30/100) (0.5) + (40/100) (1.5)

= 0.6+0.15+0.6 = 1.35

The effect of adding a stock with a Beta higher than the Portfolio's is that it increases the Portfolio's

Beta or Risk. In this case we also increase the beta by adding new stock but the expected rate of return

also increases for the portfolio. So, the increase rate of return would compensate the increase in risk.

Required Rate of Return (CAPM)

Required ROR vs. Expected ROR

Expected ROR (r*):

The Most Likely (or Mean) ROR expected in the future. It is calculated using Weighted

Average Formula and Probabilities (what we have been calculating so far).It is basically the weighted

average or mean of the expected return of the individual investments in the portfolio.

Required ROR (r):

It is the minimum return that investors require from the stock to invest in it. It

varies from individual to individual. It is based on

1) Perceived Risk relative to the Market and Psychological Risk Profile of each Investor and

2) His personal Opportunity Cost of Capital preference.

We have mentioned earlier that ROR or opportunity cost varies from person to person because

every individual have a different preference for risk taking. Some people have tendency to be gamblers

whereas other people put their money at national saving schemes.

However, the ROR can be linked

to the Beta risk because based on the portfolio theory and CAPM where we mentioned there is direct

relationship between risk and return.

Average Required ROR for all rational investors in an Efficient Market can be estimated using

the CAPM Theory: Beta and Risk Free Rate of Return.

Total Rate of Return (ROR) for Single Stock = Dividend Yield + Capital Gain. GORDON'S

FORMULA FOR COMMON STOCK PRICING OR VALUATION USES REQUIRED RETURN r =

DIV/Po + g. In Efficient Markets, Price of Stocks is based on Market Risk (or Beta).

We can formulate the required rate of return in terms of Beta risk so how can we use beta coefficient to

calculate the required rate of return for the average investor in the market. The answer to it is the

105

Financial Management MGT201

Security Market line SML. It is the part of CAPM and it is the most critical part of CAPM. SML is

straight line relationship that contains all possible combinations of efficient stocks in the market. If the

combination of risk and return for any stock does not lie on the SML then that stock is not efficiently

priced. In other words, it means that for most of the investors in the market there ROR for investment in

stock A is directly proportional to Beta risk for that stock A. You will recall that we are not unfamiliar

with straight line relationship between risk and return when we are talking about the portfolio when we

calculate the portfolio risk with a +ve correlation coefficient we came up with a continuously increasing

relationship between portfolio risk and return. So that model is similar to SML.

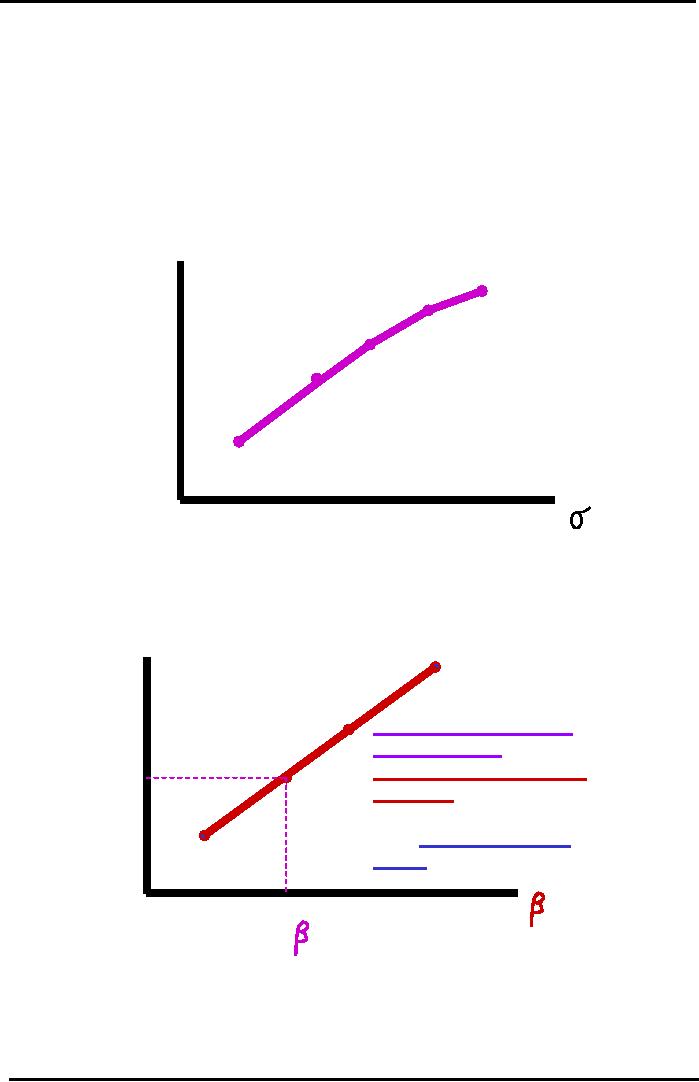
Risk vs. Return Graph (Risk Theory)

2-Stock Portfolio with Positive Correlation Coefficient

rP*

Non-Efficient Portfolio

25%

Portfolio

23%

Return

20%

15%

10%

12% 15% 20%

Risk

Risk vs. Return Graph (SML- CAPM)

EFFICIENT MARKETS WITH FULLY DIVERSIFIED

PORTFOLIOS AND EFFICIENTLY PRICED STOCKS

25%

Required

Return 20%

FULLY DIVERSIFIED

PORTFOLIOS AND

EFFICIENTLY PRICED

15%

STOCKS IN EFFICIENT

MARKETS WILL LIE ON

10%

THE STRAIGHT SML

LINE.

1.0

0.5

1.5

2.0

Market Risk

= M

Security Market Line (SML):

It is a Straight Line Model for Beta Risk and Required Return. It is Similar to the Relationship for

the 2-Stock Portfolio with ρ >0. Beta Risk is Directly Proportional to Required Return. The

Investors require an extra Return which exactly compensates them for the extra Risk of the Stock

relative to the Market.

106

Financial Management MGT201

SML Linear Equation for the Required Return of any Stock A:

rA = rRF + (rM - rRF ) β A .

In the above equation

rA = Return that Investors Require from Investment in Stock A.

rRF = Risk Free Rate of Return (ie. T-Bill ROR).

rM = Return that Investors Require from Investment in an Average Stock (or the Market Portfolio of All

Stocks where β M = + 1.0 always). β A = Beta for Stock A. (rM - rRF )

β A = Risk Premium or Additional Return in Excess of Risk Free ROR to compensate the Investor for

the additional Risk.

107

Table of Contents: