BONDS VALUATION AND YIELD ON BONDS:Present Value formula for the bond

<< BONDSâ€™ VALUATION:Long Bond - Risk Theory, Bond Portfolio Theory, Interest Rate Tradeoff

INTRODUCTION TO STOCKS AND STOCK VALUATION:Share Concept, Finite Investment >>

Financial Management MGT201

Lesson 15

BONDS VALUATION AND YIELD ON BONDS

Learning Objectives:

After going through this lecture, you would be able to have an understanding of the following topics

· Bond Valuation

· Yield of Bonds

In previous lecture, we studied are bonds are long term debt instruments. Like Stock bonds are also

direct claim securities which means that the value of these bonds is determined by the future cash flows

that bond holders will receive. These cash flows are of two basic types

1. Cash inflow: in the form of coupon receipt with regular interval over the life of the bond

2. Other cash flow is the par value of the bond which you will receive at the maturity date of the

bond.

Present Value formula for the bond:

PV= ∑ CFt / (1+rD)t =CF1/(1+rD)+CFn/(1+rD)2 +..+CFn/ (1+rD) n +PAR/ (1+rD) n

t =1

NPV = Intrinsic Value of Bond or Fair Price (in rupees) paid to invest in the bond. It is the Expected or

Theoretical Value and needs to be compared to the Market Price. It is different from the Par (or Face)

Value which is printed on the Bond paper.

rD = Bondholder's (or Investor's) Required Rate of Return for investing in Bond (Debt). DIFFERENT

from the Coupon Rate and the Market / Macroeconomic Interest Rate!

There are basically 2 kinds of Cash Flows:

(1) Annuity from Fixed Regular Coupon Receipts (CF= Coupon Rate x Par Value) and

(2) Single Cash Flow from Par Value (or Initial Investment) Returned to the Investor on maturity.

In this equation

rD: It represents the required rate of return. It is the return which is required by the investor based on

his opportunity cost. In case of Pakistan, the investor required a higher return on bond then the rate of

markup offered by the PLS account in bank. It is different from the Coupon Rate and the Market /

Macroeconomic Interest Rate.

Coupon or CF:

It is a fixed rate and it is equal to

(CF= Coupon Rate x Par Value)

Par value of the bond is fixed but the market price varies with the change in the supply and demand,

perception of investor for that bond.

Example:

Defense Savings Certificates: Suppose that you invest in a Defense Savings Certificate whose Par

Value is Rs 100,000. The Bond Issuer is the Government of Pakistan. The Certificate has small

detachable coupons. You (as the Bondholder or Investor) can present one Coupon at the end of

every month and receive Rs 1,000 cash. After 1 year, you will be repaid your Principal Investment

(or Par Value) of Rs 100,000. Assume your Required Return (rD) is 10% pa. What is the Present

Value of this Investment to you?

In the previous lectures, we have solved simple version of similar example we solved a problem

similar to this where we had to calculate the NPV of the Defense Savings Certificate with 1 Annual

Coupon payment after 1 year.

We arrived at the following approximate answer:

NPV =

-Io +

CF1 / (1+ i) +

CFI1 / (1+ i)

= -100,000 + 12,000/(1+0.10) + 100,000/(1+0.10)

= -100,000 +

10,909

90,909

= 1,818

(NOTE: PV = NPV + Io = 10,909 + 90,909 = 101,818)

But this is not the correct exact answer to our present example because it ignores monthly compounding.

Accurate Solution - Monthly Compounding:

The Accurate solution to the Savings Certificate Example with Monthly Coupons requires us to

use a monthly cash flow diagram and do monthly discounting. There is an Annuity Stream of 12

Financial Management MGT201

Coupons (Cash Inflows) of Rs 1,000 each at the end of every month. There is a final Cash Inflow worth

the Par Value of Rs 100,000 at the end of the 12th month.

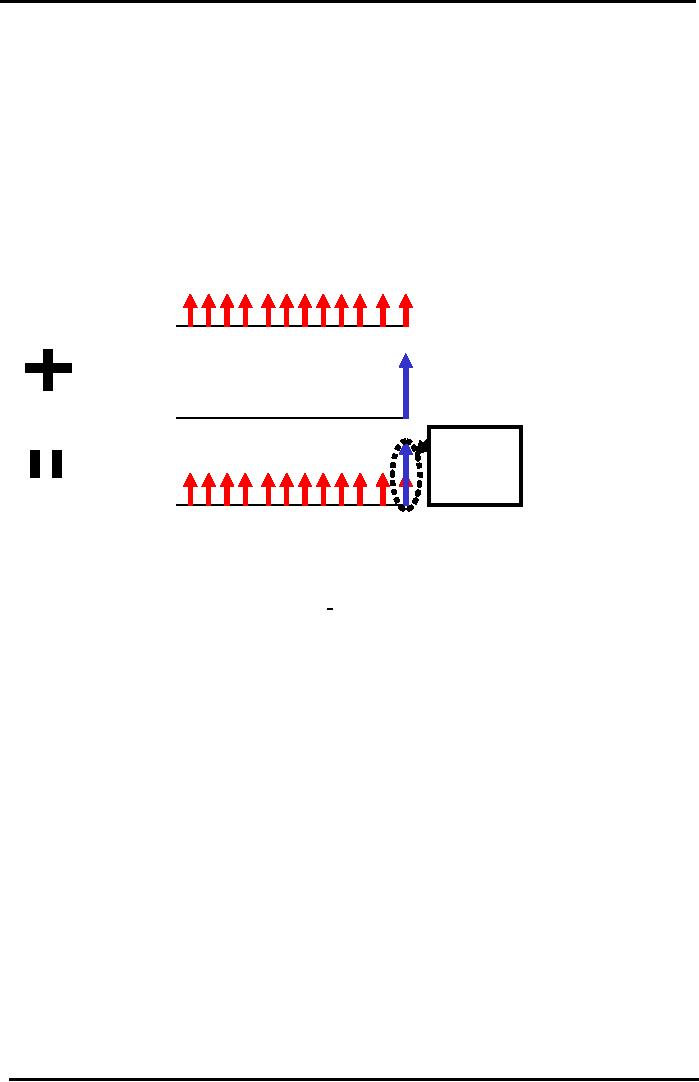
The Cash Flow Diagram for Bonds is a Combination of 2 Flows: (1) an Annuity Stream (of

Coupon Receipts) every month for 12 months and (2) One Par Receipt at the end of the 12th month.

You can draw their individual Cash Flow Diagrams and then add them up later. You can compute their

PV's separately and then add them up later.

Cash flows from coupons represents by the upward pointing arrows which represents cash

inflows.

In combine diagram, at the end of the year there are two upward pointing arrows. One for

coupon rate and the other is for the payment of par value of bond.

Bond Cash Flow Diagram

Savings Certificate Example

Rs 1,000

Coupon Annuity:

(Monthly)

Time (Months)

01 2

Rs 100,000

Par Receipt :

(at Maturity)

2 Cash Flow

Arrows at

SAME point in

time can be

Combined

added.

Time (Months)

Cash Flow

01 2

Diagram

Calculate the PV of Coupons from the FV Formula for Annuities (with multiple compounding

within 1 year):

FV = CCF (1 + rD/m )nxm - 1/rD/m

Use Monthly Basis for this example. n = 1 year m = 12 months

CCF = Constant Cash Flow = Rs 1,000 = Monthly Coupon

rD = Annual Nominal Required Rate of Return for investment in Bond (Debt) = 10% pa.

Periodic Monthly Required Rate of Return is rD/m = 10/12 = 0.833 % = 0.00833 p.m.

m = 12 months

Coupon Annuity Cash Flow Receipts

FV = 1,000 x [(1.00833)12- 1] /0.00833 = +Rs 12,566 (at the end of 1 year)

PV (Coupons Annuity) = FV / (1 + rD/m) nxm

= 12,566 / (1.00833)12 = +Rs 11,374

Final Par Value Cash Flow Receipt

FV = 100,000 (at the end of 1 year)

PV (Par) = 100,000 / (1.00833)12 = +Rs 90,522

PV = PV (Coupons Annuity) + PV (Par) = 11,374 + 90,522

= + Rs 101,896 (Final Answer)

So this Certificate is worth Rs 101,896 to you today. It is worth more than the Market Price (Rs

100,000). So it is a good investment.

NOTE: Our answer is slightly higher than what we got when we used Annual compounding (Rs

101,818).when we consider multiple compounding the present value of the bond increases. Its NPV is

greater than zero so on the basis of our capital budgeting techniques you should invest in that project.

Now, we consider over all rate of return on a bond. We have studied expected price of the bond. These

two are complimentary. When bonds trader talk about he overall return on a particular bond they

referred to yield to maturity.

Financial Management MGT201

Bond Yield to Maturity (YTM):

We can calculate the Value of our Investment in Bonds. But how can we compute its Rate of

Return? Both are important whether you are talking about Investment in Real Assets or Securities.

The most common way to compare the Overall Rate of Return of different Bonds is to compare

their YTM's.

In capital budgeting, you can calculate IRR using the NPV equation. Similarly, you can calculate it

by setting the PV Equation for Bond Valuation equal to the Present Market Price and solve for "rD".

Use Trial and Error or Iteration. The value of "rD" that gives PV = Market Price is the YTM for that

Bond.

= Bond Market Price =

CFt / (1+rD)t

CF1/ (1+rD) +CF2/(1+rD)2+...+CFn/(1+rD)n+ PAR/(1+rD)n

All variables are known (ie. CF, PAR, and n) EXCEPT rD .Set PV equal to the Actual Present Market

Price of Bond and solve for rD

YTM = rD

Bond YTM Example:

Term Finance Certificate (TFC): The TFC (a kind of Bond) of Company ABC is traded in the

Karachi Stock Exchange for Rs 900. The Par Value of the TFC is Rs 1,000. The Coupon Rate is fixed

at 15% pa. Coupons are paid annually. The TFC will Mature after exactly 2 Years (it is a 5 Year Bond

issued 3 Years ago). What is the Overall Expected Rate of Return (ie. YTM) offered by this TFC?

Market Price (Rs 900) is LESS than its Par Value (Rs 1,000). This Bond is selling at a Discount. Why?

Possibly Interest Rate Risk. Market Interest Rate rises above TFC's Fixed Coupon Rate so Market Price

of the TFC falls below Par. Note: when Market Interest Rates rise, Required Rate of Return (rD) for

Investors rises. But, Coupon Rate fixed by Bond Issuer at time of issue.

The Expected (or Promised) Rate of Return for Investors is the Yield to Maturity (or YTM).

Compute the Overall Return (or YTM) for the TFC using the Old IRR-like Approach:

PV = Market Price = Rs 900

Par Value =Rs 1,000. Receive this after 2 Years (remaining life)

Annual Coupons =Coupon Rate x Par =15%x1, 000 = Rs 150

rD = Minimum Return Required by the Investors investing in

The Bond Market = YTM.

This is unknown in the equation.

PV = 900 = 150 / (1+ rD) + 150 / (1+rD)2 + 1,000 / (1+rD)2

900 = 150 / (1+ rD) + 1,150 / (1+rD)2 . Use Trial & Error

rD > 15%:

Try rD = 20%: PV = 924 (close)

Try rD = 21%: PV = 909 (closer)

YTM = 21.7%: (Gives PV=Rs 900)

YTM: YTM is the expected rate of return for which the bond holder holds the bond until maturity but if

the bond holder before maturity is called by the issuer or if the holder of the bond decides to sell the

bond before maturity then your answer will change .all the calculation will remain the same only par

value is replaced as

PV=∑ CFt / (1+rD)t =CF1/(1+rD)+CF2/(1+rD)2+...+CFn/(1+rD)n+CALL/(1+rD)n

t=1

Where CALL = PAR Value + 1 Year's Worth of Coupon Receipts

YTM =Total or Overall Yield = Interest Yield + Capital Gains Yield

TFC Example Total Yield = YTM = +21.7%

Interest Yield or Current Yield = Coupon / Market Price

TFC Example Interest Yield = Rs 150 / Rs 900 = +16.7% pa

Capital Gains Yield = YTM - Interest Yield

TFC Example Capital Gains Yield = 21.7% - 16.7% = +5 %

n = Maturity or Life of Bond (in years)

FV=CCF[(1+rD/m)n*m-1]/rD/m

N=1 year ,m= no. of intervals in a year =12

CCF=constant cash flow =1000=monthly copoun .we can plug the values in this formula to know what

the future value of annuity is going to be ?take a look at the copoun annuity :

Financial Management MGT201

FV=1000[(1-0.00833)12-1]/0.0083=+12566 at the end of one year what is the present value of this

copoun annuity

PV=FV/(1+rD/m )n*m

=12566(1.00833)12

=+11374

This is the present value of cash flow from coupon. Now we need to calculate the present value of face

value at maturity suppose face value =100,000 then

PV(PAR)=100,000/(1.00833)12

=+90,522

Now, we combine the present value of coupon interest and present value of par both

i.e.=11374+90522= Rs.101896.

When we compare the answer with annual cash flows where coupon was not compounded

monthly .it is grater because monthly compounding increase future cash flows as well as the present

value .2nd thing is that this NPV is grater than the initial investment which is Rs.100,000so, we should

under take this project because the NPV is grater .now, the next area is the rate of return so, the

important thing in this regard is yield to maturity .this is abbreviated as YTM.it is easy to understand

because we have discussed IRR in capital budgeting .where we set NPV=0 and calculated for r .here

market price is the YTM of the bond and then solving for the variable rD=required rate of return .so,

let's try to understand YTM using a very simple example ,the example that we will pick out is that of

term finance certificate or TFC which is by the stock exchanges of Pakistan for Rs.900.let's assume that

its par value is Rs.1000fixed or coupon interest rate is 15 p.a. and it is paid annually ,total life of the

TFC is 5 years 3 years have already passed and it will mature 2 years from now what will be over all

expected rate of return .So, let's see the equation if we compute the over all yield here we can equate

PV=market value is the YTM for the bond the PV=900 which is market price PAR=1000.

Annual coupon rate =coupon rate *par =15/100*1000=150

rD=minimum return required by the investor in the bond market =YTM it is unknown ?

PV= 900=150/(1+rD )+150/ (1+rD)2+1000/(1+rD)2

we also know that the value of rD should be more than 15% you will try different values for example if

you try 20% you will come up with PV=24 (close),try rD=21.7% PV=900 so,

YTM =21.7% =900

Therefore 21.7% is the yield to maturity for this TFC because rD=YTM .YTM is the expected

rate of return for which the bond holder holds the bond until maturity but if the bond holder before

maturity is called by the issuer or if the holder of the bond decides to sell the bond before maturity then

your answer will change .all the calculation will remain the same only par value is replaced as call value

so,

Call=par value +I, year copoun receipts

Another thing to keep in mind is that YTM has two components first is

YTM=interest yield on bond +capital gain yield on bond from his example

YTM= 21.7% so,let's calculate the interest yield

INTEREST YIELD =annual copoun interest /market price

=150/900 =16.7% so,

CAPITAL YIELD =YTM INTEREST YIELD

=21.7%-16.7%=5%

Table of Contents: