 |
Measuring Risk |
| << TYPES & SOURCES OF RISK |
| ANALYZING PORTFOLIO RISK >> |
Investment
Analysis & Portfolio Management
(FIN630)
VU
Lesson
# 33
PORTFOLIO
THEORY
Measuring
Risk:
Risk
is often associated with the
dispersion in the likely
outcomes. Dispersion refers
to
variability.
Risk is assumed to arise out
of variability', which is consistent
with our
definition
of risk as the chance that
the actual outcome of an
investment will differ from
the
expected
outcome. If an asset's return
has no variability, in effect it
has no risk. Thus, a
one-
year
treasury bill purchased to yield 10
percent and held to maturity
will, in fact, yield
(a
nominal)
10 percent No other outcome is possible,
barring default by the U.S.
government,
which
is not considered a reasonable
possibility.
Consider
an investor analyzing a series of
returns (TRs) for the
major types of
financial
asset
over some period of years.
Knowing the mean of .this
series is not enough;
the
investor
also needs to know something
about the variability in the
returns. Relative to
the
other
assets, common stocks show,
the largest variability
(dispersion) in returns, with
small
common
stocks showing f ten greater variability.
Corporate bonds have a much
smaller
variability
and therefore a more compact
distribution of returns. Of course,
Treasury bills
are
the least risky. The
"dispersion of annual returns
for bills is compact.
Standard
Deviation:
The
risk of distributions' can be measured
with an absolute measure of
dispersion, or
variability.
The most commonly used
measure of dispersion over
some period of years is
the
standard
deviation, which measures
the deviation of each
observation from the
arithmetic
mean of
the observations and is a reliable
measure of variability, because
all the information
in a sample is
used.
The
standard deviation is a measure of the
total risk of an asset or a
portfolio. It captures the
total
variability in the assets or
portfolios return whatever
the source of that variability.
The
standard
deviation can be calculated from
the variance, which is
calculated as:
n
2
σ
=
∑
(X -
X)
i=1
n-1
Where;
σ2 = the variance of
a set of values
X =
each value in the
set
X =
the mean of the
observations
n =
the number of returns in the
sample
σ2 = (σ2) 1 / 2 = standard
deviation
Knowing
the returns from the sample,
we can calculate the standard deviation
quite easily.
Dealing
with Uncertainty:
Realized
returns are important for
several reasons. For
example, investors need to
know
how
their portfolios have
performed. Realized returns, also can be
particularly important in
helping
investors to form expectations
about future returns,
because investors must
concern
189

Investment
Analysis & Portfolio Management
(FIN630)
VU
themselves
with their best estimate of
return over the next
year, or six months, or
whatever.
How
do we go about estimating returns,
which is what investors must
actually do in
managing
their portfolios?
The
total return measure, TR, is
applicable whether one is measuring
realized returns; or
estimating,
future (expected) returns.
Because it includes everything
the investor can
expect
to
receive over any specified
future period, the TR is
useful in conceptualizing the
estimated
returns
from securities.
Similarly,
the variance, or its square
root, the standard deviation, is an
accepted measure of
variability
for both realized returns
and expected returns. We will calculate
both the
variance
and the standard deviation below and
use them interchangeably as
the situation
dictates.
Sometimes it is preferable to use one and sometimes
the other.
Using
Probability Distributions:
The
return an investor will earn from
investing is not known; it
must be estimated. Future
return
is an expected return and may or may
not actually be-realized. An
investor may
expect
the TR on a particular security to be
0.10 for the coming
year, but in truth this
is
only
a "point estimate." Risk, or
the chance that some
unfavorable event will occur,
is
involved
when investment decisions are
made. Investors are often
overly optimistic
about
expected
returns.
Probability
Distributions:
To deal
with the uncertainty of
returns, investors need to
think explicitly about a: security's
distribution
of probable TRs. ln other
words, investors need to
keep in mind that,
although
they
may expect a security to
return 10 percent, for
example, this is only a
one-point
estimate of
the entire range of possibilities.
Given that investors must
deal with the
uncertain
future, a number of possible returns
can, and will, occur.
In
the case of a Treasury bond
paying fixed rate of interest,
the interest payment will
be
made
with l00-percent certainty
barring a financial collapse of
the economy. The
probability
of
occurrence is 1.0; because no
other outcome is possible.
With
the possibility of two or
more outcomes, which is the
norm for common stocks,
each
possible
likely outcome must be considered and a
probability of its occurrence
assessed.
The
probability for a particular
outcome is simply the chance
that the specified
outcome
will
occur. The result of
considering these outcomes and
their probabilities together is
a
probability
distribution consisting of the
specification of the likely
outcomes that may
occur
and
the probabilities associated
with these likely
outcomes.
Probabilities
represent the likelihood of various
outcomes and are typically
expressed as a
decimal.
The sum of the probabilities
of all possible outcomes must be
1.0, because they
must
completely describe all the
(perceived) likely
occurrences.
How
are these probabilities and
associated outcomes obtained? In the
final analysis,
investing
for some future period
involves uncertainty, and therefore
subjective estimates.
Although
past occurrences (frequencies) may be
relied on heavily to estimate
the
probabilities
the past must be modified
for any changes expected in
the future.
190

Investment
Analysis & Portfolio Management
(FIN630)
VU
Calculating
Expected Return for a
Security:
To
describe the single most
likely outcome from a
particular probability distribution, it
is
necessary
to calculate its expected value.
The expected value is the
weighted average
of'all
possible
return outcomes, where each
outcome is weighted by its
respective probability of
occurrence.
Since investors are interested in
returns, we will-call this expected
value the
expected rate of
return, or simply expected-return, and
for any security, it is
calculated as;
m
E
(R) = ∑
Ri pri
i=1
Where;
E (R)
= the expected return on a
security'
Ri
= the
ith possible return
pri = the probability of
the ith return Ri
m =
the number of possible
returns
Calculating
Risk for a
Security:
Investors
must be able to quantify and measure
risk. To calculate the total
risk associated
with
the expected return, the
variance or standard deviation is used,
the variance and,
its
square
root, standard deviation, are
measures of the spread or
dispersion in the
probability
distribution;
that is, they measure
the dispersion of a random
variable around its mean.
The
larger
this dispersion, the larger
the variance or standard
deviation.
To
calculate the variance or standard
deviation from the
probability distribution,
first
calculate
the expected return of the
distribution. Essentially, the
same procedure used
to
measure
risk, but now the
probabilities associated with
the outcomes must be
included,
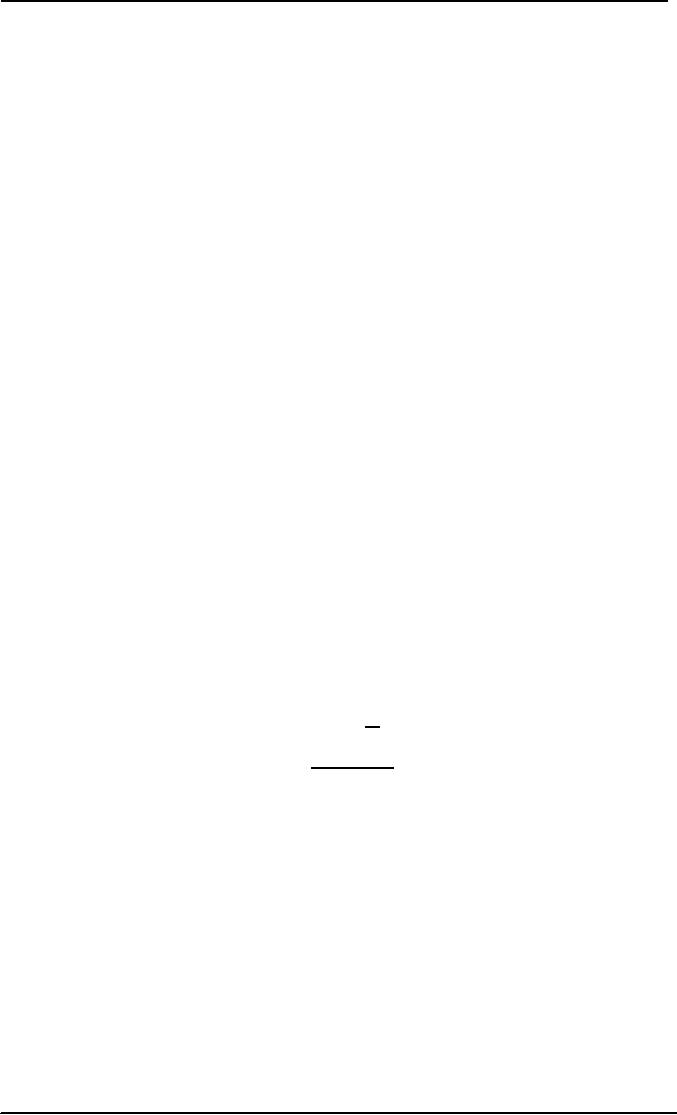
m
The
variance of returns = σ2
= ∑
-
[Ri E (R)]2pri
i=1
And
The
standard deviation of returns =
σ
=
(σ2)1/2
Portfolio
Expected Return:
The
expected return on any portfolio is
easily calculated as a weighted
average of the
individual
securities expected returns. The
percentages of a portfolio's total
value that are
invested
in each portfolio asset are
referred to as portfolio weights,
which will denote by w.
The
combined portfolio weights
are assumed to sum to 100
percent of, total
investable
funds,
or 1.0, indicating that all
portfolio funds are
invested. That is,
n
w1 + w2 + ... + wn = ∑
wi = 1.0
i=1
191

Investment
Analysis & Portfolio Management
(FIN630)
VU
Portfolio
Risk:
The
remaining computation in investment
analysis is that of the risk
of the portfolio. Risk
is
measured
by the variance (or standard
deviation) of the portfolio's
return, exactly as in
the
case
of each individual security.
Typically, portfolio risk is
stated in terms of standard
deviation
which is simply the square
root of the variance.
It is at
this point that the
basis of modern portfolio
theory emerges, which can be
stated as
follows:
Although the expected return of a
portfolio is a weighted average of
its expected
returns,
portfolio risk (as measured
by the variance or standard deviation) is
not a weighted
average
of the risk of the
individual securities in the
portfolio. Symbolically,
n
E
(Rp) = ∑
wi E (Ri)
i=1
But
n
σ2p
≠ ∑
wi σ2i
i=1
Precisely,
investors can reduce the risk of a
portfolio beyond what it
would be if risk
were,
in
fact, simply a weighted
average of the individual
securities' risk. In order to
see how this
risk
reduction can be accomplished, we must
analyze portfolio risk in
detail.
192
Table of Contents:
- INTRODUCTION OF INVESTMENT
- THE ROLE OF THE CAPITAL MARKETS
- THE NASDAQ STOCK MARKET
- Blue Chip Stocks, Income Stock, Cyclical Stocks, Defensive Stocks
- MARKET MECHANICS
- FUNDAMENTAL STOCK ANALYSIS
- BEYOND FUNDAMENTAL ANALYSIS
- What is Technical Analysis
- Indicators with Economic Justification
- Dow Theory
- VALUATION PHILOSOPHIES
- Ratio Analysis
- INVESTMENT RATIOS
- Bottom-Up, Top-Down Approach to Fundamental Analysis
- The Industry Life Cycle
- COMPANY ANALYSIS
- Analyzing a Company’s Profitability
- Objective of Financial Statements
- RESEARCH PHILOSPHY
- What Is An Investment Company
- Exchange-Traded Funds (ETFs)
- COMMON STOCK: ANALYSIS AND STRATEGY
- THE EFFICIENT MARKET HYPOTHESIS (EMH)
- Behavioral Finance
- MARKET INDEXES
- POPULAR INDEXES
- BOND PRINCIPLES
- BOND PRICING AND RETURNS
- Accrued Interest
- BOND RISKS
- UNDERSTANDING RISK AND RETURN
- TYPES & SOURCES OF RISK
- Measuring Risk
- ANALYZING PORTFOLIO RISK
- Building a Portfolio Using Markowitz Principles
- Capital Market Theory: Assumptions, The Separation Theorem
- Risk-Free Asset, Estimating the SML
- Formulate an Appropriate Investment Policy
- EVALUATION OF INVESTMENT PERFORMANCE
- THE ROLE OF DERIVATIVE ASSETS
- THE FUTURES MARKET
- Using Futures Contracts: Hedgers
- Financial Futures: Short Hedges, Long Hedges
- Risk Management, Risk Transfer, Financial Leverage
- OVERVIEW