 |

Corporate
Finance FIN 622
VU
Lesson
15
RISK
AND RETURNS
The
following topics will be
discussed in this lecture.
Risk
and Uncertainty
Measuring
risk
Variability
of return Historical Return
Variance
of return
Standard
Deviation
RISK
AND UNCERTAINTY:
If you
buy an asset or any stock or
share, the gains or losses
you get on this investment are
called return on
investment. This
return has normally two
components. First, it is the income part
that you may receive
in
terms
of dividend (owning a share)
and second part comes
from the capital appreciation or increase
in the
market
value of that share.
The
above discussion suggests
that the reward of return
you get is the due to
bearing the risk. Risk
refers to
the
variability of returns. You
may get dividend on a share
say 2% or 15%, or even
you may not
get
anything
from the issuing firm. Look
at this simple example: the expected
returns (income part only)
can
vary
from 0 to 15%. This is
called risk. However, you can
use probabilities to determine
your return. For
instance,
if the economy remains in boom,
which has 60% chances, then
our return will be 8%. So
attaching
probability
we can to some extent, determine the
return under risk conditions.
The
other important thing to
remember is that greater the risk,
larger the profit.
Uncertainty,
refers to a situation where
our ability to attach a
probability to an outcome is
ceased.
From
hereafter, we shall discuss the
ways and means to measure
the risk.
HISTORICAL
RETURN ANALYSIS:
The
problem with most financial
planning is they accept a return
rate on each of your
investments and
project
your financial future on those
rates. The argument is over
a span of years your
investments will
return
that rate "on average."
Unfortunately this is an invalid and
risky assumption. Investment rates
vary
from
year to year. Sometimes they vary
greatly. We cannot accurately predict the
return rate on
investments
or the
inflation rate. Consider the
following simple
example
You
have $1000.00 invested and
you expect a 10.0% average
yearly return on your investment. In
two years
your
investment will be worth
$1210.00.
Now
lets assume your same
$1000.00 returns -10.00% the
first year and +30.00% the
second. Your
investments
after those two years are
worth only $1170.00 even
though your investment returned
"on
average"
10.0%.
The
above example demonstrates the
need for a mechanism to
account for the volatility of
investment
return
rates and the variability of
inflation. The J&L Financial Planner
has chosen to include
two
alternatives,
a Monte Carlo Analysis and a
Historical Return Analysis, as that
mechanism.
J&L
Financial Planner's Historical
Return Analysis
The
following paragraphs outline
how the Historical Return Analysis is
implemented by the J&L Financial
Planner.
The
J&L Financial Planner allows you to
create simple or complex financial
scenarios (financial plans)
revolving
around your existing accounts consisting
of investment, retirement, asset, and equity
accounts.
The
planner allows you to create
and assign up to 10 asset allocation
classes for each of your
accounts. A
simple
example would have you
create three asset allocation
classes Stocks, Bonds, and
Cash. You would
assign
each account the percentage of
each of its allocation classes. A mutual
fund account may consist
of
70
percent Stocks and 30
percent Bonds, whereas a
savings account would be 100
percent Cash. For
each
allocation
class you assign a historical
return data file
representing the returns for
that class over an
historical time
span. The planner comes with
6 example data files including 2
stock files, 2 bond files, 1
cash
file,
and an inflation file covering the
years 1928 through 2003.
The files are provided as
examples and
should be
replaced with data files
which meet your needs.
You can create and
edit up to 10 files,
each
corresponding to an
asset allocation class.
The
planner gives you two
options with the Historical Return
Analysis.
The
first allows you to execute
your financial plan over the historical
time span. This generates your
net
worth
for each year of your plan
based on the returns of the historical
data starting with the first
year of the
47

Corporate
Finance FIN 622
VU
data.
In the provided files this would generate
a net worth (a line graph) starting
with the returns from
1928.
Next
it would generate a net
worth starting with the returns
from 1929. It would do this
for each year of
your
financial plan.
The
second allows you to randomly
select return data from the
historical data files and use
that data to
calculate
your net worth over the span
of your financial plan. It also gives
you the option of selecting
the
number of
sequential years the program will
use. In other words, if you
select 10 years of sequential
data,
the program
randomly selects the first year
and then uses the data
from the files for the following 9
years
before randomly
selecting another year. For
example if you choose the number of
sequential years as 1
and
select
1000 trials it will randomly select
return data from the historical
data files for each year of
your plan
and
execute your plan 1000
times. This has the effect of a
Monte Carlo analysis with the random
data being
randomly
selected from real historical
return data.
Summary
In
summary, the Historical Return Analysis
is able to estimate the probability of
achieving the success of
your
scenario by accounting for the
yearly variability in the two
main factors contributing to
its outcome,
the
return rate on your
investments and the inflation
rate. You can execute up to
a thousand trials of your
scenario.
Each trial is a fully independent
execution of your financial plan, where
each year the return
rate
on
your investments and the
inflation rate can take on a
range of values based on historical
asset class return
data.
The
large number of trials allows the
analysis to compute the statistical
probability your financial plan
will
be
successful. For example, if after
1000 trials, 750 of those
trials achieved your financial goals,
your
financial plan
success rate is
75.0%.
If
your financial plan success rate is
below your expectations the J&L
Financial Planner allows you to
make
easy
scenario changes to play "what-if"
with your financial
future.
VARIANCE
OF RETURN:
The
variance essentially measures the
average squared difference between the
actual returns and the
average
return.
The bigger this number is, the
more the actual returns tend
to differ from the average
return. Also,
the
larger the variance is the ore
spread out the returns will
be.
It is
pertinent to note here that
calculating variance and
standard deviation will be
different for historical
and
projected returns.
This
is usually very close to the correlation
squared. To understand what variance
explained means, think of
a
manager and a Style
Benchmark. Any variance in the difference
between manager and Style
Benchmark,
i.e.,
any variance in the excess
return of manager over
benchmark, represents a failure of the
Style
Benchmark
variance to explain the manager variance.
Hence, the quotient of variance of
excess return over
variance
of manager represents the unexplained
variance. The variance explained is 1
minus the unexplained
variance:
Variance
Explained = 1 - Var (e) /
Var (M)
Where:
Var
(M) = variance of manager
returns
Var
(e) = variance of excess
return of manager over
benchmark
STANDARD
DEVIATION:
Were
this set a sample drawn from a
larger population of children, and the
question at hand was the
standard
deviation of the population, convention
would replace the N (or 4)
here with N-1 (or
3).
The
standard deviation of a probability
distribution is defined as the square
root of the variance ,
(1)
(2)
Where
is the
mean,
is the
second raw moment, and
denotes an expectation
value.
is therefore
equal to the second central moment
(i.e., moment about the mean),
The
variance
(3)
48
Corporate
Finance FIN 622
VU
The
square root of the sample
variance of a set of
values
is the sample standard
deviation
(4)
The
sample standard deviation
distribution is a slightly complicated,
though well-studied and
well-
understood,
function.
However,
consistent with widespread
inconsistent and ambiguous
terminology, the square root of the
bias-
corrected
variance is sometimes also
known as the standard
deviation,
(5)
Physical
scientists often use the term root-mean
square as a synonym for
standard deviation when they refer
to the
square root of the mean
squared deviation of a quantity
from a given baseline.
The
standard deviation arises naturally in
mathematical statistics through
its definition in terms of
the
second
central moment. However, a more natural
but much less frequently
encountered measure of
average
deviation
from the mean that is used
in descriptive statistics is the
so-called mean
deviation.
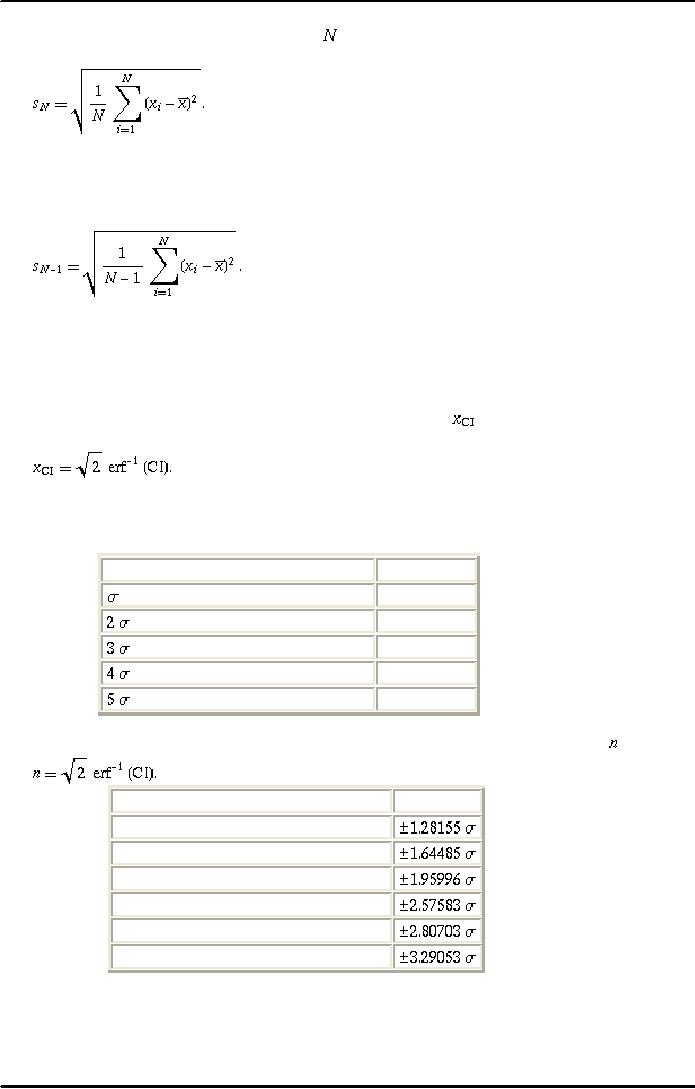
The
variants value producing a confidence
interval CI is often denoted ,
and
(6)
The
following table lists the confidence
intervals corresponding to the first few multiples of the
standard
deviation.
Range
CI
0.6826895
0.9544997
0.9973002
0.9999366
0.9999994
To
find the standard deviation
range corresponding to a given confidence interval,
solve (5) for ,
giving
(7)
CI
range
0.800
0.900
0.950
0.990
0.995
0.999
49
Table of Contents:
- INTRODUCTION TO SUBJECT
- COMPARISON OF FINANCIAL STATEMENTS
- TIME VALUE OF MONEY
- Discounted Cash Flow, Effective Annual Interest Bond Valuation - introduction
- Features of Bond, Coupon Interest, Face value, Coupon rate, Duration or maturity date
- TERM STRUCTURE OF INTEREST RATES
- COMMON STOCK VALUATION
- Capital Budgeting Definition and Process
- METHODS OF PROJECT EVALUATIONS, Net present value, Weighted Average Cost of Capital
- METHODS OF PROJECT EVALUATIONS 2
- METHODS OF PROJECT EVALUATIONS 3
- ADVANCE EVALUATION METHODS: Sensitivity analysis, Profitability analysis, Break even accounting, Break even - economic
- Economic Break Even, Operating Leverage, Capital Rationing, Hard & Soft Rationing, Single & Multi Period Rationing
- Single period, Multi-period capital rationing, Linear programming
- Risk and Uncertainty, Measuring risk, Variability of return–Historical Return, Variance of return, Standard Deviation
- Portfolio and Diversification, Portfolio and Variance, Risk–Systematic & Unsystematic, Beta – Measure of systematic risk, Aggressive & defensive stocks
- Security Market Line, Capital Asset Pricing Model – CAPM Calculating Over, Under valued stocks
- Cost of Capital & Capital Structure, Components of Capital, Cost of Equity, Estimating g or growth rate, Dividend growth model, Cost of Debt, Bonds, Cost of Preferred Stocks
- Venture Capital, Cost of Debt & Bond, Weighted average cost of debt, Tax and cost of debt, Cost of Loans & Leases, Overall cost of capital – WACC, WACC & Capital Budgeting
- When to use WACC, Pure Play, Capital Structure and Financial Leverage
- Home made leverage, Modigliani & Miller Model, How WACC remains constant, Business & Financial Risk, M & M model with taxes
- Problems associated with high gearing, Bankruptcy costs, Optimal capital structure, Dividend policy
- Dividend and value of firm, Dividend relevance, Residual dividend policy, Financial planning process and control
- Budgeting process, Purpose, functions of budgets, Cash budgets–Preparation & interpretation
- Cash flow statement Direct method Indirect method, Working capital management, Cash and operating cycle
- Working capital management, Risk, Profitability and Liquidity - Working capital policies, Conservative, Aggressive, Moderate
- Classification of working capital, Current Assets Financing – Hedging approach, Short term Vs long term financing
- Overtrading – Indications & remedies, Cash management, Motives for Cash holding, Cash flow problems and remedies, Investing surplus cash
- Miller-Orr Model of cash management, Inventory management, Inventory costs, Economic order quantity, Reorder level, Discounts and EOQ
- Inventory cost – Stock out cost, Economic Order Point, Just in time (JIT), Debtors Management, Credit Control Policy
- Cash discounts, Cost of discount, Shortening average collection period, Credit instrument, Analyzing credit policy, Revenue effect, Cost effect, Cost of debt o Probability of default
- Effects of discounts–Not effecting volume, Extension of credit, Factoring, Management of creditors, Mergers & Acquisitions
- Synergies, Types of mergers, Why mergers fail, Merger process, Acquisition consideration
- Acquisition Consideration, Valuation of shares
- Assets Based Share Valuations, Hybrid Valuation methods, Procedure for public, private takeover
- Corporate Restructuring, Divestment, Purpose of divestment, Buyouts, Types of buyouts, Financial distress
- Sources of financial distress, Effects of financial distress, Reorganization
- Currency Risks, Transaction exposure, Translation exposure, Economic exposure
- Future payment situation – hedging, Currency futures – features, CF – future payment in FCY
- CF–future receipt in FCY, Forward contract vs. currency futures, Interest rate risk, Hedging against interest rate, Forward rate agreements, Decision rule
- Interest rate future, Prices in futures, Hedging–short term interest rate (STIR), Scenario–Borrowing in ST and risk of rising interest, Scenario–deposit and risk of lowering interest rates on deposits, Options and Swaps, Features of opti
- FOREIGN EXCHANGE MARKET’S OPTIONS
- Calculating financial benefit–Interest rate Option, Interest rate caps and floor, Swaps, Interest rate swaps, Currency swaps
- Exchange rate determination, Purchasing power parity theory, PPP model, International fisher effect, Exchange rate system, Fixed, Floating
- FOREIGN INVESTMENT: Motives, International operations, Export, Branch, Subsidiary, Joint venture, Licensing agreements, Political risk